标签:
(注:此贴是为了回答同事提出的一个问题而匆匆写就,算法代码只求得出答案为目的,效率方面还有很大的改进空间)
最小生成树是指对于给定的带权无向图,需要生成一个总权重最小的连通图。
其问题描述及算法可以详见:https://en.wikipedia.org/wiki/Minimum_spanning_tree
以下我选用其中一个简单的算法描述,编写 Python 代码尝试解决此问题。
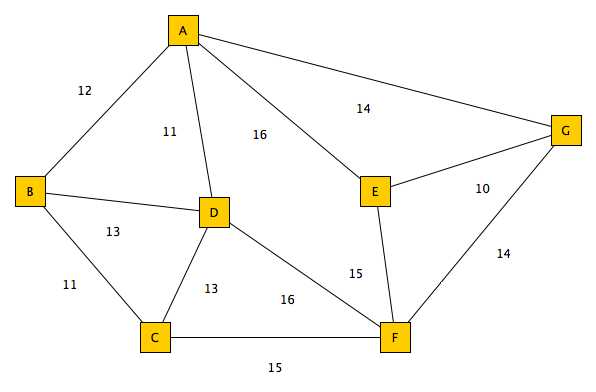
下面是同事提出的问题的原图:
程序:
1 # coding: utf-8 2 3 from sets import Set 4 5 def edge_name(v1, v2): 6 if v1 < v2: 7 return v1 + v2 8 return v2 + v1 9 10 11 # Prim algorithm 12 def mst(vectors, costs, vectors_in_g, edges_in_g): 13 if len(vectors) == len(vectors_in_g): 14 return (vectors_in_g, edges_in_g) 15 16 min_cost = max(costs.values()) + 1 17 remaining_vectors = vectors.difference(vectors_in_g) 18 next_vector = ‘‘ 19 next_edge = ‘‘ 20 21 for x in vectors_in_g: 22 for y in remaining_vectors: 23 ename = edge_name(x, y) 24 if ename not in costs: 25 continue 26 cost = costs[ename] 27 if cost < min_cost: 28 next_vector = y 29 next_edge = ename 30 min_cost = cost 31 32 if next_vector <> ‘‘ and next_edge <> ‘‘: 33 new_vectors_in_g = vectors_in_g.copy() 34 new_edges_in_g = edges_in_g[:] 35 new_vectors_in_g.add(next_vector) 36 new_edges_in_g.append(next_edge) 37 38 return mst(vectors, costs, new_vectors_in_g, new_edges_in_g) 39 else: 40 raise Exception("The MST can not be found.") 41 42 my_vectors = Set([‘a‘, ‘b‘, ‘c‘, ‘d‘, ‘e‘, ‘f‘, ‘g‘]) 43 my_costs = { 44 ‘ab‘: 12, 45 ‘ad‘: 11, 46 ‘ae‘: 16, 47 ‘ag‘: 14, 48 ‘bc‘: 11, 49 ‘bd‘: 13, 50 ‘cd‘: 13, 51 ‘cf‘: 15, 52 ‘df‘: 16, 53 ‘ef‘: 15, 54 ‘eg‘: 10, 55 ‘fg‘: 14 56 } 57 58 vs, es = mst(my_vectors, my_costs, Set(list(my_vectors)[0]), []) 59 60 print vs 61 print es 62 63 total_cost = 0 64 for e in es: 65 total_cost += my_costs[e] 66 67 print total_cost
程序输出如下:
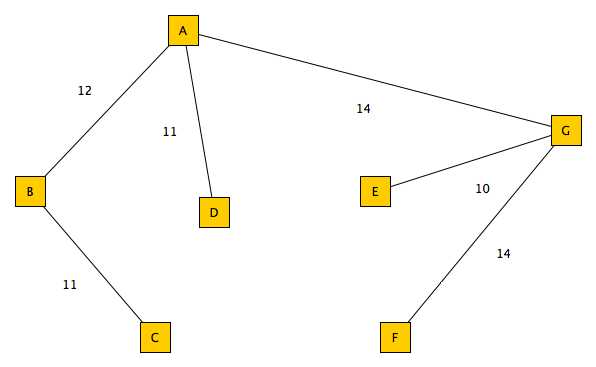
Set([‘a‘, ‘c‘, ‘b‘, ‘e‘, ‘d‘, ‘g‘, ‘f‘]) [‘ad‘, ‘ab‘, ‘bc‘, ‘ag‘, ‘eg‘, ‘fg‘] 72
从输出结果可知最优解的总权重72. 图示如下:
算法练习:最小生成树 (Minimum Spanning Tree)
标签:
原文地址:http://www.cnblogs.com/thomas888/p/mst.html