十大算法系列之(一)快速排序

标签：

&1 思想和时间复杂度

• 分治法思想(Divide and Conquer)，这是算法导论里面讲的第一个算法思想，很经典也很实用。
• 时间复杂度分析，最好是O(n)，最差是O(n²)，平均性能是O(nlog(n))。

&2 算法

　　#1. 在待排数列中(n个数)选择一个基准数(理论上可以任意)；

　　#2. 剩下的 n-1 个数与基准数对比，比基准数小的放左边，反之在右边；(也有人称为划分操作)

　　#3. 一轮循环结束，此时基准数是位于它的最终位置；

　　#4. 重复步骤#1~#3，直到最后一个基准数左边和右边的元素个数为0或1，则排序完成。

&3 举例

&4 c++程序实现

#include<iostream>
#include<vector>

using namespace std;

void QuickSort(vector<int > &A, int p, int q);
int Partition(vector<int> &a, int p, int q);
void swapv(int &a, int &b);

int main(){
　　vector<int> seti = { 13, 19, 9, 5, 12, 8, 7, 4, 21, 2, 6, 11 };
　　int len = seti.size();
　　QuickSort(seti,0,len-1);

　　cout << "The sorted array is: \n";
　　for (vector<int>::iterator iter = seti.begin(); iter != seti.end(); iter++)
　　cout << *iter << "\t";
　　cout << endl;

　　system("Pause");
　　return 0;
}

void QuickSort(vector<int > &A,int p,int q){
　　if (p < q){
　　　　int pos = Partition(A, p, q);
　　　　QuickSort(A, p, pos - 1);
　　　　QuickSort(A, pos + 1, q);
　　}
}

int Partition(vector<int> &a,int p, int q){
　　int val = a[p];
　　int j = q + 1;
　　for (int i = q; i > p-1; i--){
　　　　if (a[i]>val){
　　　　　　j = j - 1;
　　　　　　if (i != j)
　　　　　　　　swapv(a[j],a[i]);
　　　　}
　　}

　　if (p != j - 1)
　　　　swapv(a[p],a[j-1]);

　　return j - 1;
}

void swapv(int &a,int &b){
　　a = a + b;
　　b = a - b;
　　a = a - b;
}

&5 程序实现结果截图

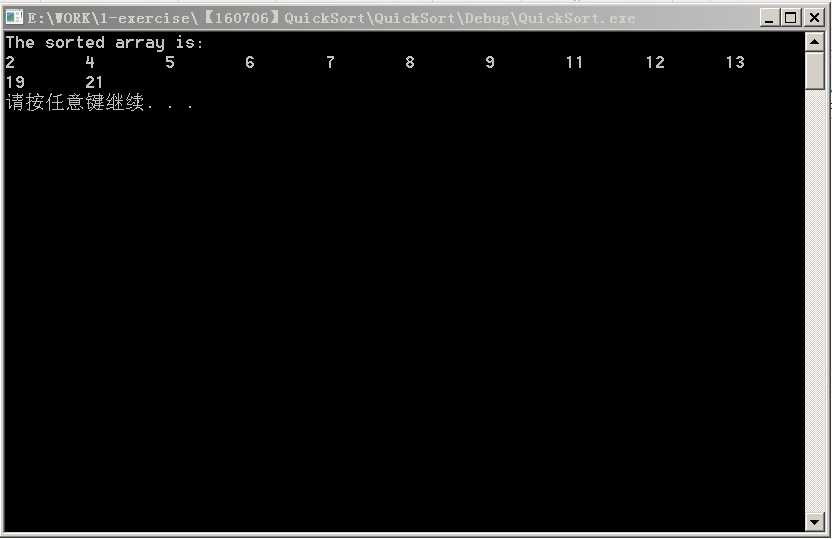
如果对代码优化，亲有更好的想法，咱可以一起学习讨论哦！

十大算法系列之(一)快速排序

标签：

原文地址：http://www.cnblogs.com/sophia-hxw/p/5357824.html