标签:解释 color pytho ... while blog title 公式 python
平方,开根号在java中是很简单的,Math.sqrt(double n)或者 Math.pow(double a, double b),求a的b次方。但是我们可以自己想想,这些方法到底是怎么实现的。
就拿开根号来解释,它有两种方法,二分法和牛顿迭代法。
二分法:
比如求根号5
第一步:折半: 5/2=2.5
第二步:平方校验: 2.5*2.5=6.25>5,并且得到当前上限2.5,记录。
第三步:再次向下折半:2.5/2=1.25
第四步:平方校验:1.25*1.25=1.5625<5,得到当前下限1.25,记录
第五步:再次折半:2.5-(2.5-1.25)/2=1.875
第六步:平方校验:1.875*1.875=3.515625<5,得到当前下限1.875,替换下限值
......
一直到与5的差值在你定义的误差范围内才结束循环
代码:
import java.text.DecimalFormat; public class Main { public static double sqrt(double num){ if(num<0) { return -1; } double low = 0; double high = num/2; double precision = 0.000001; //格式化,保证输出位数 DecimalFormat df = new DecimalFormat("#.00"); double res = high; while(Math.abs(num-(res*res))>precision) { if(high*high > num) { double n= high - (high-low)/2; if(n*n>num) { high = n; } else if(n*n<num){ low = n; }else { return Double.valueOf(df.format(n)); } res = n; } else if(high*high < num){ double m = high + (high-low)/2; if(m*m>num) { low = high; high = m; } else if(m*m<num){ low = high; high = m; }else { return Double.valueOf(df.format(m)); } res = m; } else { return Double.valueOf(df.format(high)); } } return Double.valueOf(df.format(res)); } public static void main(String[] args) { double a = 7; System.out.println(sqrt(37)); } }
牛顿迭代法:
其实就是逼近的思想,例如我们要求a的平方根,首先令f(x)=x^2-a,那么我们的目的就是求得x使得f(x)=0,也就是求x^2-a这条曲线与x轴的交点,画图举例:
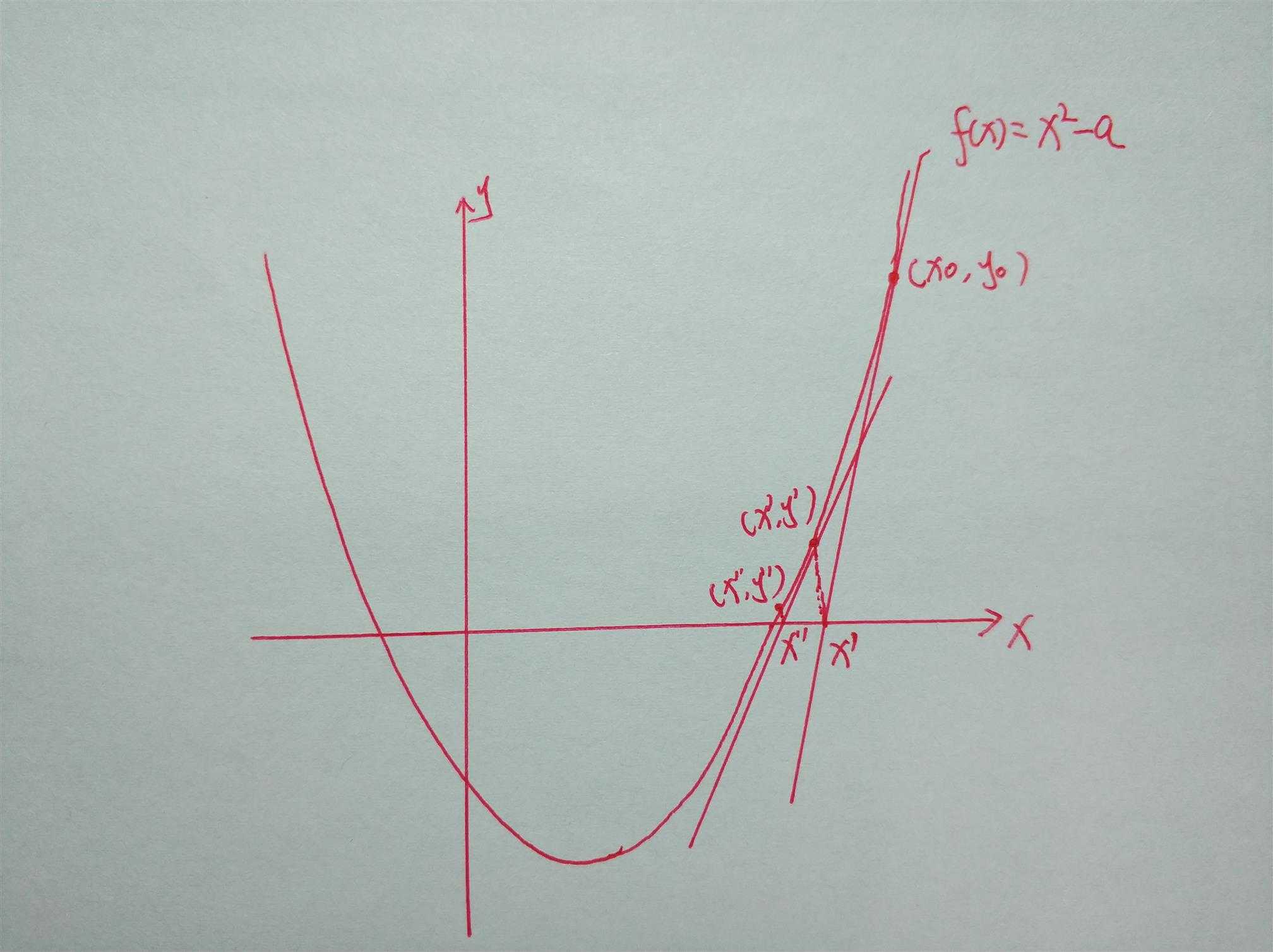
由函数f(x)=x^2-a,我们求导可以知道,函数上任意一点(x,y)的切线的斜率为2x。假设过点(x0,y0)的切线方程为y=kx+b,那么切线与x轴的交点横坐标为-b/k。而b=y0-kx0,k=2x0,y0=x0^2-a,化简-b/k=(x0+a/x0)/2。
也就是说(x0+a/x0)/2是过点(x0,y0)的切线与x轴的交点的横坐标。记(x0+a/x0)/2=x‘,继续求过点(x‘,f(x‘))的切线与x轴的交点的横坐标x‘‘,很明显x‘‘比x‘更靠近函数f(x)=x^2-a与x轴的交点的横坐标(即a的正平方根)。逐渐的逼近f(x)=0;
所以公式为:x‘ = (x‘+a/x‘)/2。
代码:
import java.text.DecimalFormat; public class Main1 { public static double sqrt(double x) { if(x<0) { return -1; } //格式化,保证输出位数 DecimalFormat df = new DecimalFormat("#.00"); double k = x; double precision = 0.000001; while((k*k-x)>precision) { k=0.5*(k+x/k); } return Double.valueOf(df.format(k)); } public static void main(String[] args) { double a = 9; System.out.println(sqrt(a)); } }
参考文献:
标签:解释 color pytho ... while blog title 公式 python
原文地址:http://www.cnblogs.com/loren-Yang/p/7532476.html