Minimum Window Substring

标签：minimum window subst   leetcode   

Given a string S and a string T, find the minimum window in S which will contain all the characters in T in complexity O(n).

For example,
S = "ADOBECODEBANC"
T = "ABC"

Minimum window is "BANC".

Note:
If there is no such window in S that covers all characters in T, return the emtpy string "".

If there are multiple such windows, you are guaranteed that there will always be only one unique minimum window in S.

我们下面使用 S = "acbbaca" and T = "aba" 来演示这个算法。基本思路是在遍历S的过程中，使用两个指针（合法window的begin和end索引）和两个table（needToFind和hasFound），needToFind保存T中每个字母的个数（二娃：相当于我们的needToFill），hasFound保存当前收集到的每个字母的个数。我们也同时使用一个count变量，用来保存当前收集到的字母总数，但是那些收集过了的字母数并不算在count里面。这样的话，当count等于T.length，那我们就知道遇到一个合法的window了。

我们利用end指针来遍历S，假设当前end指向S中的字母x，如果x是T中的字母，hasFound[x]加一。如果hasFound[x]目前还小于等于needToFind[x]（二娃：说明字母x还没有收集全或者刚刚收集全哦），那么我们同时也增加count。当合法window的条件满足，也就是count等于T.length，我们立即递增begin指针，并且保证在递增的过程中始终有count等于T.length。

在递增begin指针的过程中，我们怎么样才能保证“始终有count等于T.length”呢？

假设begin指向字母x，如果hasFound[x]大于了needToFind[x]，hasFound[x]减去一，同时递增begin。（二娃：这里很有画面感哦。因为当前遇到的x是冗余的靠左的字母，这里的操作其实等价于前面两个算法中的“删除charAppearanceRecorder中相应的字母的链表头节点”，有点儿像一个是lazy去重，一个是eager去重）否则的话，当前的begin就是window的起始索引了。

接下来我们就可以通过end - begin + 1得到当前window的长度了。这里便可以更新最小window长度。

算法实际上首先找到第一个合法的window，然后在接下来的扫描过程中保持window的合法性（二娃：其实就是count 始终小于等于（当遇到新window）T.length）。

看下面的图图。

i）S = "acbbaca" and T = "aba".

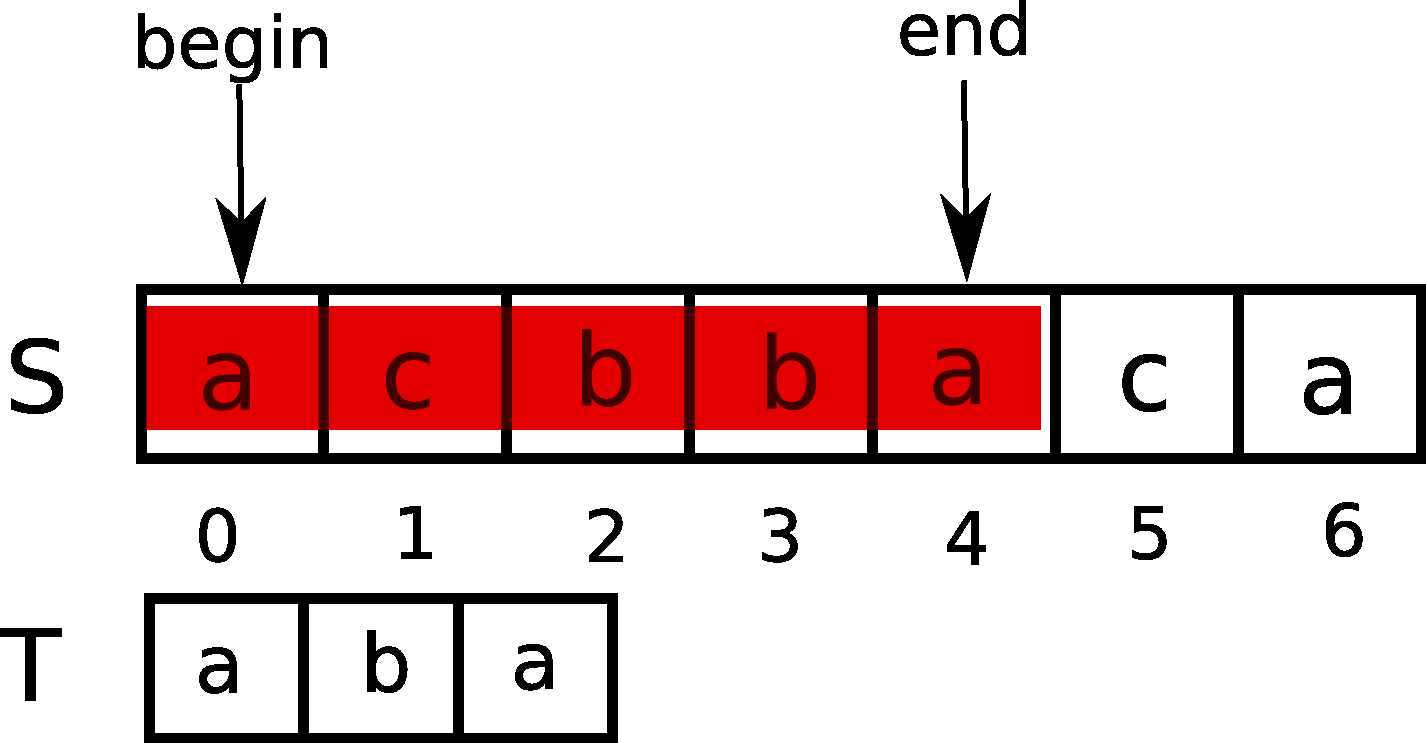
ii）找到第一个合法的window。这里注意我们不能递增begin指针因为hasFound[‘a‘] 等于 needToFind[‘a‘]，即2. 如果我们此时递增begin，那就不是合法window了。

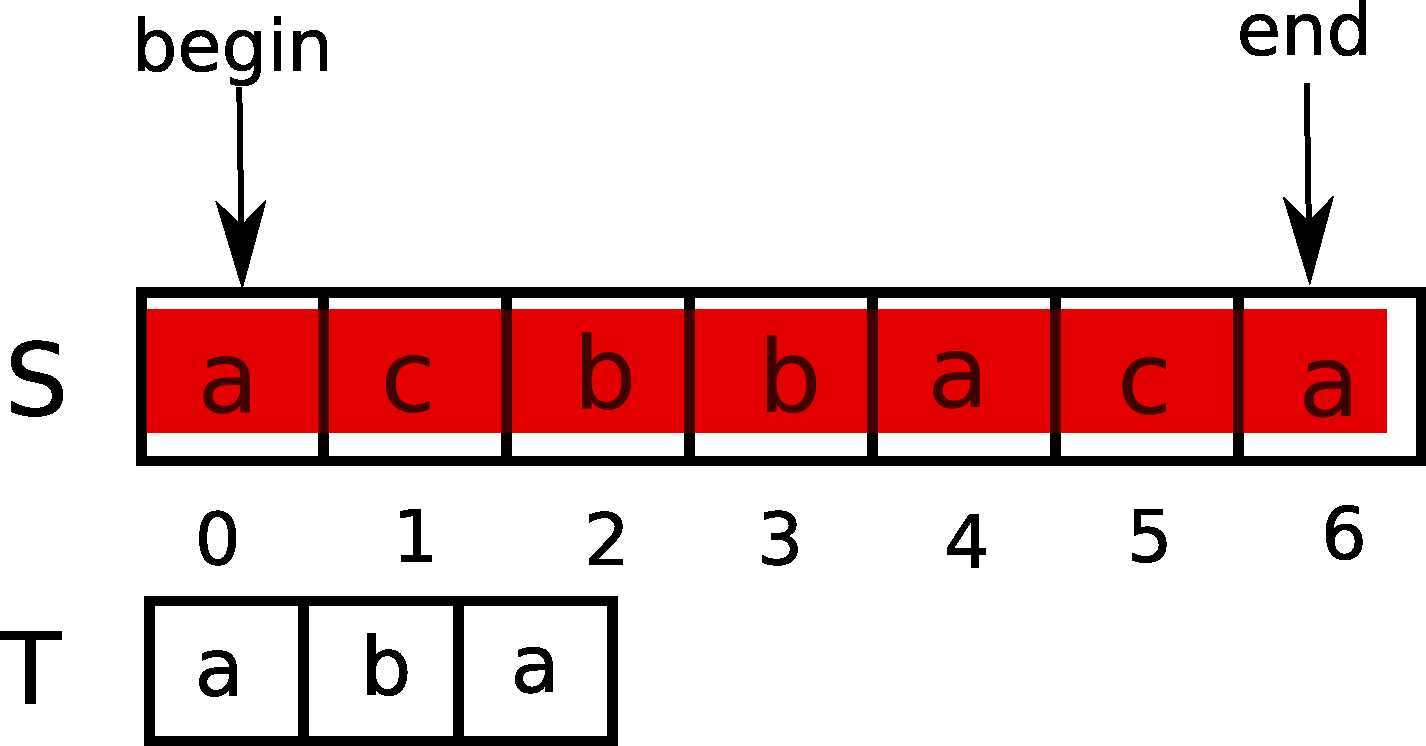
iii）找到第二个合法的window。begin指针指向第一个a，hasFound[‘a‘]等于3，而needToFind[‘a‘]等于2，说明这个a是一个冗余的a，我们递减hasFound[‘a‘]同时递增begin。

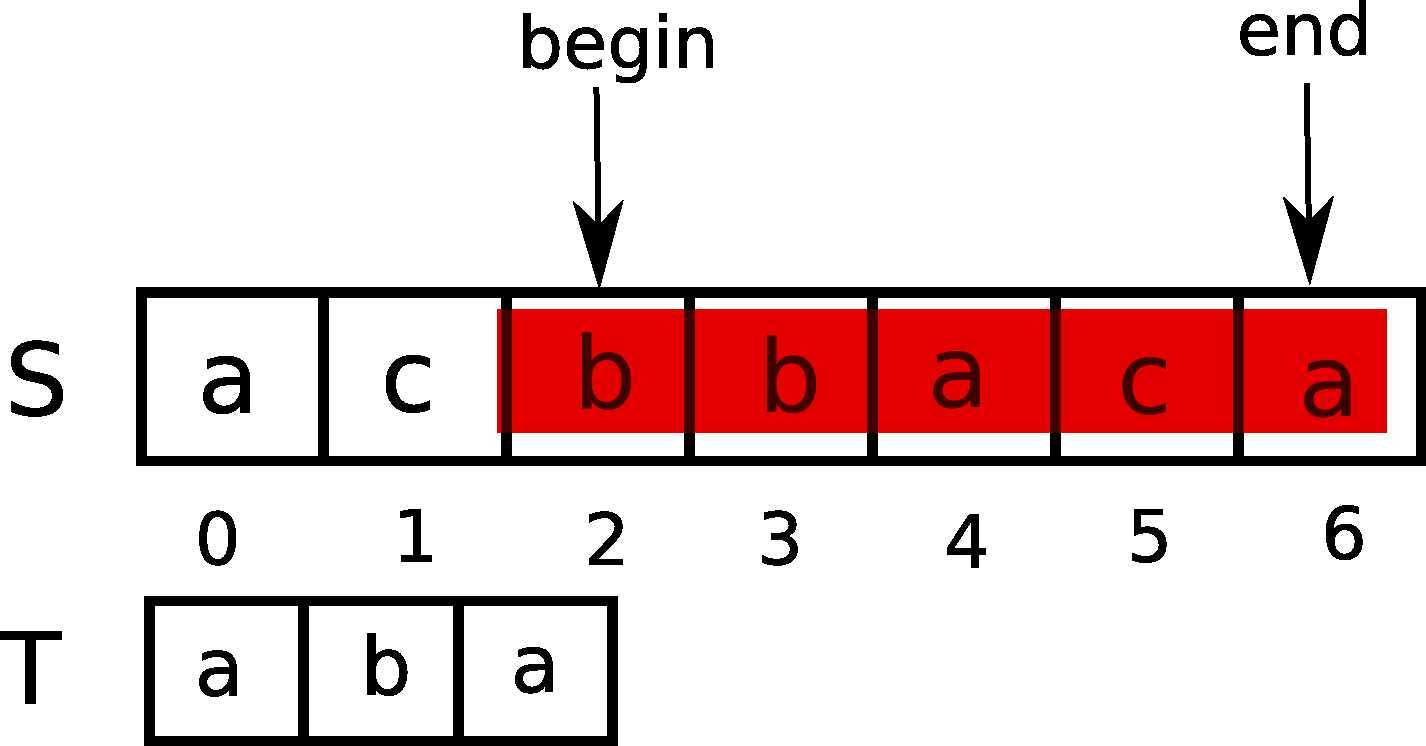
iv）我们也需要跳过那些不在T中的字母，例如上面的c。现在beging指向了b，hasFound[‘b‘]等于2，大于了needToFind[‘b‘]，说明这也是一个冗余的b，我们递减hasFound[‘a‘]同时递增begin。

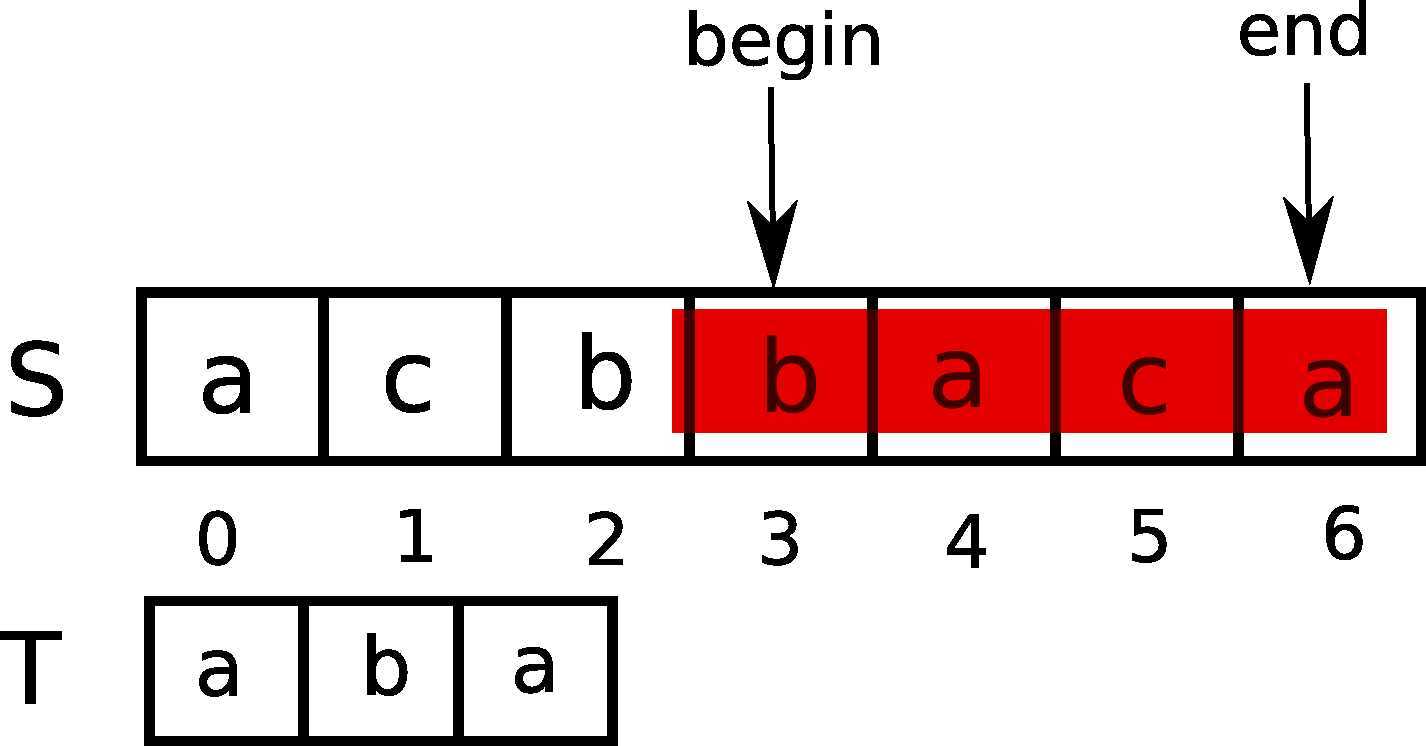
v）begin指向b，这时候hasFound[‘b‘]等于needToFind[‘b‘]。不能再减了，同时begin也不能再次移动了，这里就是一个短window的起点位置。

begin和end都最多前进N次，从而整个算法执行小于2N. 复杂度是O(N)。

class Solution {
private:
    int count1[256];
    int count2[256];
public:
    string minWindow(string S, string T) {
        // Start typing your C/C++ solution below
        // DO NOT write int main() function
        if (T.size() == 0 || S.size() == 0)
            return "";
            
        memset(count1, 0, sizeof(count1));
        memset(count2, 0, sizeof(count2));
        
        for(int i = 0; i < T.size(); i++)
        {
            count1[T[i]]++;
            count2[T[i]]++;
        }
        
        int count = T.size();
        
        int start = 0;
        int minSize = INT_MAX;
        int minStart;
        for(int end = 0; end < S.size(); end++)
        {
            if (count2[S[end]] > 0)
            {
                count1[S[end]]--;
                if (count1[S[end]] >= 0)
                    count--;
            }
            
            if (count == 0)
            {
                while(true)
                {
                    if (count2[S[start]] > 0)
                    {
                        if (count1[S[start]] < 0)
                            count1[S[start]]++;
                        else
                            break;
                    }
                    start++;
                }
            
                if (minSize > end - start + 1)
                {
                    minSize = end - start + 1;
                    minStart = start;
                }
            }
        }
        
        if (minSize == INT_MAX)
            return "";
        
        string ret(S, minStart, minSize);
        
        return ret;        
    }
};

Minimum Window Substring

标签：minimum window subst   leetcode   

原文地址：http://blog.csdn.net/huruzun/article/details/39831039