标签:tle inf int .com pen 结果 end 棋盘覆盖 pre
编写象棋界面
import turtle t=turtle.Pen() t.speed(100) def angle(x,y): t.penup() t.goto(x+3,y+3) t.pendown() t.setheading(0) t.forward(5) t.goto(x+3,y+3) t.left(90) t.forward(5) t.penup() t.goto(x+3,y-3) t.pendown() t.setheading(0) t.forward(5) t.goto(x+3,y-3) t.left(90) t.forward(-5) t.penup() t.goto(x-3,y+3) t.pendown() t.setheading(0) t.forward(-5) t.goto(x-3,y+3) t.left(90) t.forward(5) t.penup() t.goto(x-3,y-3) t.pendown() t.setheading(0) t.forward(-5) t.goto(x-3,y-3) t.left(90) t.forward(-5) def v(x,y): t.penup() t.goto(x+3,y+3) t.pendown() t.setheading(0) t.forward(5) t.goto(x+3,y+3) t.left(90) t.forward(5) t.penup() t.goto(x+3,y-3) t.pendown() t.setheading(0) t.forward(5) t.goto(x+3,y-3) t.left(90) t.forward(-5) t.penup() def a(x,y): t.penup() t.goto(x-3,y+3) t.pendown() t.setheading(0) t.forward(-5) t.goto(x-3,y+3) t.left(90) t.forward(5) t.penup() t.goto(x-3,y-3) t.pendown() t.setheading(0) t.forward(-5) t.goto(x-3,y-3) t.left(90) t.forward(-5) #1.绘制所有横线 t.penup() t.goto(-80,90) t.pendown() for i in range(1,6,1): t.forward(160) t.penup() t.right(90) t.forward(20) t.right(90) t.pendown() t.forward(160) t.penup() t.left(90) t.forward(20) t.left(90) t.pendown() #2.绘制所有竖线 t.left(90) t.penup() t.forward(20) t.pendown() for i in range(1,5,1): t.forward(80) t.penup() t.forward(20) t.pendown() t.forward(80) t.right(90) t.forward(20) t.right(90) t.forward(80) t.penup() t.forward(20) t.pendown() t.forward(80) t.left(90) t.forward(20) t.left(90) t.forward(180) t.left(90) t.forward(160) t.left(90) t.forward(180) #3.绘制斜线 t.left(90) t.forward(60) t.left(45) t.forward(40*1.414) t.left(45) t.forward(-40) t.left(45) t.forward(40*1.414) t.penup() t.goto(-20,90) t.pendown() t.right(180) t.forward(40*1.414) t.right(45) t.forward(-40) t.right(45) t.forward(40*1.414) #4.绘制炮和兵的位置 angle(60,50) angle(-60,50) angle(60,-50) angle(-60,-50) angle(40,30) angle(-40,30) angle(40,-30) angle(-40,-30) angle(0,30) angle(0,-30) a(80,30) a(80,-30) v(-80,-30) v(-80,30) #5.绘制外围线 绘制一个长方形,设置笔的粗细 t.penup() t.goto(-90,-100) t.pendown() t.pensize(10) t.forward(200) t.right(90) t.forward(180) t.right(90) t.forward(200) t.right(90) t.forward(180) t.right(90)
棋盘覆盖问题
在2^k*2^k个方格组成的棋盘中,有一个方格被占用,用下图的4种L型骨牌覆盖所有棋盘上的其余所有方格,不能重叠。
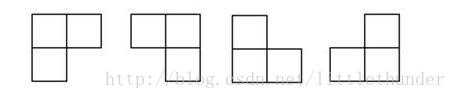
代码如下:
def chess(tr,tc,pr,pc,size): global mark global table mark+=1 count=mark if size==1: return half=size//2 if pr<tr+half and pc<tc+half: chess(tr,tc,pr,pc,half) else: table[tr+half-1][tc+half-1]=count chess(tr,tc,tr+half-1,tc+half-1,half) if pr<tr+half and pc>=tc+half: chess(tr,tc+half,pr,pc,half) else: table[tr+half-1][tc+half]=count chess(tr,tc+half,tr+half-1,tc+half,half) if pr>=tr+half and pc<tc+half: chess(tr+half,tc,pr,pc,half) else: table[tr+half][tc+half-1]=count chess(tr+half,tc,tr+half,tc+half-1,half) if pr>=tr+half and pc>=tc+half: chess(tr+half,tc+half,pr,pc,half) else: table[tr+half][tc+half]=count chess(tr+half,tc+half,tr+half,tc+half,half) def show(table): n=len(table) for i in range(n): for j in range(n): print(table[i][j],end=‘ ‘) print(‘‘) mark=0 n=8 table=[[-1 for x in range(n)] for y in range(n)] chess(0,0,2,2,n) show(table)
n是棋盘宽度,必须是2^k,本例中n=8,特殊格子在(2,2)位置,如下图所示:
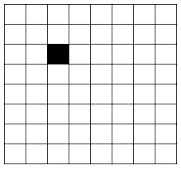
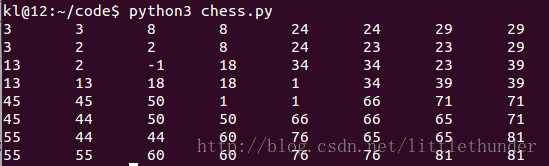
采用分治法每次把棋盘分成4份,如果特殊格子在这个小棋盘中则继续分成4份,如果不在这个小棋盘中就把该小棋盘中靠近中央的那个格子置位,表示L型骨牌的1/3占据此处,每一次递归都会遍历查询4个小棋盘,三个不含有特殊格子的棋盘置位的3个格子正好在大棋盘中央构成一个完整的L型骨牌,依次类推,找到全部覆盖方法。运行结果如下:
标签:tle inf int .com pen 结果 end 棋盘覆盖 pre
原文地址:https://www.cnblogs.com/daofaziran/p/10083120.html