标签:列表 sea 实现 arc queue alt 国际 图片 朋友
广度优先算法是为了解决两样东西之间的最短距离,其中最短距离的含义很多,如:
图由节点和边组成,模拟一组链接。
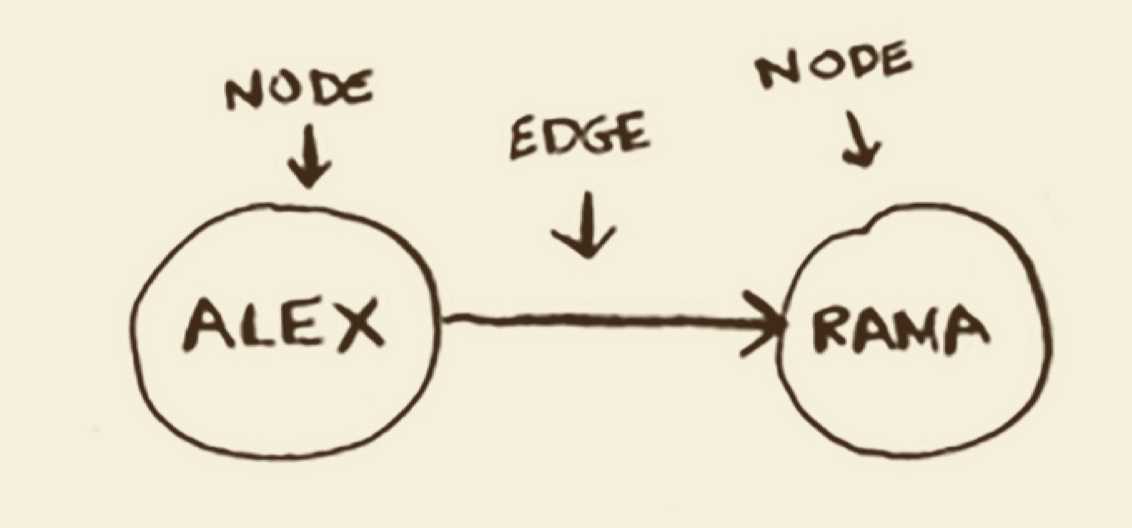
应用场景
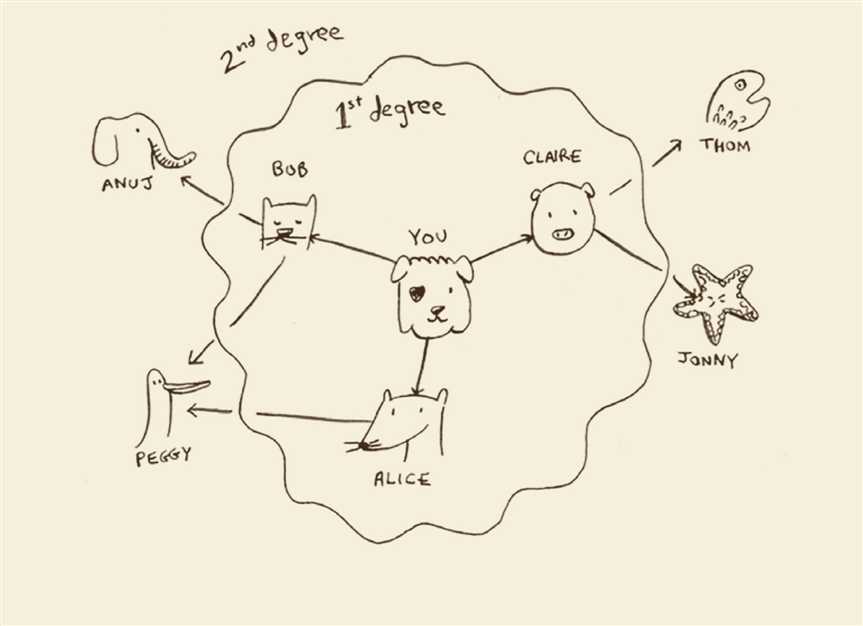
问题:你经营着一个芒果农场,你要找一个芒果销售商并把芒果卖给他。
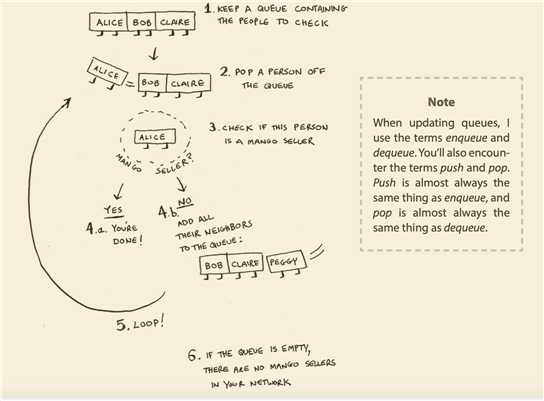
解决思路:你需要优先从你的朋友中开始找,朋友中没有,再从朋友的朋友中找,在找完所有朋友之前,不去找朋友的朋友。以此类推。如下图所示:
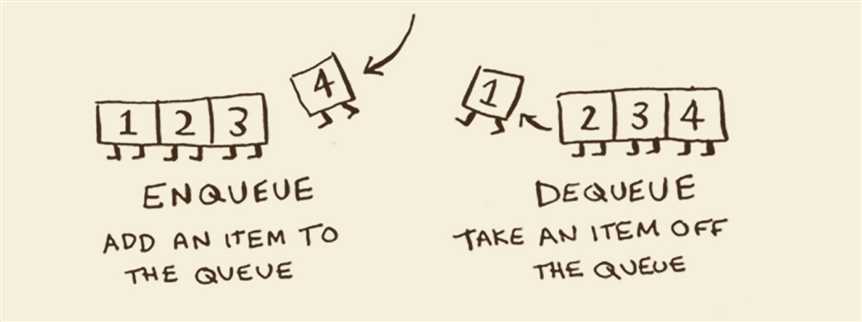
这就需要按顺序进行添加,并按顺序进行查找,有一种数据结构可以实现这个目的,就是队列。
队列的工作原理就和在医院排队挂号一张,排在前面的先挂号。
队列是一种先进先出(First In First Out)的数据结构,而栈是一种后进先出(Last In First Out,LIFO)的数据结构。
队列图示:

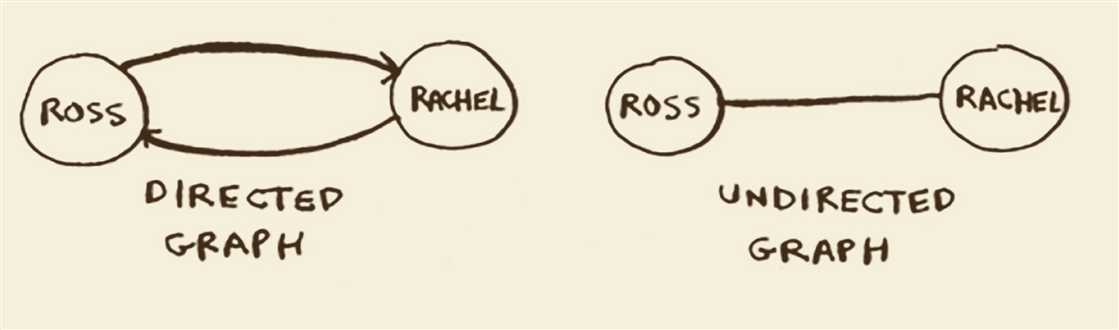
有箭头指向自己,但是没有从自己指向其他人的箭头,这被称为有向图(directed graph),其中的关系是单向的。 (Anuj、Peggy、Thom、Jonny)
直接相连的节点互为邻居,被称为无向图。
from collections import deque graph = {} graph[‘you‘] = [‘alices‘, ‘bobs‘, ‘claires‘] graph[‘bobs‘] = [‘anujs‘, ‘peggys‘] graph[‘alices‘] = [‘peggys‘] graph[‘claires‘] = [‘thoms‘, ‘jonnys‘, ‘jack‘] graph[‘anujs‘] = [] graph[‘peggys‘] = [] graph[‘thoms‘] = [] graph[‘jonnys‘] = [] graph[‘jack‘] = [‘lucy‘, ‘mango_andrew‘] graph[‘lucy‘] = [] graph[‘mango_andrew‘] = [] def person_is_seller(name): return ‘mango‘ in name def search(name): search_queue = deque() search_queue += graph[name] searched = [] while search_queue: person = search_queue.popleft() if person not in searched: if person_is_seller(person): print(person[6:] + ‘ is a mongo seller‘) return True else: search_queue += graph[person] searched.append(person) print(‘Not find mango seller‘) return False search(‘you‘)
顺便提一下,字典这么写是为了看起来更清晰,它的添加顺序对结果是没有影响的,因为散列表是无序的。
graph[‘you‘] = [‘alices‘, ‘bobs‘, ‘claires‘] graph[‘bobs‘] = [‘anujs‘, ‘peggys‘] # 写成下面这样对结果一点影响都没有 graph[‘bobs‘] = [‘anujs‘, ‘peggys‘] graph[‘you‘] = [‘alices‘, ‘bobs‘, ‘claires‘]
你在整个人际关系网中搜索芒果销售商,就意味着你需要沿每条边前行(一个人到另一个人的箭头),时间至少为O(边数)。将每一个人添加到队列的时间是O(1),因此广度搜索的运行时间为O(人数 + 边数),通常写作O(V+E),其中V为定点(vertice),E为边数。
标签:列表 sea 实现 arc queue alt 国际 图片 朋友
原文地址:https://www.cnblogs.com/lshedward/p/10480342.html