标签:tree dynamic programming leetcode
题目:
Given n, how many structurally unique BST‘s (binary search trees) that store values 1...n?
For example,
Given n = 3, there are a total of 5 unique BST‘s.
1 3 3 2 1
\ / / / \ 3 2 1 1 3 2
/ / \ 2 1 2 3
背景知识:
二叉查找树(英语:Binary Search Tree),也称二叉搜索树、有序二叉树(英语:ordered binary tree),排序二叉树(英语:sorted binary tree),是指一棵空树或者具有下列性质的二叉树:
Anwser 1: 递归方法
思路:数字1~n 可以构成多少种二叉查找树。
从1~n中遍历选取一个点i作为树的根,则左子树的组合numTrees(left) 和右子树的组合numTrees(right)乘积即为当前根的组合数。
根据二叉查找树的定义,左子树结点个数为(i-1),右子树为(n-i)。
AC Code:
class Solution {
public:
int numTrees(int n) {
//数字1~n 可以构成多少种二叉查找树
//递归方法 从1~n中遍历选取一个点i作为树的根,则左子树的组合numTrees(left) 和右子树的组合numTrees(right)乘积即为当前根的组合数。
//根据二叉查找树的定义,左子树结点个数为(i-1),右子树为(n-i)
if(n == 0 || n == 1)
{
return 1;
}
else
{
int ret = 0;
for(int i = 1; i <= n; i++) //i代表root
{
ret += (numTrees(i-1)) * (numTrees(n-i)); //left * right
}
return ret;
}
}
};思路:
分析问题,可知,假设f(n)表示1~n个结点给出时,BST‘s树的个数
f(n) = f(0) * f(n-1) // 令1作为根,2~n右子树
+ f(1) * f(n-2) // 令2作为根,1作为左子树,3~n右子树
+ ........
+ f(n-1)* f(0) //令n作根,1~n-1作为左子树。
递归形式符合卡塔兰数模型。
卡塔兰数:
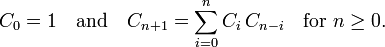
维护result[i]表示含有i个结点的二叉查找树的数量。然后依据上面分析的递推公式依次求出f(1), f(2),......f(n)。
Attention:
1. 初始化容器前两项,后面才能开始迭代。
result.push_back(1);
result.push_back(1);
2. 计算含i个结点时二叉查找树的种类数,j表示1~i中取任一结点作为根。所以计算方式同递归一样,左子树(J-1)*右子树(I-J)
3. 实现递推公式
sum += (result[j-1] * result[i-j]);
4. 维护一个result的容器,容器最末尾的数即为所求F(N). 所以每次计算时需先重置0,迭代得到结果后再push进容器。
for(int i = 2; i <= n; i++)
{
int sum = 0;
//计算含i个结点时二叉查找树的种类数,j表示1~i中取任一结点作为根。
for(int j = 1; j <= i; j++)
{
sum += (result[j-1] * result[i-j]); //左树*右树 实现递推公式
}
result.push_back(sum);
}
复杂度:每次求解i个结点的二叉查找树数量的需要一个i步的循环,总体需要n次。总时间复杂度O(1+2+3+....+n) = O(N^2)
空间需要一个容器维护,并且需要前i个的所有信息,所以是O(N)
AC Code:
class Solution {
public:
int numTrees(int n) {
vector<int> result;
result.push_back(1);
result.push_back(1);
//二叉查找树种类总数是以所有结点为根的可行结果累加起来
for(int i = 2; i <= n; i++)
{
int sum = 0;
//计算含i个结点时二叉查找树的种类数,j表示1~i中取任一结点作为根。
for(int j = 1; j <= i; j++)
{
sum += (result[j-1] * result[i-j]); //左树*右树 实现递推公式
}
result.push_back(sum);
}
return result.back();
}
};[C++]LeetCode: 53 Unique Binary Search Trees
标签:tree dynamic programming leetcode
原文地址:http://blog.csdn.net/cinderella_niu/article/details/42107921