标签：
Mr. Kitayuta has just bought an undirected graph consisting of n vertices and m edges. The vertices of the graph are numbered from 1 to n. Each edge, namely edge i, has a color ci, connecting vertex ai and bi.
Mr. Kitayuta wants you to process the following q queries.
In the i-th query, he gives you two integers — ui and vi.
Find the number of the colors that satisfy the following condition: the edges of that color connect vertex ui and vertex vi directly or indirectly.
The first line of the input contains space-separated two integers — n and m (2?≤?n?≤?100,?1?≤?m?≤?100), denoting the number of the vertices and the number of the edges, respectively.
The next m lines contain space-separated three integers — ai, bi (1?≤?ai?<?bi?≤?n) and ci (1?≤?ci?≤?m). Note that there can be multiple edges between two vertices. However, there are no multiple edges of the same color between two vertices, that is, if i?≠?j, (ai,?bi,?ci)?≠?(aj,?bj,?cj).
The next line contains a integer — q (1?≤?q?≤?100), denoting the number of the queries.
Then follows q lines, containing space-separated two integers — ui and vi (1?≤?ui,?vi?≤?n). It is guaranteed that ui?≠?vi.
For each query, print the answer in a separate line.
4 5 1 2 1 1 2 2 2 3 1 2 3 3 2 4 3 3 1 2 3 4 1 4
2 1 0
5 7 1 5 1 2 5 1 3 5 1 4 5 1 1 2 2 2 3 2 3 4 2 5 1 5 5 1 2 5 1 5 1 4
1 1 1 1 2
Let‘s consider the first sample.
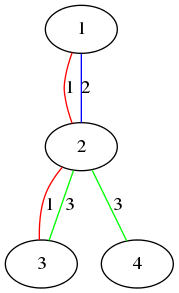The figure above shows the first sample.
<pre name="code" class="cpp">#include <iostream>
#include<cstdio>
#include<cstring>
using namespace std;
int Mat[102][102][102];
int main()
{
int n,m;
while(~scanf("%d%d",&n,&m))
{
memset(Mat,0,sizeof(Mat));
for(int i=1; i<=m; i++)
{
int a,b,c;
scanf("%d%d%d",&a,&b,&c);
Mat[a][b][c]=1;
Mat[b][a][c]=1;
}
for(int c=1;c<=m;c++){
for(int k=1;k<=n;k++){
for(int i=1;i<=n;i++)
for(int j=1;j<=n;j++){
if(Mat[i][k][c]&&Mat[k][j][c]){
Mat[i][j][c]=1;
Mat[j][i][c]=1;
}
}
}
}
int q;
scanf("%d",&q);
for(int i=0;i<q;i++){
int a,b;
int sum=0;
scanf("%d%d",&a,&b);
for(int i=1;i<=m;i++){
sum+=Mat[a][b][i];
}
printf("%d\n",sum);
}
}
return 0;
}Codeforces Round #286 (Div. 2) B. Mr. Kitayuta's Colorful Graph +foyd算法的应用
标签：
原文地址：http://blog.csdn.net/u012870383/article/details/43015693
