标签:
As you know, an undirected connected graph with n nodes and n - 1 edges is called a tree. You are given an integer d and a tree consisting of n nodes. Each node i has a value ai associated with it.
We call a set S of tree nodes valid if following conditions are satisfied:
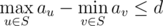 .
.Your task is to count the number of valid sets. Since the result can be very large, you must print its remainder modulo1000000007 (109 + 7).
The first line contains two space-separated integers d (0 ≤ d ≤ 2000) and n (1 ≤ n ≤ 2000).
The second line contains n space-separated positive integers a1, a2, ..., an(1 ≤ ai ≤ 2000).
Then the next n - 1 line each contain pair of integers u and v (1 ≤ u, v ≤ n) denoting that there is an edge between u and v. It is guaranteed that these edges form a tree.
Print the number of valid sets modulo 1000000007.
1 4
2 1 3 2
1 2
1 3
3 4
8
0 3
1 2 3
1 2
2 3
3
4 8
7 8 7 5 4 6 4 10
1 6
1 2
5 8
1 3
3 5
6 7
3 4
41
In the first sample, there are exactly 8 valid sets: {1}, {2}, {3}, {4}, {1, 2}, {1, 3}, {3, 4} and {1, 3, 4}. Set{1, 2, 3, 4} is not valid, because the third condition isn‘t satisfied. Set {1, 4} satisfies the third condition, but conflicts with the second condition.
题意:
给定一棵树,树有点权,现在有树中有多少个有效的集合
有效的集合:
1.集合非空
2.集合是连通的,也就是说集合组成的还是一棵树
3.集合中,最大点权-最下点权<=d
这道题暑假的时候有想过,没有想出来
今天一想,其实就是一道简单的计数问题
由于n很小,O(n^2)是可以的
要max-min<=d
也就是要max<=min+d
dp[i]:i在集合里面,并且集合的最小点权就是i的点权的有效集合的个数
则:ans=sigma(dp[i])
对于一个节点root,我们考虑这个点的点权是他所在的有效集合中的最小点权,并且以root为根开始进行树形DP
如果节点i的点权>=a[root]&& 点权<=a[root]+d
我们就认为root可以扩展到i,不断扩展
并且有dp[u]=dp[u]*(1LL+dp[v])%mod
这样dfs一遍就可以在O(n)算出dp[root]了
以每一个点作为root 来dfs一遍,累加就可以得到ans了
注意一个问题:
有可能a[u]==a[v]
我们以root=u时扩展到v,并且加入了v,算了一遍
然后以root=v时扩展到u,这个时候我们如果把u加入,就会重复计算了
那么在有多个点的点权相等时,我们怎么避免重复计算,只算一次呢?
其实只要我们设一个数组vis[i][j],算第一次的时候我们把数组标记为true,后面就不再加入了
#include<cstdio> #include<cstring> #include<algorithm> #include<iostream> #define LL long long using namespace std; const int maxn=2010; const int mod=1e9+7; LL dp[maxn]; int a[maxn]; bool vis[maxn][maxn]; int sum; int root; struct Edge { int to,next; }; Edge edge[maxn<<1]; int head[maxn]; int tot; void init() { memset(head,-1,sizeof head); tot=0; } void addedge(int u,int v) { edge[tot].to=v; edge[tot].next=head[u]; head[u]=tot++; } void solve(int ,int d); int main() { init(); int n,d; scanf("%d %d",&d,&n); for(int i=1;i<=n;i++){ scanf("%d",&a[i]); } for(int i=1;i<n;i++){ int u,v; scanf("%d %d",&u,&v); addedge(u,v); addedge(v,u); } solve(n,d); return 0; } void dfs(int u,int pre) { dp[u]=1; for(int i=head[u];~i;i=edge[i].next){ int v=edge[i].to; if(v==pre || a[v]<a[root] || a[v]>sum) continue; if(a[v]==a[root]){ if(!vis[v][root]){ vis[v][root]=true; vis[root][v]=true; dfs(v,u); } else continue; } else{ dfs(v,u); } dp[u]=dp[u]*(1LL+dp[v])%mod; } } void solve(int n,int d) { memset(vis,false,sizeof vis); LL ans=0; for(int i=1;i<=n;i++){ sum=a[i]+d; root=i; dfs(root,root); ans=(ans+dp[root])%mod; ans=(ans+mod)%mod; //cout<<dp[root]<<endl; } printf("%I64d\n",ans); return ; }
标签:
原文地址:http://www.cnblogs.com/-maybe/p/4874223.html