标签:hdu1071
The area
Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 65536/32768 K (Java/Others)
Total Submission(s): 7476 Accepted Submission(s): 5222
Problem Description
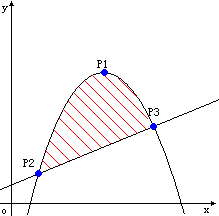
Ignatius bought a land last week, but he didn‘t know the area of the land because the land is enclosed by a parabola and a straight line. The picture below shows the area. Now given all the intersectant points shows in the picture,
can you tell Ignatius the area of the land?
Note: The point P1 in the picture is the vertex of the parabola.
Input
The input contains several test cases. The first line of the input is a single integer T which is the number of test cases. T test cases follow.
Each test case contains three intersectant points which shows in the picture, they are given in the order of P1, P2, P3. Each point is described by two floating-point numbers X and Y(0.0<=X,Y<=1000.0).
Output
For each test case, you should output the area of the land, the result should be rounded to 2 decimal places.
Sample Input
2
5.000000 5.000000
0.000000 0.000000
10.000000 0.000000
10.000000 10.000000
1.000000 1.000000
14.000000 8.222222
Sample Output
33.33
40.69
Hint
For float may be not accurate enough, please use double instead of float.
题意:给定一个抛物线的顶点P1坐标和另外两点坐标P2、P3,以及一条穿过P2、P3的直线。求直线与抛物线围城的面积。
题解:每次做磊哥出的题总会学到些新东西,这次也不例外。这是我第一次做积分的题。高数总算是没白学。但是已知抛物线三点坐标求抛物线的方程我愣是没解出来,感觉太复杂了,在网上找的别人推出好的公式:click
here
#include <stdio.h>
int main()
{
int t;
double x1, y1, x2, y2, x3, y3, a, b, c, k, d, p, q, r, ans;
scanf("%d", &t);
while(t--){
scanf("%lf%lf%lf%lf%lf%lf", &x1, &y1, &x2, &y2, &x3, &y3);
p = y1 / ((x1 - x2) * (x1 - x3));
q = y2 / ((x2 - x1) * (x2 - x3));
r = y3 / ((x3 - x1) * (x3 - x2));
a = p + q + r;
b = -p*(x2 + x3) - q*(x1 + x3) - r * (x1 + x2);
c = p * x2 * x3 + q * x1 * x3 + r * x1 * x2;
k = (y3 - y2) / (x3 - x2);
d = y2 - k * x2;
ans = (1.0/3*a*x3*x3*x3 + 1.0/2*(b-k)*x3*x3 + (c-d)*x3) -
(1.0/3*a*x2*x2*x2 + 1.0/2*(b-k)*x2*x2 + (c-d)*x2);
if(ans < 0) ans = -ans;
printf("%.2lf\n", ans);
}
return 0;
}
HDU1071 The area 【积分】
标签:hdu1071
原文地址:http://blog.csdn.net/chang_mu/article/details/38060839
