标签:
In the following equation x, y, and n are positive integers.
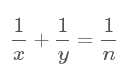
For n = 4 there are exactly three distinct solutions:
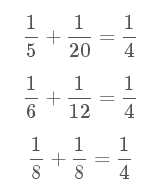
It can be verified that when n = 1260 there are 113 distinct solutions and this is the least value of n for which the total number of distinct solutions exceeds one hundred.
What is the least value of n for which the number of distinct solutions exceeds four million?
NOTE: This problem is a much more difficult version of Problem 108 and as it is well beyond the limitations of a brute force approach it requires a clever implementation.
在如下方程中,x、y、n均为正整数。
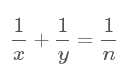
对于n = 4,上述方程恰好有3个不同的解:
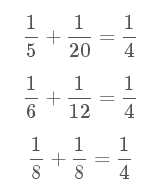
可以验证当n = 1260时,恰好有113种不同的解,这也是不同的解的总数超过一百种的最小n值。
不同的解的总数超过四百万种的最小n值是多少?
注意:这是第108题一个极其困难的版本,而且远远超过暴力解法的能力范围,因此需要更加聪明的手段。
解题
先占坑
待完善
Project Euler 110:Diophantine reciprocals II 丢番图倒数II
标签:
原文地址:http://www.cnblogs.com/theskulls/p/5155229.html