CFD冲蚀模拟的一些理论

时间：2016-03-26 23:33:26 阅读：2689 评论：0 收藏：0 [点我收藏+]

标签：

1 冲蚀速率（Erosion Rate）
2 STAR CCM+中的冲蚀率模型
2.1 Ahlert Correlation
2.2 DNV Correlection
2.3 Neilson-Gilchrist Correlation
2.4 Oka Correlation
2.5 DEM中的磨蚀模型
3 FLUENT中的冲蚀模型
4 CFX中的冲蚀模型
4.1 Finnie模型
4.2 CFX中使用Finnie模型
4.2 Tabakoff and Grant模型
4.4 CFX中使用Tabakoff and Grant模型
参考文献

在CFD中计算颗粒对固体壁面的冲蚀往往采用冲蚀模型（Erosion Model）。

1 冲蚀速率（Erosion Rate）

冲蚀速率定义为壁面材料在单位时间单位面积上损失的质量（单位： $k g / (m^{2} \cdot s)$ ）。通过计算每一个颗粒对壁面的累积损伤来计算冲蚀速率。

E_{f} = \frac{1}{A_{f}} \sum_{π (f)} {\dot{m}}_{π} e_{r}

式中：

$A_{f}$ 为网格单元面积
${\dot{m}}_{π}$ 为冲击壁面的颗粒质量流量
$e_{r}$ 为冲蚀率

冲蚀速率取决于颗粒流动状态（以何种方式冲击壁面）以及选择的冲蚀率。

2 STAR CCM+中的冲蚀率模型

STAR CCM+内置了四种常用的冲蚀率：

Ahlert Correlation
DNV Correlation
Neilson-Gilchrist Correlation
Oka Correlation
这四种模型定义了由于颗粒直接冲击壁面造成的冲击损伤，还可以利用Archard Correlation则定义了由于颗粒切向或以低角度冲击壁面造成的磨蚀。

2.1 Ahlert Correlation

Ahlert Correlation[1]定义冲蚀率为：

E_{r} = K F_{s} f (α) (\frac{u_{r e l}}{u_{r e f}})^{n}

式中：

$K$ 为与材料相关的常数
$F_{s}$ 为颗粒形状因子。对于完全球形颗粒取0.2，对于半圆形颗粒取0.5，不规则颗粒取1
$f (α)$ 为颗粒冲击角函数。冲击函数被分为两部分：冲击角低于转变角度 $α_{0}$ 时表示为冲击角 $α$ 的多项式函数（角度单位为弧度），高于转变角度时，遵循三角函数关系：
$f (α) = x c o s^{2} α s i n ω α + y s i n^{2} α + z$
式中常数 $ω$ 、 $x$ 及 $y$ 均为用户自定义，Z值为程序内部计算得到以满足曲线的连续性。
$u_{r e l}$ 为粒子与壁面间的相对速度， $| u_{r e f} |, u_{r e f} = u_{p a r t i c l e} - u_{w a l l}$
$u_{r e f}$ 为颗粒参考速度常数
{n}为指数

STAR CCM+中Ahlert correlation默认参数为液体驱动半圆形砂颗粒侵蚀铝，参数采用文献[2]。

2.2 DNV Correlection

DNV Correlection[3]对于冲蚀率表示为：

e_{r} = K f (θ) (\frac{u_{r e l}}{u_{r e f}})^{n}

式中：

$K$ 为材料相关阐述，对于普通钢材，其默认参数为2.0e-9
$f (θ)$ 为粒子冲击角函数，默认形式为：
$f (θ) = A θ + B θ^{2} + C θ^{3} + D θ^{4} + E θ^{5} + F θ^{6} + G θ^{7} + H θ^{8}$
其中关于系数值：

参数	取值
A	9.37
B	-42.295
C	110.864
D	-175.804
E	170.137
F	-98.398
G	31.211
H	-4.170

$θ$ 取值为（0， $\frac{π}{2}$ ），单位为弧度

$u_{r e l}$ 为粒子与壁面间的相对速度， $| u_{r e f} |, u_{r e f} = u_{p a r t i c l e} - u_{w a l l}$
$u_{r e f}$ 为颗粒参考速度常数，默认值1m/s
{n}为指数，默认值2.6
DNV修正模型的默认系数是基于空气驱动砂粒侵蚀碳钢，数据来源于文献[4]。DNV修正模型并不显式的基于颗粒粒径，然而，本修正模型时基于平均粒径 $225 μ m$ 条件下的实验测量数据。

2.3 Neilson-Gilchrist Correlation

Neilson-Gilchrist Correlation[5]将冲蚀率描述为：

e_{r} = e_{r C} + e_{r D}

式中，

e_{r C}

及

e_{r D}

分别表示切削量与变形量。切削冲蚀率表示为冲击角

α

的函数：

式中：

$α_{0}$ 为用户自定义的转换角
$ε_{C}$ 为用户指定的剪切系数
$u_{r e l}$ 为粒子与壁面间的相对速度， $| u_{r e f} |, u_{r e f} = u_{p a r t i c l e} - u_{w a l l}$

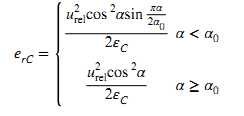
变形冲蚀定义为：

e_{r D} = \frac{m a x (u_{r e l} s i n α ? K, 0)^{2}}{2 ε_{D}}

式中，

ε_{D}

为变形系数，

K

为切除速度。
默认参数为液体驱动砂粒侵蚀AISI4130钢，数据来源文献[6]

2.4 Oka Correlation

Oka Correlation[7][8]将冲蚀率表述为：

e_{r} = e_{90} g (α) (\frac{u_{r e l}}{u_{r e f}})^{k_{2}} (\frac{D_{p}}{D_{r e f}})^{k_{3}}

式中，

角度函数 $g (α)$ 定义为：
$g (α) = (s i n α)^{n_{1}} (1 + H_{v} (1 ? s i n α))^{n_{2}}$
其中， $n_{1}$ 、 $n_{2}$ 及 $H_{v}$ 为用户指定常数。 $H_{v}$ 为材料的维氏硬度，单位 $G P a$
$u_{r e l}$ 为粒子与壁面间的相对速度， $| u_{r e f} |, u_{r e f} = u_{p a r t i c l e} - u_{w a l l}$
$u_{r e f}$ 为颗粒参考速度常数
$D_{ref}为用户指定的参考粒径
$k_{2}$ 与 $k_{3}$ 为指定的指数
$e_{90}$ 定义为参考冲蚀率，其为 $u_{r e l} = u_{r e f}$ ， $D_{p} = D_{r e f}$ 及 $α = 90^{\circ}$ 时的冲蚀率。在STAR CCM+中， $e_{90}$ 的值为Oka及DNV模型在 $90^{\circ}$ 情况下的冲蚀率。参考粒径 $D_{r e f} = 326 μ m$ ，参考速度104m/s，
$e_{90} = e_{r, D N V @ 90} (D_{r e f} / D_{p})^{k_{3}}$
Oka模型可以用于不同材质的材料冲蚀模拟，比如说可以考虑砂-钢冲蚀及砂-铝冲蚀，不同的是材料的维氏硬度不同。

Oka模型的默认参数来自于空气驱动的砂粒侵蚀0.25%碳钢，默认参数来自于文献[7][8]，除了 $e_{90}$ 来自于DNV模型。

2.5 DEM中的磨蚀模型

STAR CCM+中DEM模型计算磨蚀（Abrasive Rate）采用Archard Correlation模型。
磨蚀（Abrasive erosion）利用磨蚀模型（Abrasive Wear model）及磨蚀率（Abrasive Wear Rate)来表示。该模型目前可以用于DEM及EMP。磨蚀主要有颗粒切向与壁面接触及以低角度冲击壁面所造成。磨蚀速率可描述为：

E_{f} = \frac{1}{A_{f} d t} \sum_{π (f)} e_{r}

式中：

$d t$ 为流动时间步长
$e_{r}$ 为利用Archard correlation模型[9]计算的磨蚀率

Archard Correlation模型描述磨蚀率为：

e_{r} = a F s

式中：

$a$ 为磨蚀系数，默认值为0.01kg/J（建议值为1e-8~1e-2，从轻微磨蚀到严重磨蚀）
$F$ 为法向力
$s$ 为滑移距离

3 FLUENT中的冲蚀模型

在FLUENT中模拟颗粒冲蚀比较简单，其定义冲蚀速率为:

R_{e r o s i o n} = \sum_{p = 1}^{N_{p a r t i c l e}} \frac{\dot{m_{p}} C (d_{p}) f (α) v^{b (v)}}{A_{f a c e}}

式中：

$C (d_{p})$ 为颗粒粒径函数
$α$ 为颗粒路径与壁面间冲击角
$f (α)$ 为冲击角函数
$v$ 为颗粒相对速度
$b (v)$ 为颗粒相对速度函数
$A_{f a c e}$ 为壁面面积

默认值：

$C = 1.8 \times - 9$
$f = 1$
$b = 0$
需要注意的是，参数 $C$ 、 $f$ 以及 $b$ 均定义为壁面的边界条件，而不是定义为材料属性，因此默认参数值无法反应所使用的真实值。因此，在实际应用过程中应当为这些参数值赋予真实的值。对于颗粒侵蚀碳钢及铝材的数据可参加文献[10].
参数 $C$ 、 $f$ 以及 $b$ 需要定义为分段线性、分段多项式或多项式函数以将其定义为边界条件。因此，在实际工作中很有必要在文献中找寻合适的函数。

上述公式计算的冲蚀速率为单位时间单位面积去除的材料质量。

The erosion rate as calculated above is displayed in units of removed material/(area-time), that is, mass flux, and can therefore be changed accordingly to the defined units in ANSYS Fluent. The functions and have to be specified in consistent units to build a dimensionless group with the relative particle velocity and its exponent. To compute an erosion rate in terms of length/time (mm/year, for example) you can either define a custom field function to divide the erosion rate by the density of the wall material or include this division in the units for and/or . Note that the units given by ANSYS Fluent when displaying the erosion rate are no longer valid in the latter case.

在ANSYS FLUENT中很容易实现不同的冲蚀模型如文献[11][12][13]14][15][16]中包含的模型常数[15][13]以及角度函数。这些方程所描述的冲蚀模型很容易修改为通用冲蚀速率模型。
例如：文献[13]中描述的Tusla Angle Dependent模型：

E R = 1.559 e^{? 6} B^{? 0.59} F_{s} v^{1.73} f (α)

通过改写：

v^{1.73} = v^{b (v)}

1.559 e^{? 6} B^{? 0.59} F_{s} = C (d_{p})

式中，

E R

为冲蚀率（Erosion Ratio），

B

为布氏硬度，

F_{s}

为颗粒形状系数。Tusla模型推荐尖角砂粒形状系数取1，半圆形砂粒取0.53，球形砂粒取0.2.

冲击角函数可以通过分段线性函数进行拟合。下面是一个砂粒冲蚀钢的冲击角函数：

f (α) = {\begin{cases} 0 + 22.7 α ? 38.4 α^{2} & α \leq 0.267 r a d \\ 2.00 + 6.80 α ? 7.50 α^{2} + 2.25 α^{3} & α > 0.267 r a d \end{cases}

对于更复杂的模型，还可以是用UDF宏DEFINE_DPM_EROSION来定义冲蚀模型。

4 CFX中的冲蚀模型

CFX中对于冲蚀的模拟主要有两种模型：

Finnie模型
Tabakoff and Grant模型

4.1 Finnie模型

壁面由于颗粒的冲蚀效应造成的磨损是关于颗粒冲击、颗粒及壁面属性的复杂函数。对于大多数金属，冲蚀可认为是粒子冲击角及速度之间的函数[17]：

E = k V_{p}^{n} f (γ)

式中， $E$ 为无量纲质量， $V_{p}$ 为颗粒冲击速度， $f (γ)$ 为冲击角的无量纲函数。冲击角为颗粒轨迹与壁面的夹角(以弧度为单位)，指数 $n$ 通常取2.3-2.5（金属）。

Finnie模型[18]定义冲蚀速率为冲击到壁面上的颗粒动能的函数（ $n = 2$ ），其表示为：

E = k V_{p}^{2} f (γ)

式中：

f (γ) = {\begin{cases} \frac{1}{3} c o s^{2} γ & t a n γ > \frac{1}{3} \\ s i n (2 γ) ? 3 s i n^{2} γ & t a n γ \leq \frac{1}{3} \end{cases}

4.2 CFX中使用Finnie模型

在CFX中使用Finnie模型，需要调整系数 $k$ 以获得无量纲冲蚀因子：

E = (\frac{V_{p}}{V_{0}})^{n} f (γ)

式中，

V_{0} = (\frac{1}{\sqrt[n]{k}})

，一些典型材料的

V_{0}

如下表所示：

$V_{0}$	壁面材料
952[m/s]	铝材
661[m/s]	铜
1310[m/s]	低碳钢
3321[m/s]	淬火钢

4.2 Tabakoff and Grant模型

在Tabakoff and Grant模型中，冲蚀速率 $E$ 表示为：

E = k_{1} f (γ) v_{p}^{2} c o s^{2} γ [1 ? R_{T}^{2}] + f (V_{P N})

其中：

f (γ) = [1 + k_{2} k_{12} s i n (γ \frac{π / 2}{γ_{0}})]^{2} R_{T} = 1 ? k_{4} V_{p} s i n γ f (V_{P N} = k_{3} (V_{p} s i n γ)^{4} k_{2} = {\begin{cases} 1.0 & γ \leq 2 γ_{0} \\ 0.0 & γ > 2 γ_{0} \end{cases}

4.4 CFX中使用Tabakoff and Grant模型

原始Tabakoff and Grant模型中的一些常数仅适用于颗粒速度以ft/s形式指定，在CFX中对原始模型进行了修订：

E = F (γ) (\frac{V_{p}}{V_{1}})^{2} c o s^{2} γ (1 ? R_{T}^{2}) + f (V_{P N}) 其 中 ： f (γ) = [1 + k_{2} k_{12} s i n (γ \frac{π / 2}{γ_{0}}]^{2} R_{T} = 1 ? \frac{V_{p}}{V_{3}} s i n γ f (V_{P N}) = (\frac{V_{p}}{V_{2}} s i n γ)^{4} k_{2} = {\begin{cases} 1.0 & γ \leq 2 γ_{0} \\ 0.0 & γ > 2 γ_{0} \end{cases}

The Tabakoff model typically returns an erosive wear with the dimensions milligrams of eroded material per gram of colliding particles. In CFX, this variable is converted into grams of eroded material per gram of colliding particles.

CFX中的变量与原始模型变量对照如下表所示：

变量	量纲	CFX变量
$k_{12}$	无量纲	K12常数
$k_{2}$	无量纲
$V_{1}$	[Velocity]	参考速度1
$V_{2}$	[Velocity]	参考速度2
$V_{3}$	[Velocity]	参考速度3
$γ_{0}$	[deg]	最大冲蚀角

其中：

V_{1} = 1 / \sqrt{k_{1}} V_{2} = 1 / (\sqrt[4]{k_{3}}) V_{3} = 1 / k_{3}

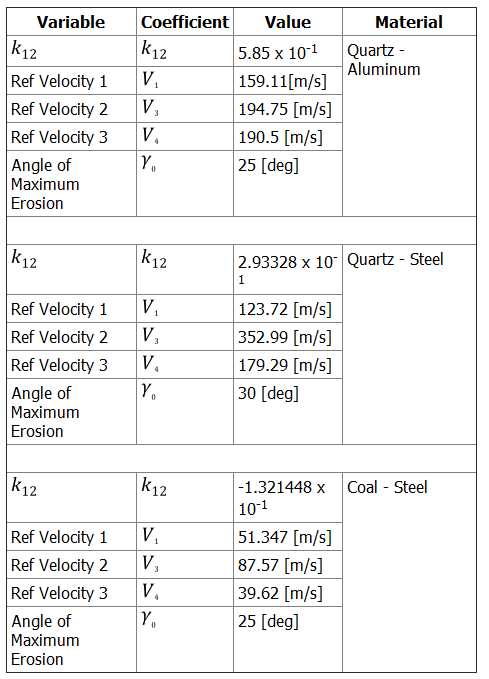
在CFX中使用Tabakoff模型需要5个参数，下面是一些材料冲蚀的参数，包括石英-铝、石英-钢、煤-钢的冲蚀数据。
技术分享

参考文献

[1] Ahlert, K. 1994. “Effects of particle impingement angle and surface wetting on solid particle erosion of AISI 1018 steel”, MS Thesis,where: University of Tulsa, USA.
[2] McLaury, B.S., Shirazi, S.A., Shadley, J.R., and Rybicki, E.F. 1996. “Modeling erosion in chokes”, ASME FED conference, 236(1), pp. 773–781.
[3] Haugen, K., Kvernvold, O., Ronold, A., and Sandberg, R. 1995. “Sand erosion of wear-resistant materials: Erosion in choke valves”, Wear, 186-187, pp. 179–188.
[4] Haugen, K., Kvernvold, O., Ronold, A., and Sandberg, R. 1995. “Sand erosion of wear-resistant materials: Erosion in choke valves”, Wear, 186-187, pp. 179–188.
[5] Neilson, J.H., and Gilchrist, A. 1968. “Erosion by a stream of solid particles”, Wear, 11, pp.111–122.
[6] Wallace, M.S., Peters, J.S., Scanlon, T.J., Dempster, W.M., McCulloch, S., and Ogilvie, J.B.2000. “CFD-based erosion modeling of multi-orifice choke valves”, ASME, Paper FEDSM2000-11244.
[7] Oka, Y.I, Okamura, K., and Yoshida, T. 2005. “Practical estimation of erosion damage caused by solid particle impact. Part 1: Effect of impact parameters on a predictive equation”, Wear, 259, pp. 95–101.
[8] Oka, Y.I., and Yoshida, T. 2005. “Practical estimation of erosion damage caused by solid particle impact. Part 2: Mechanical properties of materials directly associated with erosion damage”, Wear, 259, pp. 102–109.
[9] Archard, J. F., and Hirst, W. 1956. “The wear of metals under unlubricated conditions”,Proceedings of the Royals Society of London. Series A, Mathematical and Physical Sciences. 236(1206), pp 397-410.
[10] J. K. Edwards, B. S. McLaury, and S. A.Shirazi. “Evaluation of Alternative Pipe Bend Fittings in Erosive Service”. In Proceedings of ASME FEDSM’00: ASME 2000 Fluids Engineering Division Summer Meeting, Boston, MA. June 2000.
[11] I. Finnie. “Erosion of Surfaces by Solid Particles”. Wear. 3. 87–103. 1960.
[12] B. S. McLaury, J. Wang, S. A. Shirazi, J. R. Shadley, and E. F. Rybicki. “Solid Particle Erosion in Long Radius Elbows and Straight Pipes”. SPE Annual Technical Conference and Exhibition, II Production Operations and Engineering/General, San Antonio, Texas. SPE Paper 38842 October 1997.
[13] J. K. Edwards, B. S. McLaury, and S. A. Shirazi. “Supplementing a CFD Code with Erosion Prediction Capabilities”. In Proceedings of ASME FEDSM’98: ASME 1998 Fluids Engineering Division Summer Meeting, Washington DC. June 1998.
[14] L. Nokleberg and T. Sontvedt. “Erosion of Oil and Gas Industry Choke Valves Using Computational Fluid Dynamics and Experiment”. International Journal of Heat and Fluid Flow. 636–643. 1998.
[15] K. Haugen, O. Kvernvold, A. Ronald, and R. Sandberg. “Sand Erosion of Wear-resistant Materials: Erosion in Choke Valves.Wear”. 186-187. 179–188. 1995.
[16] M. M. Salama and E. S. Venkatesh. “Evaluation of api rp14e erosional velocity limitations for offshore gas wells”. In OTC Conference, Houston, TX. 371–376. May 1983.
[17]Hutchings, I.M.,“Mechanical and metallurgical aspects of the erosion of metals”,Proc. Conf. on Corrosion-Erosion of Coal Conversion System Materials, NACE (1979) 393.
[18]Dosanjh, S., and Humphrey, J.A.C.,“The influence of turbulence C on erosion by a particle laden fluid jet, Wear”,V.102, 1985, pp. 309-330.

来自为知笔记(Wiz)

CFD冲蚀模拟的一些理论

标签：

原文地址：http://www.cnblogs.com/LSCAX/p/5324255.html

踩

(0)

(3)

评论一句话评论（0）

分享档案

更多>

2021年07月29日 (22)
2021年07月28日 (40)
2021年07月27日 (32)
2021年07月26日 (79)
2021年07月23日 (29)
2021年07月22日 (30)
2021年07月21日 (42)
2021年07月20日 (16)
2021年07月19日 (90)
2021年07月16日 (35)

周排行