标签:个数 first mat bin n个元素 ges lucas定理 倒数 height
从m个不同元素中,任取n(n≤m)个元素并成一组,叫做从m个不同元素中取出n个元素的一个组合;
从m个不同元素中取出n(n≤m)个元素的所有组合的个数,叫做从m个不同元素中取出n个元素的组合数。
combinatorial number
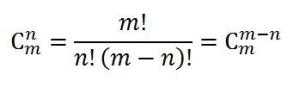
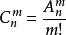 /
/ 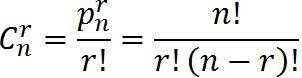
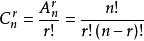

在线性写法中被写作C(m,n)。
c(m,n)=p(m,n)/n!=m!/((m-n)!*n!)
组合数的计算:C(n,k)有多种解法,1,dp递推;2,直接计算;3,lucas定理
性质:
性质1 C(n,m)= C(n,n-m)互补性质
例如C(9,2)=C(9,7),即从9个元素里选择2个元素的方法与从9个元素里选择7个元素的方法是相等的。
规定:C(m,0)=1
性质2 C(n,m)= C(n-1,m-1)+C(n-1,m) 组合恒等式
摘自http://blog.csdn.net/wty__/article/details/20048467
1.最简单的情况,数据比较小,直接采用C(a, b) = a * (a - 1) *....* (a - b + 1) / (b * (b - 1) *...* 2 * 1)
试用数据范围:a <= 29。在a = 30, b = 15时,计算分子乘机时即超范围
1 LL combi(LL a,LL b)///从a中取b 2 { 3 if(a<b){ 4 return 0; 5 } 6 LL r=1; 7 for(int i=a;i>=a-b+1;i--) 8 r*=i; 9 for(int j=b;j>1;j--) 10 r/=j; 11 return r; 12 }
2.采用分解质因子的方式,可以计算足够大的数(因为数字会超过long long的范围,所以结果依然用质因子表示,模板中计算出了相应的数)
1 #include <iostream> 2 #include "cstdio" 3 #include "map" 4 #include "cmath" 5 using namespace std; 6 #define LL long long 7 map <int, LL> m; 8 9 ///分解质因数 10 ///k为1或-1 11 void fun(int n, int k) 12 { 13 for (int i = 2; i <= sqrt(n * 1.0); i++) 14 { 15 while (n % i == 0) 16 { 17 n /= i; 18 m[i] += k;///存储因数+次数(k=-1倒数) 19 } 20 } 21 if (n > 1) 22 { 23 m[n] += k; 24 } 25 } 26 27 ///快速幂 28 LL quick_pow(LL a, LL b) 29 { 30 31 LL ret = 1; 32 while (b) 33 { 34 if (b & 1) 35 { 36 ret *= a; 37 } 38 b >>= 1; 39 a *= a; 40 } 41 return ret; 42 } 43 44 ///求组合数 45 LL C(LL a, LL b) 46 { 47 if (a < b || a < 0 || b < 0) 48 return 0; 49 m.clear(); 50 LL ret = 1; 51 b = min(a - b, b); 52 for (int i = 0; i < b; i++) 53 { 54 fun(a - i, 1);///分母 55 } 56 for (int i = b; i >= 1; i--) 57 { 58 fun(i, -1);///分子 59 } 60 61 ///以下计算出了具体的数 62 for (__typeof(m.begin()) it = m.begin(); it != m.end(); it++) 63 { 64 if ((*it).second != 0) 65 { 66 ret *= quick_pow((*it).first, (*it).second);///快速幂,在之前分解质因数时 在过程+(-1)中其实已经运算了一部分,所以才不容易乘过界。 67 } 68 } 69 return ret; 70 } 71 int main() 72 { 73 LL a,b; 74 while(~scanf("%lld%lld",&a,&b)&&a&&b) 75 { 76 cout<<C(a,b)<<endl; 77 } 78 }
标签:个数 first mat bin n个元素 ges lucas定理 倒数 height
原文地址:http://www.cnblogs.com/kimsimple/p/6390996.html