标签:begin tab min count output names nec width ram
| Time Limit: 3000MS | Memory Limit: 30000K | |
| Total Submissions: 1020 | Accepted: 407 |
Description
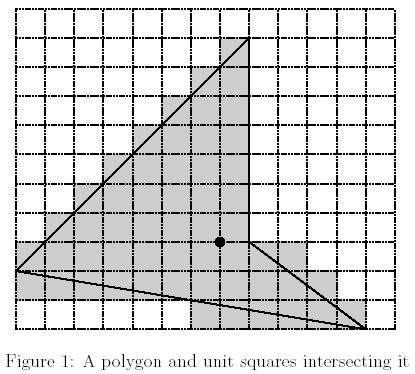
Input
Output
Sample Input
4 5 -3 1 0 1 7 -7 -1 3 5 5 18 5 5 10 3 -5 -5 -5 -10 -18 -10 5 0 0 20 2 11 1 21 2 2 0 0
Sample Output
55 41 41 23
Source
数学问题 几何 扫描线
看到题面心惊胆战,看到数据范围发现就是个暴力扫描线。
维护一条扫描线从x轴最左边往最右边移动,对于每一列记录原图形上线段和扫描线的交点纵坐标。
将交点坐标用floor和ceil取整,就可以愉快地做线段覆盖了。
注意计算交点时候,要先乘后除。先除后乘会被卡精度。
日常犯蠢WA一串,身败名裂。
1 #include<iostream> 2 #include<algorithm> 3 #include<cstring> 4 #include<cstdio> 5 #include<cmath> 6 using namespace std; 7 const int mxn=405; 8 int read(){ 9 int x=0,f=1;char ch=getchar(); 10 while(ch<‘0‘ || ch>‘9‘){if(ch==‘-‘)f=-1;ch=getchar();} 11 while(ch>=‘0‘ && ch<=‘9‘){x=x*10+ch-‘0‘;ch=getchar();} 12 return x*f; 13 } 14 struct point{ 15 double x,y; 16 point(){} 17 point(double _x,double _y):x(_x),y(_y){} 18 }p[mxn]; 19 struct Seg{point s,t;}L[mxn]; 20 double F(const point &a,const point &b,double x){ 21 return a.y+(b.y-a.y)*(x-a.x)/(b.x-a.x); 22 } 23 struct Line{ 24 double L,R; 25 bool operator < (const Line &b)const{ 26 return (L==b.L && R<b.R) || (L<b.L); 27 } 28 }s[mxn<<1]; 29 int sct=0; 30 int ans=0; 31 void solve(){ 32 sort(s+1,s+sct+1); 33 int last=-1e10; 34 for(int i=1;i<sct;i+=2){ 35 int x=floor(min(min(s[i].L,s[i+1].L),min(s[i].R,s[i+1].R))); 36 int y=ceil(max(max(s[i].L,s[i+1].L),max(s[i].R,s[i+1].R))); 37 if(x>=last)ans+=y-x; 38 else if(y>last)ans+=y-last; 39 last=y; 40 } 41 return; 42 } 43 int n; 44 int main(){ 45 int i,j; 46 while(scanf("%d",&n)!=EOF && n){ 47 ans=0; 48 int sx=1e8,mx=-1e8; 49 for(i=1;i<=n;i++){ 50 p[i].x=read();p[i].y=read(); 51 sx=min(sx,(int)p[i].x);mx=max(mx,(int)p[i].x); 52 } 53 p[n+1]=p[1]; 54 for(i=1;i<=n;i++){//segment 55 if(p[i].x<p[i+1].x){L[i].s=p[i];L[i].t=p[i+1];} 56 else{L[i].s=p[i+1];L[i].t=p[i];} 57 } 58 for(i=sx;i<mx;i++){ 59 sct=0; 60 for(j=1;j<=n;j++){ 61 if(L[j].s.x<=i && L[j].t.x>=i+1){ 62 ++sct; 63 s[sct].L=F(L[j].s,L[j].t,i); 64 s[sct].R=F(L[j].s,L[j].t,i+1); 65 if(s[sct].L>s[sct].R)swap(s[sct].L,s[sct].R); 66 } 67 } 68 solve(); 69 } 70 printf("%d\n",ans); 71 } 72 return 0; 73 }
标签:begin tab min count output names nec width ram
原文地址:http://www.cnblogs.com/SilverNebula/p/6963351.html