标签:bsp include 不同 int() for 没有 target nbsp alt
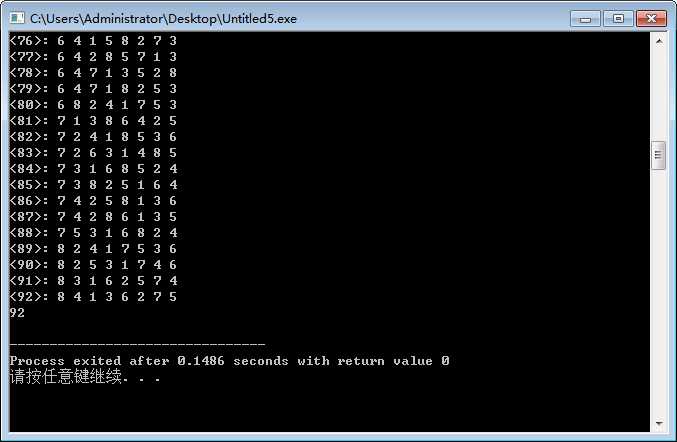
皇后问题,是一个古老而著名的问题,是回溯算法的典型案例。该问题是国际西洋棋棋手马克斯·贝瑟尔于1848年提出:在8X8格的国际象棋上摆放八个皇后,使其不能互相攻击,即任意两个皇后都不能处于同一行、同一列或同一斜线上,问有多少种摆法。 高斯认为有76种方案。1854年在柏林的象棋杂志上不同的作者发表了40种不同的解,后来有人用图论的方法解出92种结果。
默认从每行开始摆,判断竖和两斜
八皇后问题:
这题的标记数组有三个
原数组数组没有
结果数组有
1 /* 2 默认从每行开始摆,判断竖和两斜 3 八皇后问题: 4 这题的标记数组有三个 5 原数组数组没有 6 结果数组有 7 */ 8 #include <iostream> 9 using namespace std; 10 int b1[100],b2[100],b3[100]; 11 //b1是竖 b2是正斜 b3是反斜 12 int ans[100]; 13 int total=0; 14 15 void print(){ 16 total++; 17 cout<<"<"<<total<<">"<<": "; 18 for(int i=1;i<=8;i++){ 19 cout<<ans[i]<<" "; 20 } 21 cout<<endl; 22 } 23 24 void search(int t){//t是轮数,也是行数 25 if(t==9) print(); 26 else 27 for(int i=1;i<=8;i++){ 28 if(b1[i]==0&&b2[t-i+16]==0&&b3[t+i]==0){ 29 ans[t]=i,b1[i]=1,b2[t-i+16]=1,b3[t+i]=1; 30 search(t+1); 31 b1[i]=0,b2[t-i+16]=0,b3[t+i]=0; 32 } 33 } 34 } 35 int main(){ 36 search(1); 37 cout<<total<<endl; 38 return 0; 39 }
标签:bsp include 不同 int() for 没有 target nbsp alt
原文地址:http://www.cnblogs.com/Renyi-Fan/p/7119283.html