标签:insert ril ... 顺序 [1] images alt class cpp
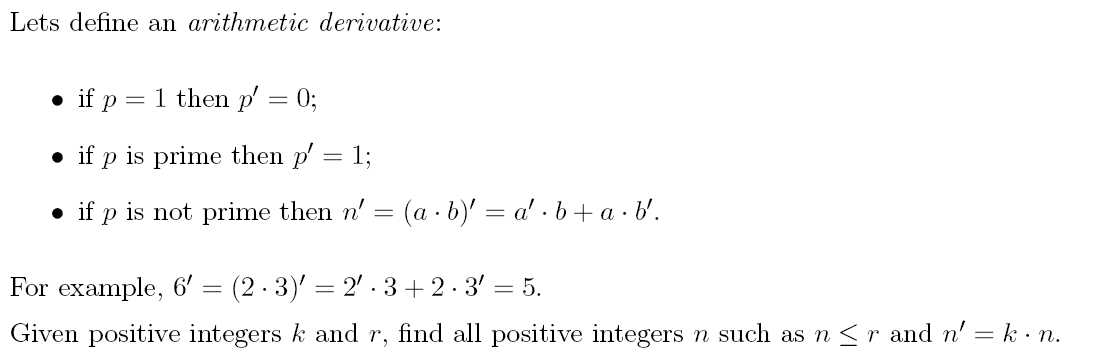
假设一个数有n个质因子a1,a2,..,an,那么n‘=Σ(a1*a2*...*an)/ai。
打个表出来,发现一个数x,如果x‘=Kx,那么x一定由K个“基础因子”组成。
这些基础因子是2^2,3^3,5^5,7^7,11^11,13^13。只有6个,K不超过30,于是可以dfs。
要注意搜索顺序(每次枚举的时候,都从大于等于前项的开始搜)和可行性剪枝(如果超过r则剪枝,虽说有可能爆long long,但其实整除就可以判,而且没有精度误差)。
#include<cstdio>
//#include<set>
#include<algorithm>
#include<cmath>
using namespace std;
//const long double EPS=0.0000000001;
typedef long long ll;
//set<ll>S;
ll base[11],path[1000010];
int pr[11];
int K,e;
ll r;
void dfs(int cur,int pre,ll now){
if(cur==K){
// if(S.find(now)==S.end()){
path[++e]=now;
// S.insert(now);
// }
return;
}
for(int i=pre;i<=6;++i){
if(base[i]>r/now){
break;
}
dfs(cur+1,i,now*base[i]);
}
}
int main(){
// freopen("a.in","r",stdin);
// freopen("a1.out","w",stdout);
pr[1]=2; pr[2]=3; pr[3]=5; pr[4]=7; pr[5]=11; pr[6]=13;
for(int i=1;i<=6;++i){
base[i]=1;
for(int j=1;j<=pr[i];++j){
base[i]*=(ll)pr[i];
}
}
while(scanf("%d%lld",&K,&r)!=EOF){
e=0;
// S.clear();
dfs(0,1,1ll);
printf("%d\n",e);
sort(path+1,path+e+1);
if(e){
for(int i=1;i<e;++i){
printf("%lld ",path[i]);
}
printf("%lld\n",path[e]);
}
}
return 0;
}
标签:insert ril ... 顺序 [1] images alt class cpp
原文地址:http://www.cnblogs.com/autsky-jadek/p/7286940.html