标签:blog http os io for 2014 div sp log
这题确实是好。
其实是求x1*a1+x2*a2+....M*xn+1=1有解的条件。很明显,就是(a1,a2,...M)=1了。然后,可以想象,直接求有多少种,很难,所以,求出选择哪些数一起会不与M互质。。。好吧,思路就到这里了。。。T_T
经过人提示,若(a1,a2,,,,an)与M不互质,则最大公约数中必定包含M中的质数。啊,愰然大悟,这不是显而易见的吗?为什么我想不到?
所以,先求出M包含哪些质数,那么,选出其中一些包含该质数的数组成数列不就好了?这很容易就能想到容斥原理了,因为选出一些数,使它具有性质P1,又选出另一些集合使它具有P2,P3....,最终求至少包含一个性质的集合。
那么,能被1~M中能被P1整除的个数为【M/p1】,以此类推。。。
由容斥原理公式
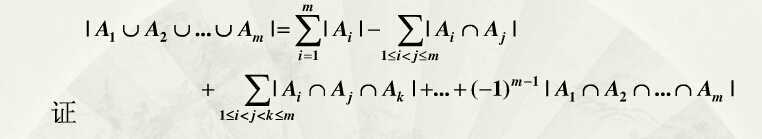
如此,从M^N中减去就可以了。
#include <iostream>
#include <cstdio>
#include <algorithm>
#define LL __int64
using namespace std;
LL prime[50],l;
LL n,m;
LL Power(LL m,LL n){
LL ret=1;
for(int i=1;i<=n;i++){
ret=ret*m;
}
return ret;
}
void wprime(LL m){
if(m%2==0){
prime[l++]=2;
while(m%2==0)
m/=2;
}
for(LL i=3;i*i<=m;i+=2)
if(m%i==0){
prime[l++]=i;
while(m%i==0)
m/=i;
}
if(m>1)
prime[l++]=m;
}
void Nest(LL p, LL re, LL c,LL &res){
if(c==0){
// cout<<re<<endl;
res+=Power(m/re,n);
return ;
}
else{
for(LL i=p;i<l;i++){
Nest(i+1,re*prime[i],c-1,res);
}
}
}
LL work(LL c){
LL res=0;
for(LL i=0;i<l;i++){
Nest(i+1,prime[i],c-1,res);
}
return res;
}
int main(){
while(scanf("%I64d%I64d",&n,&m)!=EOF){
l=0;
LL al=Power(m,n);
wprime(m);
LL c=1;
for(LL i=1;i<=l;i++){
c*=-1;
LL res=work(i);
al+=(c*res);
}
printf("%I64d\n",al);
}
return 0;
}
标签:blog http os io for 2014 div sp log
原文地址:http://www.cnblogs.com/jie-dcai/p/3956282.html