标签:弗洛伊德 atl cti logs size 路径 amp span .com
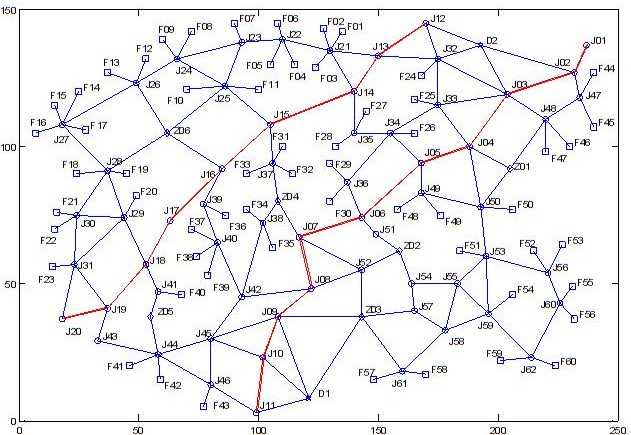
已知图中所有节点的X、Y坐标。
J01-J62:1-62;
F01-F60:63-122;
Z01-Z06:123-128;
D01-D02:129-130.
1 function [ optimal,path,maxnum ] = Floyd( distance,liantong,num,p,q ) 2 %Author:ljy 3 %Date:20170919 4 %弗洛伊德算法求最优路径和记录下最优路径中的节点信息 5 %distance为节点间的连通距离。通过已知的节点的X、Y坐标和连通矩阵计算而得。其中Inf为不连通节点间的距离。连通节点间的距离为正数(非Inf),连通情况通过连通(liantong)矩阵判断所得。 6 %num为所有节点个数 7 %p和q为不考虑路径的两端节点编号。例如p=[129,129];q=[9,10];表示129到9、129到10的路不连通。 8 %optimal为最优路径的距离信息 9 %path为最优路径的路径信息 10 %maxnum:最长的最优路径的节点总数 11 %liantong:节点间的连通情况,0为不连通,1为连通。
%path:行和列都为节点编号。path(i,j)为i到j的节点编号。 12 maxnum = 2; 13 14 15 %将distance变为邻接矩阵 16 for i = 1:num 17 for j = 1:num 18 if distance(i,j) == 0 & i ~= j 19 distance(i,j) = Inf; 20 end 21 end 22 end 23 24 %将p与q之间的路径的权重赋值为Inf,即不考虑p与q之间这条路径的最优路径。p、q为0表示没有不考虑的路径 25 for i = 1:size(p) 26 for j = 1:size(q) 27 if p(i) > 0 & q(j) > 0 28 distance(p(i),q(j)) = Inf; 29 distance(q(j),p(i)) = Inf; 30 liantong(p(i),q(j)) = 0; 31 liantong(q(j),p(i)) = 0; 32 end 33 end 34 end 35 36 %核心算法 37 for k = 1:num 38 for i = 1:num 39 for j = 1:num 40 r = 2;%最优路径所包含的节点个数 41 if distance(i,j) > distance(i,k) + distance(k,j) 42 distance(i,j) = distance(i,k) + distance(k,j); 43 %存储最优路径中的节点 44 p = i; 45 if liantong(p,j) ~= 0 46 while liantong(i,j) ~= liantong(p,k) & p ~= k 47 liantong(p,j) = liantong(p,k); 48 p = liantong(p,k); 49 r = r + 1; 50 end 51 else 52 liantong(p,j) = liantong(p,k); 53 r = r + 1; 54 end 55 end 56 if r > maxnum 57 maxnum = r; 58 end 59 end 60 end 61 end 62 63 %返回数据 64 optimal = distance; 65 path = liantong; 66 end
标签:弗洛伊德 atl cti logs size 路径 amp span .com
原文地址:http://www.cnblogs.com/landiljy/p/7567167.html