标签:mil pid 分享 close cst ret print es2017 ges
这是一道模板题
给定n,p求1~n中所有整数在模p意义下的乘法逆元。
输入格式:
一行n,p
输出格式:
n行,第i行表示i在模p意义下的逆元。
10 13
1 7 9 10 8 11 2 5 3 4
1≤n≤3×10?6??,n<p<20000528
输入保证 p 为质数。
我们有三种办法求逆元
由欧拉定理可知
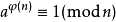
当gcd(a,n)==1 时 我们有 Aφ(n-1)≡ 1(mod n) ;
所以 我们有 A*Aφ(n-2) ≡ 1(mod n)
所以Aφ(n-2) 就是A关于mod n的逆元

1 /* 2 p为素数时 可用费马小定理 3 longlong*longlong 要慢一点 66分 4 */ 5 #include <cctype> 6 #include <cstdio> 7 8 typedef long long LL; 9 10 int n,p; 11 12 inline LL quick_pow(LL a,int k) { 13 LL ret=1; 14 while(k) { 15 if(k&1) ret=(ret*a)%p; 16 k>>=1; 17 a=(a*a)%p; 18 } 19 return ret; 20 } 21 22 int hh() { 23 scanf("%d%d",&n,&p); 24 printf("1\n"); 25 for(int i=2;i<=n;++i) { 26 LL t=quick_pow(i,p-2); 27 printf("%d\n",(t+p)%p); 28 } 29 return 0; 30 } 31 32 int sb=hh(); 33 int main(int argc,char**argv) {;}
还有我们可以用exgcd来求逆元
我们知道 若ax≡1(mod p) 这我们可以写成 ax=py+1;
移项则有 ax-by=1 这明显就是扩展欧几里得
当 ax+by=gcd(a,b) gcd(a,b) == gcd(b,a%b)
我们得到 bx1+(a-a/b)y1=gcd(b,a%b);
则 ax+by=bx1+(a-(a/b)*b)y1 //这里 / 代表整除
ax+by=bx1+ay1-b*(a/b)y1
ax+by=ay1+b(x1-(a/b)*y1)
我们得到 x=y1
y=x1-(a/b)*y1;
x 即为我们所求的逆元
由于 x 可能为负数 要(x+p)%p

1 /* 2 EXgcd 求逆元 3 比费马小定理要快一点 83分 4 */ 5 #include <cstdio> 6 #include <cctype> 7 8 int n,p; 9 10 inline int exgcd(int a,int b,int&x,int&y) { 11 if(!b) { 12 x=1;y=0; 13 return a; 14 } 15 int p=exgcd(b,a%b,x,y); 16 int t=x; 17 x=y; 18 y=t-(a/b)*y; 19 return p; 20 } 21 22 int hh() { 23 scanf("%d%d",&n,&p); 24 printf("1\n"); 25 int x,y; 26 for(int i=2;i<=n;++i) { 27 exgcd(i,p,x,y); 28 printf("%d\n",(x+p)%p); 29 } 30 return 0; 31 } 32 33 int sb=hh(); 34 int main(int argc,char**argv) {;}
但是对于 这个题来讲 复杂度还是不够
我们还有线性求逆元的方法
来看带余除法 式子 p=k*i+r
我们可以写成 k*i+r≡0(mod p)
式子两边同乘 i-1*r-1 (i-1,r-1皆为模p意义下的逆元)
所以我们有 k*r-1+i-1≡0(mod p)
i-1≡-k*r-1(mod p)
i-1≡-(p/i)*(p%i)-1(mod p)
这样我们就线性求得了逆元

1 #include <cctype> 2 #include <cstdio> 3 4 typedef long long LL; 5 const int MAXN=3000010; 6 7 int n,p; 8 9 LL inv[MAXN]; 10 11 int hh() { 12 scanf("%d%d",&n,&p); 13 printf("1\n"); 14 inv[1]=1; 15 for(int i=2;i<=n;++i) { 16 inv[i]=(LL)(p-p/i)*inv[p%i]%p; 17 printf("%d\n",inv[i]); 18 } 19 return 0; 20 } 21 22 int sb=hh(); 23 int main(int argc,char**argv) {;}
标签:mil pid 分享 close cst ret print es2017 ges
原文地址:http://www.cnblogs.com/whistle13326/p/7576986.html