标签:ges alt char def getchar opened http cst fine
期望得分:100+0+30=130
实际得分:100+36.5+0=136.5
T3 一个变量写混了,丢了30。。

模拟栈

#include<cstdio> #include<cstring> using namespace std; #define N 10001 char s[N]; int st[N],top; int main() { freopen("a.in","r",stdin); freopen("a.out","w",stdout); scanf("%s",s); int len=strlen(s); for(int i=0;i<len;i++) if(s[i]==‘(‘) st[++top]=1; else if(s[i]==‘[‘) st[++top]=2; else if(s[i]==‘{‘) st[++top]=3; else if(s[i]==‘)‘) { if(st[top]==1) top--; else { printf("Wrong");return 0; } } else if(s[i]==‘]‘) { if(st[top]==2) top--; else { printf("Wrong"); return 0; } } else { if(st[top]==3) top--; else { printf("Wrong"); return 0; } } if(top) printf("Wrong"); else printf("OK"); return 0; }
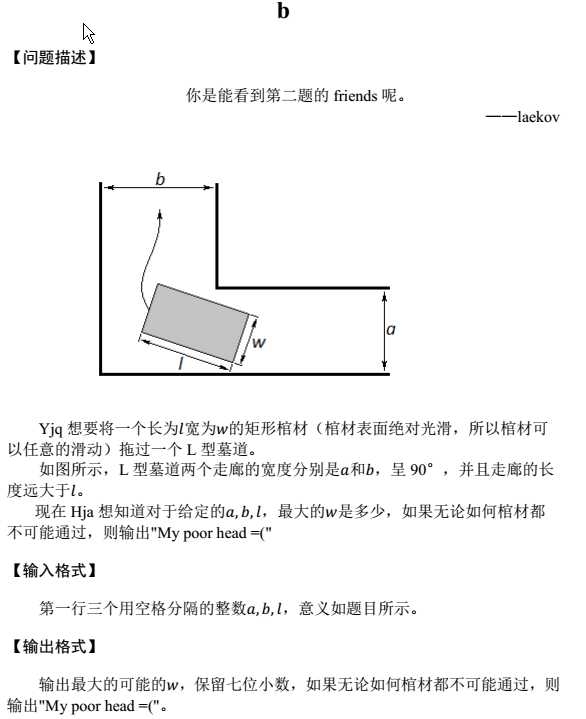

设直线解析式为 y=(-n/m)* x+n
整理,得:- n * x + m * y + n * m = 0
点(b,a)到直线的距离为:| - b * n - a * m + n * m | / L
(L : 根号下(n^2 + m^2)=L)
最优解就是 - b * n - a * m + n * m 的最大值
然后不知道为啥求了个
b * n + a * m - n *m 的最小值
单峰函数求最小值,三分法每次去掉大的一部分
式子最小值<0 时无解

#include<algorithm> #include<cstdio> #include<cmath> using namespace std; const double eps=1e-9; int a,b,l; double f(double n) { double m=sqrt(1.0*l*l-n*n); if(n*m>a*n+b*m) return -1; return (b*m+a*n-n*m)/l; } int main() { freopen("b.in","r",stdin); freopen("b.out","w",stdout); scanf("%d%d%d",&a,&b,&l); if(a>=l && b>=l) { printf("%d.0000000",l); return 0; } if(a>=l) { printf("%d.0000000",b); return 0; } if(b>=l) { printf("%d.0000000",a); return 0; } double L=0,R=l,ans=1e9,mid1,mid2,t1,t2; int T=100; while(T--) { mid1=(R-L)/3+L; mid2=L+R-mid1; t1=f(mid1); t2=f(mid2); if(t1<0 || t2<0) { printf("My poor head =("); return 0; } if(t1<t2) ans=min(ans,t1),R=mid2; else ans=min(ans,t2),L=mid1; } printf("%.7lf",ans); }
一个求最大值不知道为啥WA了的代码

#include<algorithm> #include<cstdio> #include<cmath> using namespace std; const double eps=1e-9; int a,b,l; double f(double n) { double m=sqrt(1.0*l*l-n*n); return (- b * n - a * m + n * m)/l; } int main() { // freopen("b.in","r",stdin); //freopen("b.out","w",stdout); scanf("%d%d%d",&a,&b,&l); if(a>=l && b>=l) { printf("%d.0000000",l); return 0; } if(a>=l) { printf("%d.0000000",b); return 0; } if(b>=l) { printf("%d.0000000",a); return 0; } double L=0,R=l,ans=-1,mid1,mid2,t1,t2; int T=100; while(T--) { mid1=(R-L)/3+L; mid2=L+R-mid1; t1=f(mid1); t2=f(mid2); if(t1<t2) ans=max(ans,t2),L=mid1; else ans=max(ans,t1),R=mid2; } if(ans<eps) printf("My poor head =("); else printf("%.7lf",ans); }

递归回溯时贪心
如果当前点的分支个数>=2,那么断掉它与父节点的边,子节点中只留两个最优
所以 ans+=分支个数-2
但是如果是递归的根节点,就是ans+=分支个数-1
因为根节点可以与两个分支相连
如果当前只有一个分支,那就不用断,仍然是它的父节点的一个分支
最终的答案就是ans*2+1
*2是因为断掉一个,相应的就要添加一条
+1是最后要形成一个环

#include<cstdio> #include<iostream> #define N 100001 using namespace std; int front[N],nxt[N<<1],to[N<<1],tot; int ans; void read(int &x) { x=0; char c=getchar(); while(!isdigit(c)) c=getchar(); while(isdigit(c)) { x=x*10+c-‘0‘; c=getchar(); } } void add(int u,int v) { to[++tot]=v; nxt[tot]=front[u]; front[u]=tot; to[++tot]=u; nxt[tot]=front[v]; front[v]=tot; } int dfs(int x,int f) { int sum=0; for(int i=front[x];i;i=nxt[i]) if(to[i]!=f) sum+=dfs(to[i],x); if(sum>=2) { if(x==1) ans+=sum-2; else ans+=sum-1; return 0; } return 1; } int main() { freopen("c.in","r",stdin); freopen("c.out","w",stdout); int n,u,v; read(n); for(int i=1;i<n;i++) read(u),read(v),add(u,v); dfs(1,0); printf("%d",ans*2+1); }
标签:ges alt char def getchar opened http cst fine
原文地址:http://www.cnblogs.com/TheRoadToTheGold/p/7642212.html