有一个a*b的整数组成的矩阵,现请你从中找出一个n*n的正方形区域,使得该区域所有数中
的最大值和最小值的差最小。
标签:ble namespace 蓝色 end while line memory lin stream
有一个a*b的整数组成的矩阵,现请你从中找出一个n*n的正方形区域,使得该区域所有数中
的最大值和最小值的差最小。
第一行为3个整数,分别表示a,b,n的值第二行至第a+1行每行为b个非负整数,表示矩阵中相应
位置上的数。每行相邻两数之间用一空格分隔。
100%的数据2<=a,b<=1000,n<=a,n<=b,n<=1000
仅一个整数,为a*b矩阵中所有“n*n正方形区域中的最大整数和最小整数的差值”的最小值。
在一个序列上用单调队列会吧?
那么我们在矩阵的每一行用单调队列,求出每行以i为起点,向右n个数的最小最大值
然后我们矩阵的每一个数向右的最小最大值知道了吧?然后我们在每一列上做单调队列,
求我们之前求出的每个数往右的最小最大的值的最小最大值,然后知道每个矩阵的最小
最大值...好绕....
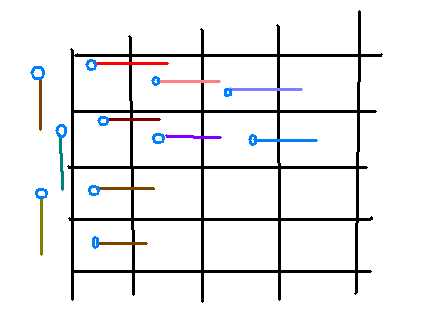
如图,假设现在n=2,我们要找2*2的正方形。蓝色的圆圈表示起点,不同颜色的横线表示以蓝色
的圆圈开头,我们求出了每个横线覆盖范围的最小最大值。然后横向的线段表示求行的最小最大
值。求完之后再对列做单调队列,在所有每行的最小值中当中选出最小的...每行最大的值中选出最
大值。太...太强了..orz...昨天晚上理解了很久的题解...今天晚上终于做完了....
╭︿︿︿╮
{/ o o /}
( (oo) ) =u= mua!...
︶ ︶ ︶
明明只找了20分钟的BUG,我们怎么感觉像一个小时....
#include<iostream> #include<cstdio> #include<cstring> #define maxn 1007 #define inf 1000000000 using namespace std; int ans,a,b,n,map[maxn][maxn]; int qmn[maxn],qmx[maxn]; int h_mn,h_mx,t_mn,t_mx; int hmn[maxn][maxn],hmx[maxn][maxn],ansmn[maxn][maxn],ansmx[maxn][maxn]; int main(){ scanf("%d%d%d",&a,&b,&n); for(int i=1;i<=a;i++) for(int j=1;j<=b;j++) scanf("%d",&map[i][j]); for(int i=1;i<=a;i++){ h_mn=h_mx=1; t_mn=t_mx=0; for(int j=1;j<=b;j++){ while(h_mn<=t_mn&&map[i][qmn[t_mn]]>map[i][j])t_mn--; qmn[++t_mn]=j; while(h_mx<=t_mx&&map[i][qmx[t_mx]]<map[i][j])t_mx--; qmx[++t_mx]=j; while(qmn[t_mn]-qmn[h_mn]>=n&&h_mn<=t_mn)h_mn++; while(qmx[t_mx]-qmx[h_mx]>=n&&h_mx<=t_mx)h_mx++; int t=max(1,j-n+1); hmn[i][t]=map[i][qmn[h_mn]]; hmx[i][t]=map[i][qmx[h_mx]]; } } for(int j=1;j<=b;j++){ h_mn=h_mx=1;t_mn=t_mx=0; for(int i=1;i<=a;i++){ while(h_mn<=t_mn&&hmn[qmn[t_mn]][j]>hmn[i][j])t_mn--; qmn[++t_mn]=i; while(h_mx<=t_mx&&hmx[qmx[t_mx]][j]<hmx[i][j])t_mx--; qmx[++t_mx]=i; while(qmn[t_mn]-qmn[h_mn]>=n&&h_mn<=t_mn)h_mn++; while(qmx[t_mx]-qmx[h_mx]>=n&&h_mx<=t_mx)h_mx++; int t=max(1,i-n+1); ansmn[t][j]=hmn[qmn[h_mn]][j]; ansmx[t][j]=hmx[qmx[h_mx]][j]; } } ans=inf; for(int i=1;i<=a-n+1;i++) for(int j=1;j<=b-n+1;j++) ans=min(ans,ansmx[i][j]-ansmn[i][j]); cout<<ans<<endl; return 0; }
标签:ble namespace 蓝色 end while line memory lin stream
原文地址:http://www.cnblogs.com/zzyh/p/7647068.html