greedy1521

标签：style   blog   http   io   os   使用   ar   for   数据   

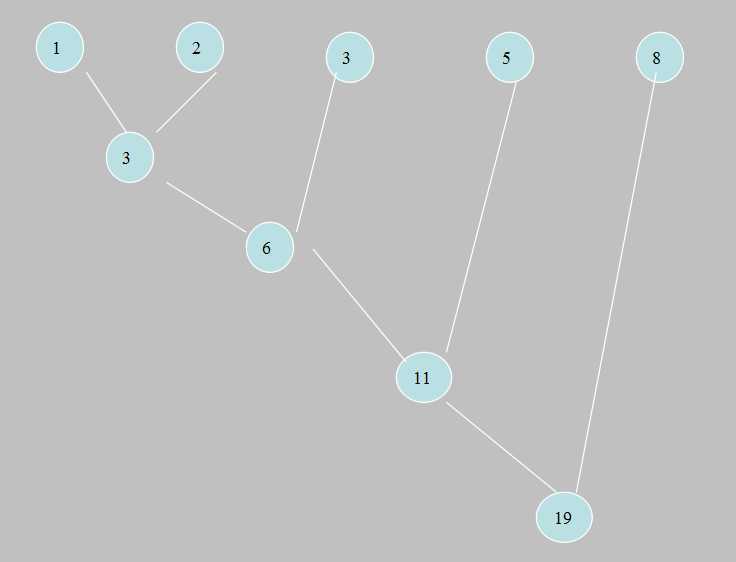
如上图中的2，用4位二进制（总共4层）来表示，同时总共出现2次。即共需要位数为2*4，实际上反应在哈佛曼树中，即为在每层都加上2，总共加了4次（4即为2的层数，从最底层一直到19,19不算），因此将除最顶层19除外，将树种所有的数总加起来，即为每个数总共需要的位数。

关于priority_queue<int,vecto<int>r,greater<int> >, 第一个参数为队列中的数据类型,第二个参数为容器类型,第三个参数为比较方法,常用的有greater,less等,也可以根据实际情况自己去写.当参数为greater<int>时,则可以保证每次插入后队头的数都是最小的数.

              Huffman树的构造就不赘述了,使用优先队列每次选择队头的两个数并将其出列,相加后将结果放入队列中,直到队列为空为止

//这题考查哈弗曼编码，但其实没必要建树得出编码，只需要统计哈弗曼编码后的总码长即可

#include <iostream>

#include <queue>

using namespace std;

class mycomparison

{

public:

  bool operator() (const int& lhs, const int&rhs) const

  {

    return (lhs > rhs);//使得从小到大排序，队列头为最小元素，优选权队列默认队列头最大

  }

};

int main(){

char s[500];

int count[200] = {0};//count[i]记录ASCII码为i的字符的个数

int i, sum, len;

priority_queue<int, vector<int>, mycomparison> pq;

while(cin>>s){

if(!strcmp(s,"END")){

break;

}

len = strlen(s);

for(i = 0; i < len; i++){

count[s[i]]++;

} 

sum = 0;

for(i = 0;i < 200; i++){

if(count[i]){

pq.push(count[i]);

count[i] = 0;

}

}

while(pq.size() > 1){

int a = pq.top(); pq.pop();

int b = pq.top(); pq.pop();

sum += a + b;//其实就是把越小的频率反复多加几次，越大的频率少加几次

pq.push(a + b);

}

if(!sum){

sum = len;//此时pq中只有一个元素

}

while(!pq.empty()){

pq.pop();

}

printf("%d %d %.1f\n",8*len,sum,double(double(8*len)/sum));//注意精度设置

}

return 0;

}

greedy1521

标签：style   blog   http   io   os   使用   ar   for   数据   

原文地址：http://www.cnblogs.com/notlate/p/3973180.html