| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 131191 | Accepted: 63828 |
Description
How far can you make a stack of cards overhang a table? If you have one card, you can create a maximum overhang of half a card length. (We‘re assuming that the cards must be perpendicular to the table.) With two cards you can make the top card overhang the bottom one by half a card length, and the bottom one overhang the table by a third of a card length, for a total maximum overhang of 1/2 + 1/3 = 5/6 card lengths. In general you can make n cards overhang by 1/2 + 1/3 + 1/4 + ... + 1/(n + 1) card lengths, where the top card overhangs the second by 1/2, the second overhangs tha third by 1/3, the third overhangs the fourth by 1/4, etc., and the bottom card overhangs the table by 1/(n + 1). This is illustrated in the figure below.
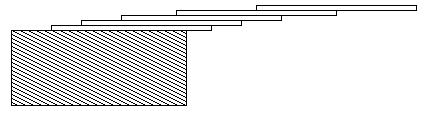
Input
Output
Sample Input
1.00 3.71 0.04 5.19 0.00
Sample Output
3 card(s) 61 card(s) 1 card(s) 273 card(s)
#include<stdio.h> #include<iostream > using namespace std; int main() { double len=0.0,overlen=0.0; int c; while(scanf("%lf",&len) == 1) { if(len == 0.0) return 0; else{ overlen = 0; for(c=1;c;c++) { overlen += 1.0/(c+1); if(overlen>=len) { break; } } cout<<c<<" card(s)"<<endl; } } return 0; }
以上代码为经过学习最终代码。。。自己的水平太差,打的代码有点乱
/*有问题*/
#include <iostream> #include <stdio.h> using namespace std; int main(void) { double len=0.00,templen=0.00; int c=0; while(scanf("%lf",&len)==1) { templen = 0.0; if(0.01<len<=0.50) c=1; else if(5.20>len>0.50) { for(c=0;len>templen;c++) templen+=1.0/(c+2); } else c=0; if(c != 0) cout<<c<<" card(s)"<<endl; else return 0; } return 0; }
出现问题:
warning: comparisons like ‘X<=Y<=Z‘ do not have their mathematical meaning [-Wparentheses]
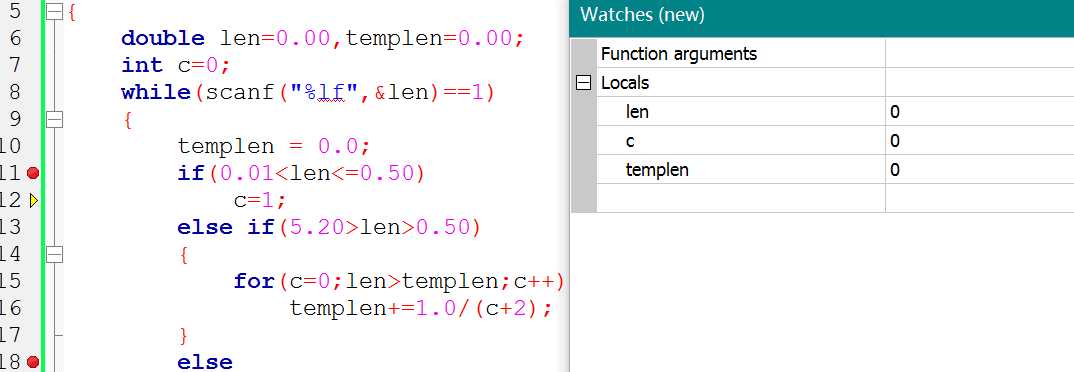
很不明白为什么为什么len=0.00会进入这层判断。。。经过研究
在比较float和double类型的时候,
因为float/double精度的问题
比如 1.000000001 可能和1.0000000000001相等
不应该直接使用 a > b 等类似的方式进行比较
而是采用 两个数做差取绝对值然后跟 你指定的精度进行比较
便可得出 两个double/float的大小
第一次的代码逻辑也有问题ying~
不改了……
