题意如标题所示。测试数据规模为100000。
首先从DP的角度考虑
状态：i（数组下标）
状态转移方程：
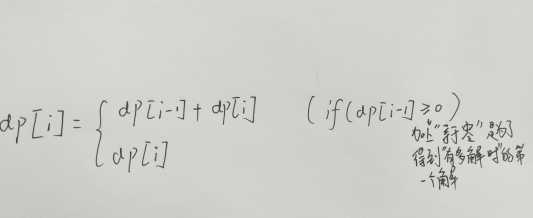注：加上“等于零”是为了得到有多解时，的第一个解。（原谅我的字 -_-）
初始边界状态极其值：dp[0]。
最大连续序列和：定义一个max_sum=dp[0]，从前到后，根据状态转移方程不断更新
最大连续序列的起点与终点（这个我觉的非常容易写错）：定义begin与end初始为零，当状态转移方程满足上半部分时，end=i；满足下半部分时，令temp=i，直到后面有dp[i]>max_sum时，begin=i；
下面展示了一种错误情况，高亮部分为错误部分（好像看不到高亮，我又加了注释），很容易修改,不再给出ac代码，一个可以测试出其错误的样例是：
2
9 100 5 -105 -1 -1 10 10 10 1000
7 0 6 -1 1 -6 7 -5
错误代码：
#include <iostream>
using namespace std;
const int maxn=100000+10;
int dp[maxn];
int main()
{
freopen("in.txt","r",stdin);
int c,n,begin,end;
int count=0;
scanf("%d",&c);
for(int i=0;i<c;i++){
scanf("%d",&n);
for(int l=0;l<n;l++){
scanf("%d",&dp[l]);
}
printf("Case %d:\n",++count);
begin=end=0;
int max_sum=dp[0];
if(n>1)
for(int l=1;l<n;l++){ //不能改为 l<=n
if( dp[l-1]>=0 ){
dp[l]+=dp[l-1];
}
else{
if(dp[l]>max_sum) //错误部分
begin=l; //错误部分
}
if(max_sum<dp[l]){
max_sum=dp[l];
end=l;
}
max_sum=max_sum>dp[l]?max_sum:dp[l];
}
printf("%d %d %d\n",max_sum,begin+1,end+1);
if(i!=(c-1))
cout<<endl;
}
return 0;
}
