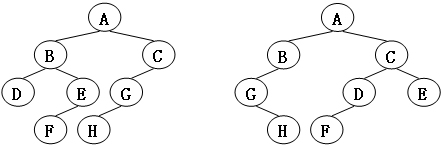
给定两棵树T1和T2。如果T1可以通过若干次左右孩子互换就变成T2,则我们称两棵树是“同构”的。例如图1给出的两棵树就是同构的,因为我们把其中一棵树的结点A、B、G的左右孩子互换后,就得到另外一棵树。而图2就不是同构的。
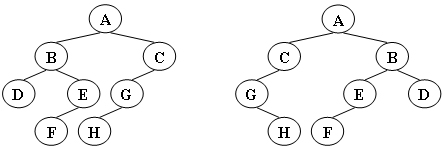
图1
图2
输入格式:
输入给出2棵二叉树树的信息。对于每棵树,首先在一行中给出一个非负整数N (≤10),即该树的结点数(此时假设结点从0到N?1编号);随后N行,第i行对应编号第i个结点,给出该结点中存储的1个英文大写字母、其左孩子结点的编号、右孩子结点的编号。如果孩子结点为空,则在相应位置上给出“-”。给出的数据间用一个空格分隔。注意:题目保证每个结点中存储的字母是不同的。
输出格式:
如果两棵树是同构的,输出“Yes”,否则输出“No”。
输入样例1(对应图1):
8
A 1 2
B 3 4
C 5 -
D - -
E 6 -
G 7 -
F - -
H - -
8
G - 4
B 7 6
F - -
A 5 1
H - -
C 0 -
D - -
E 2 -
输出样例1:
Yes
输入样例2(对应图2):
8
B 5 7
F - -
A 0 3
C 6 -
H - -
D - -
G 4 -
E 1 -
8
D 6 -
B 5 -
E - -
H - -
C 0 2
G - 3
F - -
A 1 4
输出样例2:
No
我的答案1 #include <stdio.h> 2 #include <stdlib.h> 3 #include <unistd.h> 4 5 #define MaxTree 10 6 #define ElementType char 7 #define Tree int 8 #define Null -1 9 10 struct TreeNode { 11 ElementType Element; 12 Tree Left; 13 Tree Right; 14 } T1[MaxTree], T2[MaxTree]; 15 int N, check[MaxTree]; 16 17 Tree BuildTree(struct TreeNode T[]); 18 int Isomorphic(Tree R1, Tree R2); 19 20 int main() 21 { 22 Tree R1, R2; 23 R1 = BuildTree(T1); 24 // printf("R1 build done\n"); 25 R2 = BuildTree(T2); 26 // printf("R3 build done\n"); 27 if(Isomorphic(R1, R2)) 28 printf("Yes\n"); 29 else 30 printf("No\n"); 31 return 0; 32 } 33 34 Tree BuildTree(struct TreeNode T[]) 35 { 36 char cl, cr; 37 int i; 38 Tree Root = Null; 39 scanf("%d\n", &N); 40 if(N) { 41 for(i=0;i<N;i++) 42 check[i] = 0; 43 for(i=0;i<N;i++) { 44 scanf("%c %c %c\n", &T[i].Element, &cl, &cr); 45 if(cl != ‘-‘) { 46 T[i].Left = cl - ‘0‘; 47 check[T[i].Left] = 1; 48 } else 49 T[i].Left = Null; 50 if(cr != ‘-‘) { 51 T[i].Right = cr - ‘0‘; 52 check[T[i].Right] = 1; 53 } else 54 T[i].Right = Null; 55 } 56 for(i=0;i<N;i++) 57 if(check[i] != 1) break; 58 Root = i; 59 } 60 return Root; 61 } 62 63 int Isomorphic(Tree R1,Tree R2) 64 { 65 if((R1==Null)&&(R2==Null)) /* both empty */ 66 return 1; 67 if(((R1==Null)&&(R2!=Null))||((R1!=Null)&&(R2==Null))) /* one of them is empty */ 68 return 0; 69 if(T1[R1].Element!=T2[R2].Element) /* roots are different */ 70 return 0; 71 if((T1[R1].Left==Null)&&(T2[R2].Left==Null)) /*bot have no left subtree */ 72 return Isomorphic(T1[R1].Right, T2[R2].Right); 73 if(((T1[R1].Left!=Null)&&(T2[R2].Left!=Null))&& 74 ((T1[T1[R1].Left].Element)==(T2[T2[R2].Left].Element))) 75 /* no need to swap the left and the right */ 76 return (Isomorphic(T1[R1].Left, T2[R2].Left)&& 77 Isomorphic(T1[R1].Right, T2[R2].Right)); 78 else /* need to swap the left and the right */ 79 return (Isomorphic(T1[R1].Left, T2[R2].Right) && 80 Isomorphic(T1[R1].Right, T2[R2].Left)); 81 }