标签:ima pac 分享 转移 pre 开始 printf problems time
题目链接:http://acm.csu.edu.cn/csuoj/problemset/problem?pid=1804
Input
Output对于每组数据,输出一个整数表示要求的值。Sample Input
3 3 1 1 1 1 1 1 1 2 1 3 2 3 2 2 1 0 0 2 1 2 1 2 2 1 500000000 0 0 500000000 1 2
Sample Output
4 4 250000014
题解:
首先,假如我们计算$\sum\limits_{i = 1}^n {\sum\limits_{j = 1}^n {\left( {count\left( {i,j} \right) \times a_i \times b_j } \right)} } $
这个的时候,固定一个点i,枚举j进行计算的话,就有:
$a_i \times \left[ {\sum\limits_{j = 1}^n {\left( {count\left( {i,j} \right) \times b_j } \right)} } \right]$
我们不妨设$dp\left[ i \right] = \sum\limits_{j = 1}^n {\left( {count\left( {i,j} \right) \times b_j } \right)} $
那么,最后的${\rm{ans}} = \sum\limits_{i = 1}^n {\left\{ {a_i \times \left[ {\sum\limits_{j = 1}^n {\left( {count\left( {i,j} \right) \times b_j } \right)} } \right]} \right\}} $
问题来了,状态转移方程是什么?
假设对于点i,它有K个子节点,就有:
$dp\left[ i \right] = \sum\limits_{k = 1}^K {\left( {b_k + dp\left[ k \right]} \right)} $
(根据题意无环图,则存在 Edge(i→k) 就一定不存在一条路径从k点到i点,所以计算dp[k]时就一定不会涉及到dp[i])
另外,本题如果不是有向无环图而是一棵树的话,很显然,直接从树根往下dfs计算每个节点i的dp[i]即可,
但是现在有向无环图,可能出现如下情况:
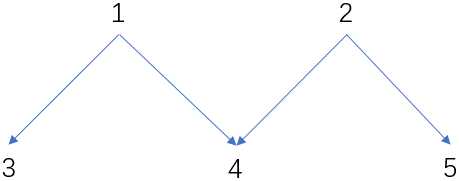
这样一来,如果主函数里单单dfs(1)或者单单dfs(2)都不能把整个图上所有节点的dp[i]都计算到,
因此要把所有in-degree[i]==0的节点i都dfs(i).
AC代码:
#include<cstdio> #include<cstring> #include<vector> using namespace std; typedef long long LL; const LL MOD=1e9+7; const int maxn=1e5+10; int n,m; int indegree[maxn]; LL a[maxn],b[maxn]; LL dp[maxn]; struct Edge{ int u,v; Edge(int u,int v){this->u=u,this->v=v;} }; vector<Edge> E; vector<int> G[maxn]; void init(int l,int r) { E.clear(); for(int i=l;i<=r;i++) G[i].clear(); } void addedge(int u,int v) { E.push_back(Edge(u,v)); G[u].push_back(E.size()-1); } LL dfs(int u) { if(dp[u]!=-1) return dp[u]; dp[u]=0; for(int i=0,_size=G[u].size();i<_size;i++) { Edge &e=E[G[u][i]]; int v=e.v; dp[u]=(dp[u]+b[v]+dfs(v))%MOD; } return dp[u]; } int main() { while(scanf("%d%d",&n,&m)!=EOF) { for(int i=1;i<=n;i++) scanf("%d%d",&a[i],&b[i]); init(1,n); //邻接表初始化 memset(indegree,0,sizeof(indegree)); for(int i=1,u,v;i<=m;i++) { scanf("%d%d",&u,&v); addedge(u,v); indegree[v]++; } memset(dp,-1,sizeof(dp)); for(int i=1;i<=n;i++) { if(indegree[i]==0) dfs(i); } LL ans=0; for(int i=1;i<=n;i++) ans = ( ans + (dp[i]*a[i]) % MOD ) % MOD; printf("%lld\n",ans); } }
标签:ima pac 分享 转移 pre 开始 printf problems time
原文地址:https://www.cnblogs.com/dilthey/p/8983122.html