标签:判断 条件 nbsp point img 技术分享 代码 估算 size
#对如下红色图形进行面积估算
x = np.linspace(0, 2, 1000)#在0~2之间产生1000个样本点x y = x **3 #求出对应的y值 plt.plot(x, y) plt.fill_between(x, y, where=(y >0), color=‘red‘, alpha=0.5)#画出y>0的面积进行填充 plt.show()
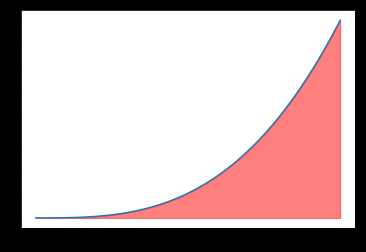
该红色区域在一个2×8的矩形方框里面。使用蒙特卡洛方法,随机在这个矩形里面产生大量随机点(数量为N),计算有多少点(数量为count)落在红色区域内(判断条件为y<x**3),count/N就是落在红色区域的点的比例,根据相应比例即可求出红色区域的面积。代码如下:
x = np.linspace(0, 2, 1000) y = x ** 3 plt.plot(x, y) plt.fill_between(x, y, where=(y >0), color=‘red‘, alpha=0.5)
N = 10000 #样本点的总量为10000,越大估算出来的结果越精确 points = [[xy[0] * 2, xy[1] * 8] for xy in np.random.rand(N, 2)]# np.random.rand(N,2)表示产生N行2列的样本点,即N个2维样本点
plt.scatter([x[0] for x in points], [x[1] for x in points], s=5, c=np.random.rand(N), alpha=0.5)
plt.show()

下面为估算有多少个点落在红色区域,并估算其面积。
count = 0 for xy in points: if xy[1] < xy[0] ** 3: count += 1 print((count / N) * (2 * 8))
结果:
利用积分计算可知该红色区域的精确面积为4,如果样本点设置为1000,估算结果为4.24,如果样本点设置为10000,估算结果为3.968,所以样本点越多,估算越精确。
标签:判断 条件 nbsp point img 技术分享 代码 估算 size
原文地址:https://www.cnblogs.com/gczr/p/9132659.html