标签:const com tis typename 小学 区间 台阶 bit type
题目大意:
给定序列\(\{a_i\}\),求一个严格递增序列\(\{b_i\}\),使得\(\sum \bigl |a_i-b_i\bigr|\)最小
正序:直接对应
逆序:取中位数(证明:“医院设置”)
最优解一定是分段
每一段台阶式上升
每一段选取中位数
沙漏型左偏树 合并区间 选取中位数
upd:貌似不需要沙漏型?
前置技能:小学奥数、可并堆
和上面类似,先不考虑严格上升,即先考虑非严格上升
序列一定是要分成若干段,每一段的\(b\)值相等,且后一段比前一段大,像台阶一样(如下图,是一个\(b(x)\)的伪函数)
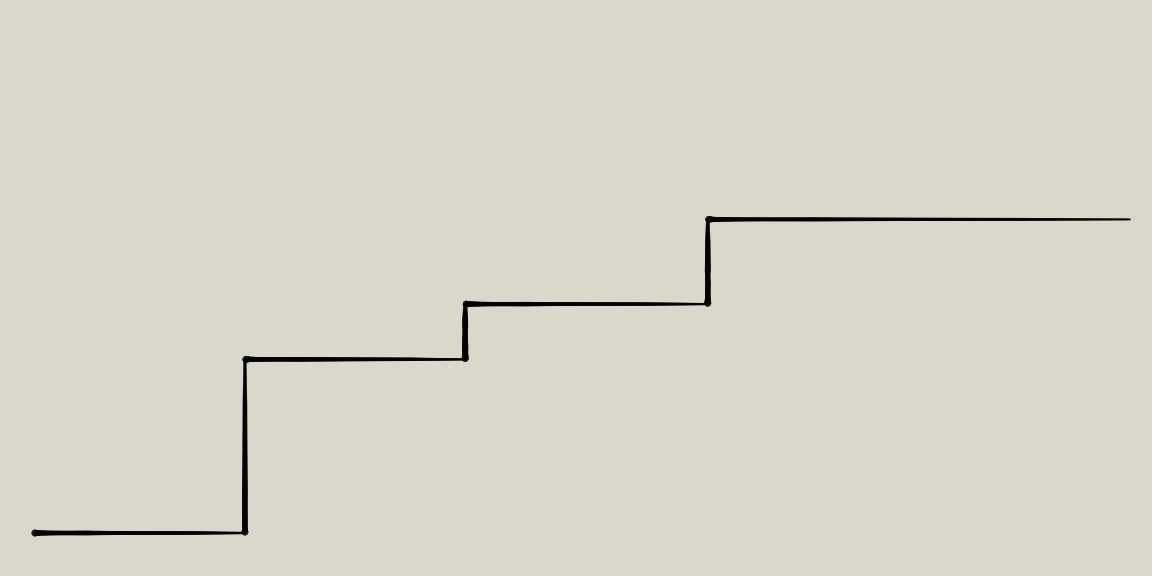
先令\(\forall i\in[1,n],a_i=b_i\),这样的答案为零,但却不合法,接下来考虑如何用最小代价使答案合法,考虑对于相邻两段数:
设当前前一段取最优值时的\(b\)统一为\(b_1\),后一段统一为\(b_2\),变换之后两者的统一\(b\)值分别变为\(b_1^{‘},b_2^{‘}\)
如果\(b_1\leq b_2\),则对于这两段来说是合法的,无需操作;
如果\(b_1>b_2\),则表示因为要求\(b_1\leq b_2\),而现在是\(b_1>b_2\),要求\(b_1^{‘}\leq b_2^{‘}\),考虑到两段的\(b\)变化得越少越好,即\(\bigl | b_1-b_1^{‘}\bigr |,\bigl | b_1-b_1^{‘}\bigr |\)取最小,则变换之后\(b_1^{‘}=b_2^{‘}\),我们再考虑\(b_1^{‘}(b_2^{‘})\)的取值,应为这两段数合在一起的中位数,证明见下方“附”,找中位数可以用线段树解决,也可以用堆解决(堆解法见TJOI2010中位数),考虑到两段需要合并,线段树需要线段树合并,而堆只需要可并堆即可
如何把相邻两段的处理扩展到整个序列呢,鉴于整个\(b\)序列是递增的,可以用单调栈实现,栈中的比较方式就是上述对于相邻两段的处理
现在解除一开始自己设置的限制,将\(a_i\)设为\(a_i-i\)即可将非严格上升序列的做法转移到严格上升序列的做法
附:证明:其实就是小学奥数题 对于一段数\(\{c_i\}\)选取\(x\)使得\(\sum \bigl |x-c_i \bigr |\),最小的\(x\)值的一个取值为\(\{c_i\}\)序列的中位数:
反证法:设原序列有\(n\)个元素,则比\(x\)大/小的数有\(\frac n2\)个,若\(x\)变小或变大,则若越过序列中另一个值时,比\(x\)大/小的数有\(\frac n2±1\)个,统计答案时只会增加\(2\)或不变
#include<bits/stdc++.h>
using namespace std;
typedef long long ll;
#define rg register
struct ios {
inline char read(){
static const int IN_LEN=1<<18|1;
static char buf[IN_LEN],*s,*t;
return (s==t)&&(t=(s=buf)+fread(buf,1,IN_LEN,stdin)),s==t?-1:*s++;
}
template <typename _Tp> inline ios & operator >> (_Tp&x){
static char c11,boo;
for(c11=read(),boo=0;!isdigit(c11);c11=read()){
if(c11==-1)return *this;
boo|=c11==‘-‘;
}
for(x=0;isdigit(c11);c11=read())x=x*10+(c11^‘0‘);
boo&&(x=-x);
return *this;
}
} io;
const int N=1001000;
struct Leftist_Tree{int l,r,dis,val;}t[N];
struct node{
int l,r,rt,sz,val;
node(){}
node(const int&L,const int&id){l=L,r=rt=id,sz=1,val=t[id].val;}
}h[N];
int n,top;
inline int merge(int u,int v){
if(!u||!v)return u|v;
if(t[u].val<t[v].val||(t[u].val==t[v].val&&u>v))swap(u,v);
int&l=t[u].l,&r=t[u].r;
r=merge(r,v);
if(t[l].dis<t[r].dis)swap(l,r);
t[u].dis=t[r].dis+1;
return u;
}
inline int del(int u){return merge(t[u].l,t[u].r);}
void work(){
io>>n;
for(rg int i=1;i<=n;++i)io>>t[i].val,t[i].val-=i;
h[top=1]=node(1,1);
for(rg int i=2;i<=n;++i){
int l=h[top].r+1;
h[++top]=node(l,i);
while(top^1&&h[top-1].val>h[top].val){
--top;
h[top].rt=merge(h[top].rt,h[top+1].rt);
h[top].r=h[top+1].r;
h[top].sz+=h[top+1].sz;
while(h[top].sz>((h[top].r-h[top].l+2)>>1)){
--h[top].sz;
h[top].rt=del(h[top].rt);
}h[top].val=t[h[top].rt].val;
}
}return ;
}
void Print(){
ll Ans=0;
for(rg int i=1,p=1;i<=n;++i){
if(i>h[p].r)++p;
Ans+=abs(h[p].val-t[i].val);
}printf("%lld\n",Ans);
for(rg int i=1,p=1;i<=n;++i){
if(i>h[p].r)++p;
printf("%d ",h[p].val+i);
}putchar(‘\n‘);
return ;
}
int main(){
work();
Print();
return 0;
}标签:const com tis typename 小学 区间 台阶 bit type
原文地址:https://www.cnblogs.com/penth/p/9239977.html