标签:blog http io os for sp div 2014 c
不妨先把所有要求的素数的对的个数写出来
f(2)=u(1)G(2)+u(2)*G(2*2)+u(3)*G(2*3)+.....u(k2)*G(2*k2)
f(3)=u(1)G(3)+u(2)*G(2*3)+u(3)*G(3*3)+.....u(k3)*G(3*k3)
....
f(p)=u(1)G(p)+u(2)*G(2*p)+u(3)*G(p*3)+.....u(kp)*G(p*kp)
相加之后
会发现,其实G的变量是从1~n的。于是,最重要便 是求出其合并后的系数了。怎么样求呢?我不懂,看了看别人的,发现竟有这样一个等式
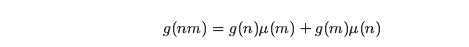
于是,在线性筛选法上加上相关语句就可以求到相应的系数了。线性筛选:
http://wenku.baidu.com/link?url=ufaT0myBu70JxfkYUDsAtgPin6Y4uI8DwU43QCiQoqOOY9xhJJg3jr6DVn24sF2mAXRYrM6Hjrai-vMwfQ7-5IbQVDqwCGIwVzZR6xMVxiq
#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#define N 10000005
using namespace std;
typedef long long LL;
bool check[N];
int mu[N],tot;
int prime[N],he[N];
void initial(){
memset(check,false,sizeof(check));
memset(he,0,sizeof(he));
mu[1]=1;
tot=0;
for(LL i=2;i<N;i++){
if(!check[i]){
prime[tot++]=i;
he[i]=1;
mu[i]=-1;
}
for(LL j=0;j<tot;j++){
if(i*prime[j]>N) break;
check[i*prime[j]]=true;
if(i%prime[j]==0){
mu[i*prime[j]]=0;
he[i * prime[j]] = mu[i];
break;
}
else{
he[i*prime[j]] = mu[i] - he[i];
mu[i*prime[j]]=-mu[i];
}
}
}
for(int i=2;i<N;i++)
he[i]+=he[i-1];
}
LL slove(int tx,int ty){
if(tx>ty) swap(tx,ty);
int l=1,r,p1,p2;
LL ans=0;
while(l<=tx){
r=min(min(tx/(p1=tx/l),ty/(p2=ty/l)),tx);
ans+=((LL) p1*(LL )p2*(LL )(he[r]-he[l-1]));
l=r+1;
}
return ans;
}
int main(){
initial();
int T;
scanf("%d",&T);
int x,y,tx,ty;
LL ans;
while(T--){
scanf("%d%d",&x,&y);
ans=slove(x,y);
printf("%lld\n",ans);
}
return 0;
}
标签:blog http io os for sp div 2014 c
原文地址:http://www.cnblogs.com/jie-dcai/p/4006162.html