标签:namespace math fine math.h line alt 建议 sqrt ret
题目链接:http://www.51nod.com/onlineJudge/questionCode.html#!problemId=1130
斯特林公式能够近似的求出n的阶乘的值 然后我们再用 求位数公式 log10(n)+1就可以求出答案
斯特林公式 sqrt( 2*pi*n ) * pow( n/e , n)
斯特林公式 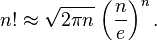
而且 log10()内的乘法 又可以转化为加法 那么我们就可以对斯特林公式优化为
0.5 * log10( 2*PI*n ) + n*log10( n/e )
要注意 公式里用到了 e 和 派 我们定义他们有两种写法
#define PI acos(-1.0) #define E exp(1.0) const double e = 2.718281828459; const double pi = 3.1415926535;
建议大家使用第一种
尤其是这道题 数据特别的卡 刚开始 我定义e =2.71828182845; 疯狂的wa wa了七八遍后 我又多加了一位e =2.718281828459 就对了。。。
所以 由于有的时候 我们不知道数据需要几位 用赋值的定义方式就可能出错
代码
#include<stdio.h> #include<string.h> #include<iostream> #include<algorithm> #include<math.h> using namespace std; typedef long long ll; #define PI acos(-1.0) #define E exp(1.0) const double e = 2.718281828459; const double pi = 3.1415926535; int T; double n; int main(){ scanf("%d",&T); while(T--){ scanf("%lf",&n); ll ans=(ll)(0.5*log10(2*PI*n) + n*(log10(n/E)) +1); printf("%I64d\n",ans); } return 0; }
标签:namespace math fine math.h line alt 建议 sqrt ret
原文地址:https://www.cnblogs.com/precious-ZPF/p/9492562.html