标签:说明 strong html 图片 bsp 帮助 ems getc vector
小Z有一片森林,含有N个节点,每个节点上都有一个非负整数作为权值。初始的时候,森林中有M条边。
小Z希望执行T个操作,操作有两类:
Q x y k查询点x到点y路径上所有的权值中,第k小的权值是多少。此操作保证点x和点y连通,同时这两个节点的路径上至少有k个点。L x y在点x和点y之间连接一条边。保证完成此操作后,仍然是一片森林。为了体现程序的在线性,我们把输入数据进行了加密。设lastans为程序上一次输出的结果,初始的时候lastans为0。
Q x y k,其真实操作为Q x^lastans y^lastans k^lastans。L x y,其真实操作为L x^lastans y^lastans。其中^运算符表示异或,等价于pascal中的xor运算符。请写一个程序來帮助小Z完成这些操作。
对于所有的数据,n,m,T<=8∗1048*10^48∗104.
输入格式:
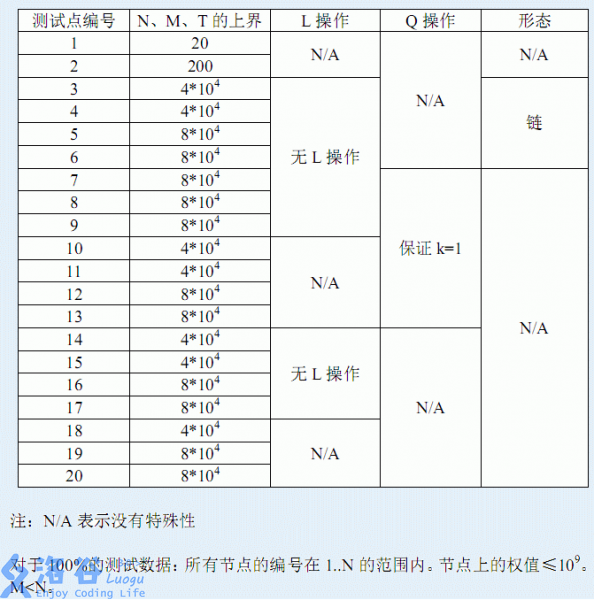
第一行包含一个正整数testcase,表示当前测试数据的测试点编号。保证1<=testcase<=20。
第二行包含三个整数N,M,T,分别表示节点数、初始边数、操作数。
第三行包含N个非负整数表示 N个节点上的权值。
接下来 M行,每行包含两个整数x和 y,表示初始的时候,点x和点y 之间有一条无向边。
接下来 T行,每行描述一个操作,格式为”Q x y k“或者”L x y “,其含义见题目描述部分。
输出格式:
对于每一个第一类操作,输出一个非负整数表示答案。
1 8 4 8 1 1 2 2 3 3 4 4 4 7 1 8 2 4 2 1 Q 8 7 3 Q 3 5 1 Q 10 0 0 L 5 4 L 3 2 L 0 7 Q 9 2 5 Q 6 1 6
2 2 1 4 2
对于第一个操作 Q 8 7 3,此时 lastans=0,所以真实操作为Q 8^0 7^0 3^0,也即Q 8 7 3。点8到点7的路径上一共有5个点,其权值为4 1 1 2 4。
这些权值中,第三小的为 2,输出 2,lastans变为2。
对于第二个操作 Q 3 5 1 ,此时lastans=2,所以真实操作为Q 3^2 5^2 1^2 ,也即Q 1 7 3。点1到点7的路径上一共有4个点,其权值为 1 1 2 4 。
这些权值中,第三小的为2,输出2,lastans变为 2。之后的操作类似。
【思路分析】
一看就是大力数据结构,没啥可说的,就是树上主席树带一个启发式合并,复杂度O(nlog^2),离散化后即可AC
但是比较坑爹的是这里的T是测试点编号,不是数据组数,害得我TLE了一上午。。。垃圾出题人
【代码实现】
1 #include<cstdio> 2 #include<cctype> 3 #include<cstring> 4 #include<algorithm> 5 #include<vector> 6 using namespace std; 7 typedef long long ll; 8 void read(int &v) 9 { 10 int f;char ch; 11 while(!isdigit(ch=getchar())&&ch!=‘-‘); ch==‘-‘?(f=-1,v=0):(f=1,v=ch-‘0‘); 12 while(isdigit(ch=getchar())) v=v*10+ch-‘0‘;v=v*f; 13 } 14 const int N=8e4+5; 15 struct sd{ 16 int son[2],sum; 17 }t[N*200]; 18 struct e{ 19 int next,to; 20 e(){}; 21 e(int a,int b){next=a,to=b;} 22 }edge[N<<1]; 23 int head[N],val[N],tmp[N],root[N],siz[N],up[N][18],rt[N],dep[N]; 24 int n,m,q,T,cur,cnt,tot,lastans; 25 void clear() 26 { 27 cnt=0,cur=0,tot=0,lastans=0; 28 memset(head,0,sizeof(head)); 29 memset(rt,0,sizeof(rt)); 30 memset(siz,0,sizeof(siz)); 31 for(int i=1;i<=n;i++) root[i]=i,up[i][0]=0; 32 } 33 void insert(int &v,int vv,int l,int r,int pos) 34 { 35 v=++cnt; 36 t[v]=t[vv],t[v].sum++; 37 if(l==r) return; 38 int mid=l+r>>1; 39 if(pos<=mid) insert(t[v].son[0],t[vv].son[0],l,mid,pos); 40 else insert(t[v].son[1],t[vv].son[1],mid+1,r,pos); 41 } 42 void dfs(int v,int ff,int rrt) 43 { 44 insert(rt[v],rt[ff],1,tot,val[v]); 45 dep[v]=dep[ff]+1,up[v][0]=ff,root[v]=rrt,siz[rrt]++; 46 for(int i=1;i<=17;i++) up[v][i]=up[up[v][i-1]][i-1]; 47 for(int i=head[v];i;i=edge[i].next) 48 { 49 int to=edge[i].to; 50 if(to==ff) continue; 51 dfs(to,v,rrt); 52 } 53 } 54 void add_edge(int a,int b) 55 { 56 edge[++cur]=e(head[a],b),head[a]=cur; 57 edge[++cur]=e(head[b],a),head[b]=cur; 58 } 59 int LCA(int a,int b) 60 { 61 if(dep[a]<dep[b]) swap(a,b); 62 int len=dep[a]-dep[b]; 63 for(int i=17;i>=0;i--) if(len&(1<<i)) a=up[a][i]; 64 if(a==b) return a; 65 for(int i=17;i>=0;i--) if(up[a][i]!=up[b][i]) a=up[a][i],b=up[b][i]; 66 return up[a][0]; 67 } 68 int query(int v1,int v2,int v3,int v4,int l,int r,int k) 69 { 70 if(l==r) return tmp[l]; 71 int mid=l+r>>1,tmp=t[t[v1].son[0]].sum+t[t[v2].son[0]].sum-t[t[v3].son[0]].sum-t[t[v4].son[0]].sum; 72 if(tmp>=k) return query(t[v1].son[0],t[v2].son[0],t[v3].son[0],t[v4].son[0],l,mid,k); 73 else return query(t[v1].son[1],t[v2].son[1],t[v3].son[1],t[v4].son[1],mid+1,r,k-tmp); 74 } 75 int main() 76 { 77 read(T),T=1; 78 while(T--) 79 { 80 int a,b,k;char opt[3]; 81 read(n),read(m),read(q); 82 clear(); 83 for(int i=1;i<=n;i++) read(val[i]),tmp[i]=val[i]; 84 sort(tmp+1,tmp+1+n); 85 tot=unique(tmp+1,tmp+1+n)-tmp-1; 86 for(int i=1;i<=n;i++) val[i]=lower_bound(tmp+1,tmp+1+tot,val[i])-tmp; 87 for(int i=1;i<=m;i++) read(a),read(b),add_edge(a,b); 88 for(int i=1;i<=n;i++) if(root[i]==i) dfs(i,0,i); 89 while(q--) 90 { 91 scanf("%s",opt),read(a),read(b),a^=lastans,b^=lastans; 92 if(opt[0]==‘L‘){ 93 add_edge(a,b); 94 if(siz[root[a]]<siz[root[b]]) swap(a,b); 95 dfs(b,a,root[a]); 96 } 97 else { 98 read(k),k^=lastans; 99 int lca=LCA(a,b); 100 lastans=query(rt[a],rt[b],rt[lca],rt[up[lca][0]],1,tot,k); 101 printf("%d\n",lastans); 102 } 103 } 104 } 105 return 0; 106 }
标签:说明 strong html 图片 bsp 帮助 ems getc vector
原文地址:https://www.cnblogs.com/genius777/p/9785723.html