标签:如何 c代码 导致 误差 http name local 结果 tin
题目链接:
2 0 0 10 10 0 15 0 15 5 0 0 10 0 0 5 0 5 5
Yes No
基础知识回顾:
点到直线距离公式:
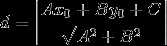
余弦定理:
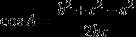
分析:
对于给定的三角形和圆,我们考虑相交的情况:


① 三角形有一点在圆内,有一点在圆外。
② 三角形有一点在圆上。
③三角形三点都在圆外,但有一条边与圆相交或相切。
前两种情况比较好写,只需要判断三角形三个端点到圆心的距离与半径的关系即可。
对于第三种情况,我们可以先判断圆心到三角形三条边的距离,如果有一条边到圆心的直线距离小于等于半径,我们进而去判断圆心到这条边所在直线的垂足是否在这条边上。如何去判断呢?
我们可以利用余弦定理,只要圆心与这条边的两个端点所成的角均为锐角(即cosα>0),那么垂足必然落在这条边上。
以下是AC代码:
#include<iostream> #include<cstdio> #include<cmath> using namespace std; struct triangle//用结构体来存三角形三点的坐标 { double x[3],y[3]; }; double x,y,r; triangle a; //计算(x1,y1)与(x2,y2)之间的距离的平方 double point_dist(double x1,double y1,double x2,double y2) { return (x1 - x2) * (x1 - x2) + (y1 - y2) * (y1 - y2); } //计算圆心(x,y)到直线Ax+By+C=0的距离的平方 double line_dist(double A,double B,double C) { double ans = ( (A*x + B*y + C) * (A*x + B*y + C) ) / (A*A + B*B); return ans > 0 ? ans : -ans; } double f(double a,double b,double c)//余弦定理 { return (b + c - a) / (2.0 * sqrt(b * c)); } int main() { int i,j,t; cin>>t; while(t--) { //Input scanf("%lf%lf%lf",&x,&y,&r); for(i = 0;i < 3; i++) scanf("%lf%lf",&a.x[i],&a.y[i]); //Solve double dis1[3],dis2[3],dis3[3]; //dis1存放三角形三点到圆心距离的平方 dis1[0] = point_dist(x,y,a.x[0],a.y[0]); dis1[1] = point_dist(x,y,a.x[1],a.y[1]); dis1[2] = point_dist(x,y,a.x[2],a.y[2]); //dis2存放三角形三条边长的平方 dis2[0] = point_dist(a.x[0],a.y[0],a.x[1],a.y[1]); dis2[1] = point_dist(a.x[1],a.y[1],a.x[2],a.y[2]); dis2[2] = point_dist(a.x[2],a.y[2],a.x[0],a.y[0]); //dis3存放三角形三条边到圆心的直线距离的平方 dis3[0] = line_dist(a.y[0]-a.y[1],a.x[1]-a.x[0],(a.x[0]-a.x[1])*a.y[0]+(a.y[1]-a.y[0])*a.x[0]); dis3[1] = line_dist(a.y[1]-a.y[2],a.x[2]-a.x[1],(a.x[1]-a.x[2])*a.y[1]+(a.y[2]-a.y[1])*a.x[1]); dis3[2] = line_dist(a.y[2]-a.y[0],a.x[0]-a.x[2],(a.x[2]-a.x[0])*a.y[2]+(a.y[0]-a.y[2])*a.x[2]); double t1,t2; t1 = min(dis1[0],min(dis1[1],dis1[2]));//t1为三点到圆心距离最小的那个 t2 = max(dis1[0],max(dis1[1],dis1[2]));//t2为三点到圆心距离最大的那个 if(t1 <= r*r &&t2 >= r*r)//一点在圆内,一点在圆外或有一点在圆上 cout<<"Yes"<<endl; else if(t1 > r*r)//三点都在圆外 { if(dis3[0] <= r*r)//dis3[0]是由点1和点2连接起来的边到圆心的距离 { if(f(dis1[0],dis2[0],dis1[1]) > 0 && f(dis1[1],dis2[0],dis1[0]) > 0) { cout<<"Yes"<<endl; continue; } } if(dis3[1] <= r*r)//dis3[1]是由点2和点3连接起来的边到圆心的距离 { if(f(dis1[1],dis2[1],dis1[2]) > 0 && f(dis1[2],dis2[1],dis1[1]) > 0) { cout<<"Yes"<<endl; continue; } } if(dis3[2] <= r*r)//dis3[2]是由点1和点2连接起来的边到圆心的距离 { if(f(dis1[0],dis2[2],dis1[2]) > 0 && f(dis1[2],dis2[2],dis1[0]) > 0) { cout<<"Yes"<<endl; continue; } } cout<<"No"<<endl; } else cout<<"No"<<endl; } return 0; }
代码需注意的几点:
② 已知三角形一条边的两端点(x1,y1)和(x2,y2),我们将这条边的直线方程斜截式y=kx+b转换为一般式ax+by+c=0所得结果为 (y1-y2)x+(x2-x1)y+(x1-x2)y1+(y2-y1)x1=0,这也是给dis3数组赋值的依据。
标签:如何 c代码 导致 误差 http name local 结果 tin
原文地址:https://www.cnblogs.com/chdforestsea/p/9795342.html