标签:技术 就是 函数 pac nbsp ace void const tar
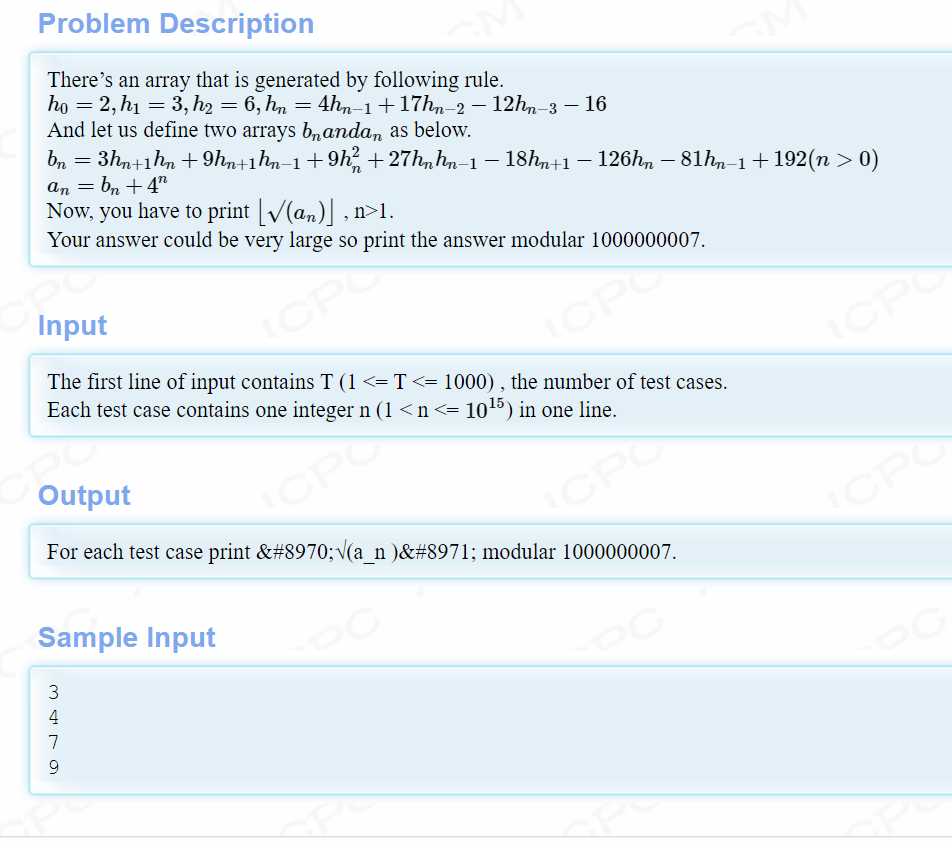
题意:给出,三个函数,h,b,a,然后T次询问,每次给出n,求sqrt(an);
思路:不会推,但是感觉a应该是线性的,这个时候我们就可以用BM线性递推,自己求出前几项,然后放到模板里,就可以求了。
数据范围在1e15,1000组都可以秒过。
那么主要的问题就是得确保是线性的,而且得求出前几项。
#include<bits/stdc++.h> using namespace std; #define rep(i,a,n) for (int i=a;i<n;i++) #define per(i,a,n) for (int i=n-1;i>=a;i--) #define pb push_back #define mp make_pair #define all(x) (x).begin(),(x).end() #define fi first #define se second #define SZ(x) ((int)(x).size()) typedef vector<int> VI; typedef long long ll; typedef pair<int,int> PII; const ll mod=1000000007; ll powmod(ll a,ll b) {ll res=1;a%=mod; assert(b>=0); for(;b;b>>=1){if(b&1)res=res*a%mod;a=a*a%mod;}return res;} ll n; namespace linear_seq { const int N=10010; ll res[N],base[N],_c[N],_md[N]; vector<int> Md; void mul(ll *a,ll *b,int k) { rep(i,0,k+k) _c[i]=0; rep(i,0,k) if (a[i]) rep(j,0,k) _c[i+j]=(_c[i+j]+a[i]*b[j])%mod; for (int i=k+k-1;i>=k;i--) if (_c[i]) rep(j,0,SZ(Md)) _c[i-k+Md[j]]=(_c[i-k+Md[j]]-_c[i]*_md[Md[j]])%mod; rep(i,0,k) a[i]=_c[i]; } int solve(ll n,VI a,VI b) { ll ans=0,pnt=0; int k=SZ(a); assert(SZ(a)==SZ(b)); rep(i,0,k) _md[k-1-i]=-a[i];_md[k]=1; Md.clear(); rep(i,0,k) if (_md[i]!=0) Md.push_back(i); rep(i,0,k) res[i]=base[i]=0; res[0]=1; while ((1ll<<pnt)<=n) pnt++; for (int p=pnt;p>=0;p--) { mul(res,res,k); if ((n>>p)&1) { for (int i=k-1;i>=0;i--) res[i+1]=res[i];res[0]=0; rep(j,0,SZ(Md)) res[Md[j]]=(res[Md[j]]-res[k]*_md[Md[j]])%mod; } } rep(i,0,k) ans=(ans+res[i]*b[i])%mod; if (ans<0) ans+=mod; return ans; } VI BM(VI s) { VI C(1,1),B(1,1); int L=0,m=1,b=1; rep(n,0,SZ(s)) { ll d=0; rep(i,0,L+1) d=(d+(ll)C[i]*s[n-i])%mod; if (d==0) ++m; else if (2*L<=n) { VI T=C; ll c=mod-d*powmod(b,mod-2)%mod; while (SZ(C)<SZ(B)+m) C.pb(0); rep(i,0,SZ(B)) C[i+m]=(C[i+m]+c*B[i])%mod; L=n+1-L; B=T; b=d; m=1; } else { ll c=mod-d*powmod(b,mod-2)%mod; while (SZ(C)<SZ(B)+m) C.pb(0); rep(i,0,SZ(B)) C[i+m]=(C[i+m]+c*B[i])%mod; ++m; } } return C; } int gao(VI a,ll n) { VI c=BM(a); c.erase(c.begin()); rep(i,0,SZ(c)) c[i]=(mod-c[i])%mod; return solve(n,c,VI(a.begin(),a.begin()+SZ(c))); } }; int main() { int T; scanf("%d",&T); while(T--){ scanf("%lld",&n); printf("%lld\n", linear_seq::gao(VI{31,197,1255,7997,50959,324725,2069239,13185773,84023455,535421093,411853810},n-2))
; } } //
再比如HDU6185,很显然是线性的,用16*16的矩阵来做会T,我可以用状压DP暴力求出前几项,然后套这个。
#include<bits/stdc++.h> using namespace std; #define rep(i,a,n) for (int i=a;i<n;i++) #define per(i,a,n) for (int i=n-1;i>=a;i--) #define pb push_back #define mp make_pair #define all(x) (x).begin(),(x).end() #define fi first #define se second #define SZ(x) ((int)(x).size()) typedef vector<int> VI; typedef long long ll; typedef pair<int,int> PII; const ll mod=1000000007; ll powmod(ll a,ll b) {ll res=1;a%=mod; assert(b>=0); for(;b;b>>=1){if(b&1)res=res*a%mod;a=a*a%mod;}return res;} ll _,n; namespace linear_seq { const int N=10010; ll res[N],base[N],_c[N],_md[N]; vector<int> Md; void mul(ll *a,ll *b,int k) { rep(i,0,k+k) _c[i]=0; rep(i,0,k) if (a[i]) rep(j,0,k) _c[i+j]=(_c[i+j]+a[i]*b[j])%mod; for (int i=k+k-1;i>=k;i--) if (_c[i]) rep(j,0,SZ(Md)) _c[i-k+Md[j]]=(_c[i-k+Md[j]]-_c[i]*_md[Md[j]])%mod; rep(i,0,k) a[i]=_c[i]; } int solve(ll n,VI a,VI b) { ll ans=0,pnt=0; int k=SZ(a); assert(SZ(a)==SZ(b)); rep(i,0,k) _md[k-1-i]=-a[i];_md[k]=1; Md.clear(); rep(i,0,k) if (_md[i]!=0) Md.push_back(i); rep(i,0,k) res[i]=base[i]=0; res[0]=1; while ((1ll<<pnt)<=n) pnt++; for (int p=pnt;p>=0;p--) { mul(res,res,k); if ((n>>p)&1) { for (int i=k-1;i>=0;i--) res[i+1]=res[i];res[0]=0; rep(j,0,SZ(Md)) res[Md[j]]=(res[Md[j]]-res[k]*_md[Md[j]])%mod; } } rep(i,0,k) ans=(ans+res[i]*b[i])%mod; if (ans<0) ans+=mod; return ans; } VI BM(VI s) { VI C(1,1),B(1,1); int L=0,m=1,b=1; rep(n,0,SZ(s)) { ll d=0; rep(i,0,L+1) d=(d+(ll)C[i]*s[n-i])%mod; if (d==0) ++m; else if (2*L<=n) { VI T=C; ll c=mod-d*powmod(b,mod-2)%mod; while (SZ(C)<SZ(B)+m) C.pb(0); rep(i,0,SZ(B)) C[i+m]=(C[i+m]+c*B[i])%mod; L=n+1-L; B=T; b=d; m=1; } else { ll c=mod-d*powmod(b,mod-2)%mod; while (SZ(C)<SZ(B)+m) C.pb(0); rep(i,0,SZ(B)) C[i+m]=(C[i+m]+c*B[i])%mod; ++m; } } return C; } int gao(VI a,ll n) { VI c=BM(a); c.erase(c.begin()); rep(i,0,SZ(c)) c[i]=(mod-c[i])%mod; return solve(n,c,VI(a.begin(),a.begin()+SZ(c))); } }; int main() { while(~scanf("%lld",&n)){ printf("%d\n",linear_seq::gao(VI{1,5,11,36,95,281,781,2245},n-1)); } }
HDU - 6172:Array Challenge (BM线性递推)
标签:技术 就是 函数 pac nbsp ace void const tar
原文地址:https://www.cnblogs.com/hua-dong/p/9886167.html