标签:style blog http color io 使用 for strong sp
Given an index k, return the kth row of the Pascal‘s triangle.
For example, given k = 3,
Return [1,3,3,1].
Note:
Could you optimize your algorithm to use only O(k) extra space?
杨辉三角想必大家并不陌生,应该最早出现在初高中的数学中,其实就是二项式系数的一种写法。
1 1 1 1 2 1 1 3 3 1 1 4 6 4 1 1 5 10 10 5 1 1 6 15 20 15 6 1 1 7 21 35 35 21 7 1 1 8 28 56 70 56 28 8 1
杨辉三角形第n层(顶层称第0层,第1行,第n层即第n+1行,此处n为包含0在内的自然数)正好对应于二项式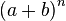 展开的系数。例如第二层1 2 1是幂指数为2的二项式
展开的系数。例如第二层1 2 1是幂指数为2的二项式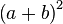 展开形式
展开形式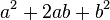 的系数。
的系数。
杨辉三角主要有下列五条性质:
 行的数字个数为
行的数字个数为 个。
个。 行的第
行的第 个数字为组合数
个数字为组合数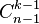 。
。 行数字和为
行数字和为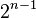 。
。 行第
行第 个数字等于第
个数字等于第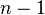 行的第
行的第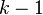 个数字与第
个数字与第 个数字的和)。这是因为有组合恒等式:
个数字的和)。这是因为有组合恒等式: 。可用此性质写出整个杨辉三角形。
。可用此性质写出整个杨辉三角形。
由于题目有额外限制条件,程序只能使用O(k)的额外空间,那么这样就不能把每行都算出来,而是要用其他的方法, 我最先考虑用的是第三条性质,算出每个组合数来生成第n行系数,代码如下:
class Solution { public: vector<int> getRow(int rowIndex) { vector<int> out; if (rowIndex < 0) return out; for (int i = 0; i <= rowIndex; ++i) { if ( i == 0 || i == rowIndex) out.push_back(1); else out.push_back (computeCnk(rowIndex, i)); } return out; } int computeCnk(int n, int k) { if (k > n) return 0; else if (k > n/2) k = n - k; int numerator = 1, denomator = 1; for (int i = 0; i < k; ++i) { numerator *= n - i; denomator *= k - i; } if (denomator != 0) return numerator/denomator; else return 0; } };
本地调试输出前十行,没啥问题,拿到OJ上测试,程序在第18行跪了,中间有个系数不正确。那么问题出在哪了呢,仔细找找,原来出在计算组合数那里,由于算组合数时需要算连乘,而整形数int的数值范围只有-32768到32768之间,那么一旦n值过大,连乘肯定无法计算。而丧心病狂的OJ肯定会测试到成百上千行,所以这个方法不行。那么我们再来考虑利用第五条性质,除了第一个和最后一个数字之外,其他的数字都是上一行左右两个值之和。那么我们只需要两个for循环,除了第一个数为1之外,后面的数都是上一次循环的数值加上它前面位置的数值之和,不停地更新每一个位置的值,便可以得到第n行的数字,具体实现代码如下:
class Solution { public: vector<int> getRow(int rowIndex) { vector<int> out; if (rowIndex < 0) return out; out.assign(rowIndex + 1, 0); for (int i = 0; i <= rowIndex; ++i) { if ( i == 0) { out[0] = 1; continue; } for (int j = rowIndex; j >= 1; --j) { out[j] = out[j] + out[j-1]; } } return out; } };
标签:style blog http color io 使用 for strong sp
原文地址:http://www.cnblogs.com/grandyang/p/4031536.html