HDU1506_Largest Rectangle in a Histogram

标签：des   style   blog   http   io   os   ar   java   for   

Problem Description
A histogram is a polygon composed of a sequence of rectangles aligned at a common base line. The rectangles have equal widths but may have different heights. For example, the figure on the left shows the histogram that consists of rectangles with the heights 2, 1, 4, 5, 1, 3, 3, measured in units where 1 is the width of the rectangles:


Usually, histograms are used to represent discrete distributions, e.g., the frequencies of characters in texts. Note that the order of the rectangles, i.e., their heights, is important. Calculate the area of the largest rectangle in a histogram that is aligned at the common base line, too. The figure on the right shows the largest aligned rectangle for the depicted histogram.
 
Input
The input contains several test cases. Each test case describes a histogram and starts with an integer n, denoting the number of rectangles it is composed of. You may assume that 1 <= n <= 100000. Then follow n integers h1, ..., hn, where 0 <= hi <= 1000000000. These numbers denote the heights of the rectangles of the histogram in left-to-right order. The width of each rectangle is 1. A zero follows the input for the last test case.
 
Output
For each test case output on a single line the area of the largest rectangle in the specified histogram. Remember that this rectangle must be aligned at the common base line.
 
Sample Input
7 2 1 4 5 1 3 3
4 1000 1000 1000 1000
0

Sample Output
8
4000
 
Source
University of Ulm Local Contest 2003

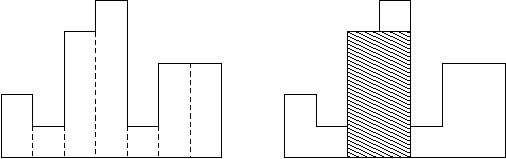
题目大意：给你一个直方图，告诉你各个条形矩形的高度，求基线对齐构成的矩形中面积

最大的矩形的面积对于每一个矩形。面积 = h[i]*(j-k+1),其中j，k是左右边界，h[i]是矩形

的高。并且对于j <= x <= k，h[i] <= h[x]。

本题中，找到左右边界j，k是关键。

利用动态规划的方法，对于位置i，如果左边条形矩形的高度大于它本身，那么左边的左边

界一定也满足位置i的左边界。同理如果右边条形矩形的高度大于它本身，那么右边的右边

界也一定满足位置i的右边界。迭代循环下去。直到找到i的左右边界。

#include<stdio.h>
#include<string.h>

int l[100010],r[100010];
__int64 h[100010];
int main()
{
    int N;
    while(~scanf("%d",&N) && N!=0)
    {
        memset(h,0,sizeof(h));
        for(int i = 1; i <= N; i++)
        {
            scanf("%I64d",&h[i]);
            l[i] = r[i] = i;
        }

        l[0] = 1;
        r[N+1] = N;
        h[0] = -1;
        h[N+1] = -1;
        //这上边不加就会超时，不加的话下边就可能一直while，跳不出循环
        for(int i = 1; i <= N; i++)
        {
            while(h[l[i]-1] >= h[i])//找位置i的左边界
                l[i] = l[l[i]-1];
        }
        for(int i = N; i >= 1; i--)
        {
            while(h[r[i]+1] >= h[i])//找位置i的右边界
                r[i] = r[r[i]+1];
        }
        __int64 MaxArea = -0xffffff0;
        for(int i = 1; i <= N; i++)
        {
            if(h[i]*(r[i]-l[i]+1) > MaxArea)
                MaxArea = h[i]*(r[i]-l[i]+1);
        }
        printf("%I64d\n",MaxArea);
    }
    return 0;
}

HDU1506_Largest Rectangle in a Histogram

标签：des   style   blog   http   io   os   ar   java   for   

原文地址：http://blog.csdn.net/lianai911/article/details/40208265