标签:卖出 memset cst clu eof class style 双向 deb
C 国有 n 个大城市和 m 条道路,每条道路连接这 n 个城市中的某两个城市。任意两个城市之间最多只有一条道路直接相连。这 m 条道路中有一部分为单向通行的道路,一部分为双向通行的道路,双向通行的道路在统计条数时也计为 1 条。
C 国幅员辽阔,各地的资源分布情况各不相同,这就导致了同一种商品在不同城市的价格不一定相同。但是,同一种商品在同一个城市的买入价和卖出价始终是相同的。
商人阿龙来到 C 国旅游。当他得知同一种商品在不同城市的价格可能会不同这一信息之后,便决定在旅游的同时,利用商品在不同城市中的差价赚回一点旅费。设 C 国 n 个城市的标号从 1~ n,阿龙决定从 1 号城市出发,并最终在 n 号城市结束自己的旅行。在旅游的过程中,任何城市可以重复经过多次,但不要求经过所有 n 个城市。阿龙通过这样的贸易方式赚取旅费:他会选择一个经过的城市买入他最喜欢的商品――水晶球,并在之后经过的另一个城市卖出这个水晶球,用赚取的差价当做旅费。由于阿龙主要是来 C 国旅游,他决定这个贸易只进行最多一次,当然,在赚不到差价的情况下他就无需进行贸易。
假设 C 国有 5 个大城市,城市的编号和道路连接情况如下图,单向箭头表示这条道路为单向通行,双向箭头表示这条道路为双向通行。
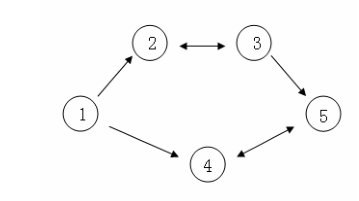
假设 1~n 号城市的水晶球价格分别为 4,3,5,6,1。
阿龙可以选择如下一条线路:1->2->3->5,并在 2 号城市以 3 的价格买入水晶球,在 3号城市以 5 的价格卖出水晶球,赚取的旅费数为 2。
阿龙也可以选择如下一条线路 1->4->5->4->5,并在第 1 次到达 5 号城市时以 1 的价格买入水晶球,在第 2 次到达 4 号城市时以 6 的价格卖出水晶球,赚取的旅费数为 5。
现在给出 n 个城市的水晶球价格,m 条道路的信息(每条道路所连接的两个城市的编号以及该条道路的通行情况)。请你告诉阿龙,他最多能赚取多少旅费。
输入格式:
第一行包含 2 个正整数 n 和 m,中间用一个空格隔开,分别表示城市的数目和道路的数目。
第二行 n 个正整数,每两个整数之间用一个空格隔开,按标号顺序分别表示这 n 个城市的商品价格。
接下来 m 行,每行有 3 个正整数,x,y,z,每两个整数之间用一个空格隔开。如果 z=1,表示这条道路是城市 x 到城市 y 之间的单向道路;如果 z=2,表示这条道路为城市 x 和城市y 之间的双向道路。
输出格式:
输出文件 trade.out 共 1 行,包含 1 个整数,表示最多能赚取的旅费。如果没有进行贸易,则输出 0。
【数据范围】
输入数据保证 1 号城市可以到达 n 号城市。
对于 10%的数据,1≤n≤6。
对于 30%的数据,1≤n≤100。
对于 50%的数据,不存在一条旅游路线,可以从一个城市出发,再回到这个城市。
对于 100%的数据,1≤n≤100000,1≤m≤500000,1≤x,y≤n,1≤z≤2,1≤各城市
水晶球价格≤100。
树dp写法:
//2018年4月30日12:20:22 #include <iostream> #include <cstdio> #include <cstring> using namespace std; const int N = 100001; const int M = 500001; const int INF = 1e9+7; int n, m; int w[N]; int fir[N], nxt[M], to[M], edge_num; void addEdge(int x, int y){ to[++edge_num] = y; nxt[edge_num] = fir[x]; fir[x] = edge_num; } int f[N], mi[N]; void dfs(int x, int minx, int pre){ int flag = 1; minx = min(w[x], minx); if(mi[x] > minx) mi[x] = minx, flag = 0; int maxx = max(f[pre], w[x]-minx); if(f[x] < maxx) f[x] = maxx, flag = 0; if(flag) return; for(int i=fir[x]; i; i=nxt[i]) dfs(to[i], minx, x); } int main(){ scanf("%d%d", &n, &m); for(int i=1; i<=n; i++) scanf("%d", &w[i]); for(int i=1; i<=N; i++) mi[i] = INF; for(int i=1; i<=m; i++){ int x, y, z; scanf("%d%d%d", &x, &y, &z); if(z == 1){ addEdge(x, y); }else if(z == 2){ addEdge(x, y); addEdge(y, x); } } dfs(1, INF, 0); printf("%d\n", f[n]); return 0; }
最短路写法:
#include<cstdio> #include<algorithm> #include<iostream> #include<cstring> #include<cstdlib> using namespace std; const int maxn=1e5+5; const int maxm=5e5+5; int first[maxn][2],next[maxm][2],to[maxm][2],w[maxn]; int edge_count[2]; inline void add(int x,int y,int b){ // printf("%d %d %d\n",x,y,b); edge_count[b]++; to[ edge_count[b] ][b]=y; next[ edge_count[b] ][b]=first[x][b]; first[x][b]=edge_count[b]; } int n,m,ans=0; inline void read(int &a){ a=0;int b=1;char x=getchar(); while(x<‘0‘||‘9‘<x){ if(x==‘-‘)b=-1; x=getchar(); } while(‘0‘<=x&&x<=‘9‘){ a=(a<<3)+(a<<1)+x-‘0‘; x=getchar(); } a*=b; } bool vis[maxn]; int dis[maxn][2],queue[maxm]; inline void spfa(int s,int b){ memset(vis,0,sizeof(vis)); if(b==0)for(int i=1;i<=n;i++)dis[i][0]=1e8;//min else for(int i=1;i<=n;i++)dis[i][1]=-1e8; //max dis[s][b]=w[s]; vis[s]=1; int front=1,rear=0; queue[rear]=s; while(rear<front){ int pos=queue[rear]; //if(b)printf("%d:\n",pos); for(int i=first[pos][b];i;i=next[i][b]){ int v=to[i][b]; // printf("%d ",v); if(b==0){ int t=min(dis[pos][b],w[ v ]); if(t<dis[ v ][0]){ dis[ v ][0]=t; if(!vis[ v ]){ vis[ v ]=1; queue[front]=v;front++; } } } else{ int t=max(dis[pos][1],w[ v ]); //printf("%d %d ",pos,t); if(t>dis[ v ][1]){ dis[ v ][1]=t; if(!vis[ v ]){ vis[ v ]=1; queue[front]=v;front++; } } } } vis[ pos ]=0; rear++; }//老子发誓以后缩进一定好好打,要不然debug非常傻逼 } int main() { read(n);read(m); for(int i=1;i<=n;i++){ read(w[i]);//printf("%d ",w[i]); } for(int i=1,x,y,c;i<=m;i++){ read(x);read(y);read(c); add(x,y,0);//正向 add(y,x,1);//反向 if(c==2){ add(y,x,0); add(x,y,1); } } spfa(1,0); spfa(n,1); for(int i=1;i<=n;i++){ ans=max(ans,dis[i][1]-dis[i][0]); //printf("%d %d %d\n",i,dis[i][0],dis[i][1]); } printf("%d",ans); return 0; }
标签:卖出 memset cst clu eof class style 双向 deb
原文地址:https://www.cnblogs.com/a-blog-of-taojiayi-2003/p/10714280.html