标签:inline void http getchar 统计 getch 计数 turn 直接
好题!一种以前没怎么见过的思路!
以什么方式,什么位置统计本质不同的方案,才能不重不漏是处理所有计数问题的主心骨。
本题难以容斥。难以DP。
所以就尝试挖掘性质,考虑过程!
首先,红色什么时候都可以选,因为可以选择1
不妨给t定一个位置,先充分利用t,再用s,(如果s先用上了,那么t肯定就没意义了)
考虑每个方案是怎样构造出来的,归到合适的t的位置统计。
不妨直接按照“先拿了x个红的,再拿一个蓝的”为标准统计!
为了能涵盖所有之后的决策,让t位于A-(x-1)位置一定最优!让t拿走这个第一个蓝色
首先t肯定是[1,A+1]的
枚举这个位置t,拿走之后,剩下t-1个红色,B-1个蓝色球
现在,之后的连续B-1个球,红色和蓝色可以任意拿!
枚举拿i个红色,贡献C(B-1,i),剩下t-1-i个红色,i个蓝色
t已经废了。
考虑s放在哪里
同样的,枚举“再拿了y个红色,再拿一个蓝色”,
枚举这个位置s,拿走之后,剩下s-1个红色,i-1个蓝色
现在,之后的连续i-1个球,红色和蓝色可以任意拿!
枚举拿j个红色,贡献C(i-1,j),剩下若干红色,若干蓝色
s也废了
直接一口气拿完即可。
由于s和t的位置不能再优秀了,每种方案都一定会考虑到!
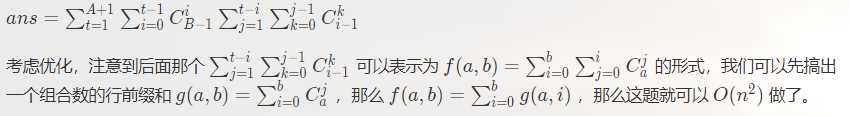
#include<bits/stdc++.h> #define reg register int #define il inline #define fi first #define se second #define mk(a,b) make_pair(a,b) #define numb (ch^‘0‘) #define pb push_back #define solid const auto & #define enter cout<<endl #define pii pair<int,int> using namespace std; typedef long long ll; template<class T>il void rd(T &x){ char ch;x=0;bool fl=false;while(!isdigit(ch=getchar()))(ch==‘-‘)&&(fl=true); for(x=numb;isdigit(ch=getchar());x=x*10+numb);(fl==true)&&(x=-x);} template<class T>il void output(T x){if(x/10)output(x/10);putchar(x%10+‘0‘);} template<class T>il void ot(T x){if(x<0) putchar(‘-‘),x=-x;output(x);putchar(‘ ‘);} template<class T>il void prt(T a[],int st,int nd){for(reg i=st;i<=nd;++i) ot(a[i]);putchar(‘\n‘);} namespace Modulo{ const int mod=1e9+7; int ad(int x,int y){return (x+y)>=mod?x+y-mod:x+y;} void inc(int &x,int y){x=ad(x,y);} int mul(int x,int y){return (ll)x*y%mod;} void inc2(int &x,int y){x=mul(x,y);} int qm(int x,int y=mod-2){int ret=1;while(y){if(y&1) ret=mul(x,ret);x=mul(x,x);y>>=1;}return ret;} } using namespace Modulo; namespace Miracle{ const int N=2003; int A,B; int f[N][N]; int C[N][N]; int main(){ rd(A);rd(B); C[0][0]=1; int lim=max(A,B)+1; for(reg i=1;i<=lim;++i){ C[i][0]=1; for(reg j=1;j<=i;++j){ C[i][j]=ad(C[i-1][j],C[i-1][j-1]); } } for(reg i=1;i<=lim;++i){ for(reg s=1;s<=lim;++s){ f[i][s]=ad(f[i][s-1],C[i-1][s-1]); } for(reg s=1;s<=lim;++s) f[i][s]=ad(f[i][s],f[i][s-1]); } int ans=0; for(reg t=1;t<=A+1;++t){ for(reg i=0;i<=t-1;++i){ if(i!=0) ans=ad(ans,mul(C[B-1][i],f[i][t-i])); else ans=ad(ans,1); } } ot(ans); return 0; } } signed main(){ Miracle::main(); return 0; } /* Author: *Miracle* */
emm,首先发现红色随意拿,s,t为拿蓝色而生!先用t再用s,所以自然就考虑到第一个蓝色在哪里。所以考虑到t放在最优位置上更好。然后任意拿,s同上。
式子的化简就很暴力了其实。
有的时候一些计数题,不妨先枚举最前面一部分的构成,尽量早或者尽量优地进行一些决策来不重不漏涵盖情况,所谓字典序最小的位置统计
标签:inline void http getchar 统计 getch 计数 turn 直接
原文地址:https://www.cnblogs.com/Miracevin/p/10963431.html