LG3389 【模板】高斯消元法 高斯消元

标签：continue   tin   puts   turn   ace   span   其他   void   max   

问题描述

LG3389

题解

高斯消元，是用来解\(n\)元一次方程组的算法，时间复杂度\(O(n^3)\)

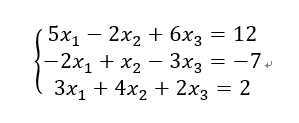
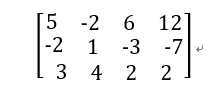
这样就构造出了这个方程组的矩阵

目标就是把这个矩阵左边\(n \times n\)消为单位矩阵

\(\mathrm{Code}\)

#include<bits/stdc++.h>
using namespace std;

void read(int &x){
    x=0;char ch=1;int fh;
    while(ch!='-'&&(ch<'0'||ch>'9')) ch=getchar();
    if(ch=='-') fh=-1,ch=getchar();
    else fh=1;
    while(ch>='0'&&ch<='9'){
        x=(x<<1)+(x<<3)+ch-'0';
        ch=getchar();
    }
    x*=fh;
}

#define maxn 107

int n;

double a[maxn][maxn];

int pla;

int main(){
    ios::sync_with_stdio(0);
    cin>>n;
    for(register int i=1;i<=n;i++){
        for(register int j=1;j<=n+1;j++) cin>>a[i][j];
    }
    for(register int i=1;i<=n;i++){
        pla=i;
        while(pla<=n&&a[pla][i]==0) pla++;
        if(pla==n+1){//如果第i列没有非0的，显然无解
            puts("No Solution");return 0;
        }
        for(register int j=1;j<=n+1;j++) swap(a[i][j],a[pla][j]);//交换到第i行
        double tmp=a[i][i];
        for(register int j=1;j<=n+1;j++) a[i][j]=a[i][j]/tmp;//消除第i行
        for(register int j=1;j<=n;j++){
            if(i==j) continue;
            double rp=a[j][i];
            for(register int k=1;k<=n+1;k++){
                a[j][k]=a[j][k]-rp*a[i][k];//消除其他
            }
        }
    }
    for(register int i=1;i<=n;i++){
        cout<<fixed<<setprecision(2)<<a[i][n+1]<<endl;
    }
    return 0;
}

LG3389 【模板】高斯消元法 高斯消元

标签：continue   tin   puts   turn   ace   span   其他   void   max   

原文地址：https://www.cnblogs.com/liubainian/p/11483289.html