标签：arc   https   struct   new   alt   扫描   NPU   str   false   

POJ2488

题目

　　骑士按照下图所示的走法对棋盘进行巡逻，每个格子只允许巡逻一次，且必须巡逻所有格子。给定棋盘的行数p和列数q，输出一条骑士巡逻路径，若不存在这样一条路径，则输出impossible。

Sample Input

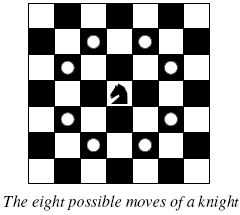
3
1 1
2 3
4 3

Sample Output

Scenario #1:
A1

Scenario #2:
impossible

Scenario #3:
A1B3C1A2B4C2A3B1C3A4B2C4

算法思路

　　深度优先搜索（Depth-First Search，DFS）算法，伪代码如下\(^{[2]}\)：

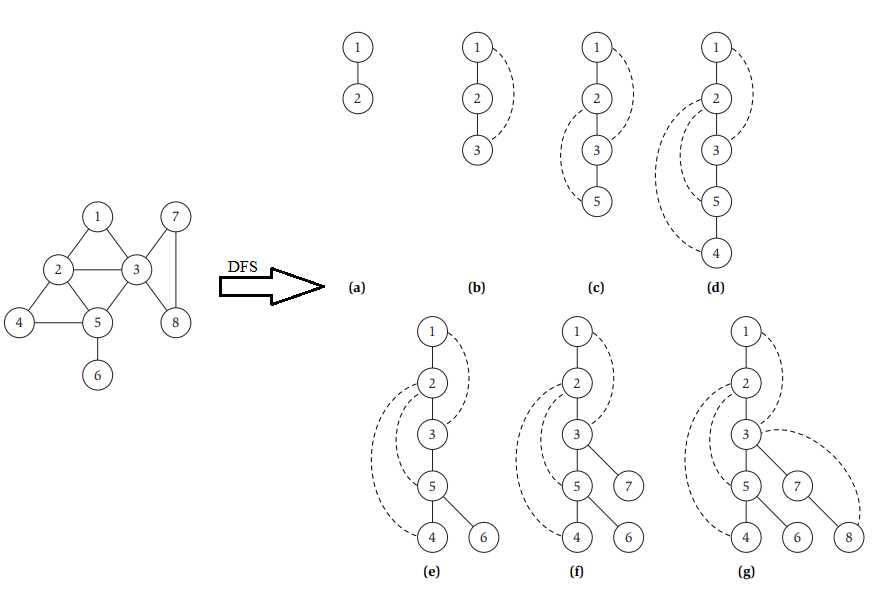
　　1个WA点：若存在多条巡逻路径，题目要求输出字典序优先的路径，lexicographically first path。也就是说，假如A1B1C1和A1C1B1都是允许的路径，则选择A1B1C1，因为B在C前面。其实这一点挺好实现，只需要从左往右扫描“列”即可保证字典序优先。

代码

Result: 372kB, 0ms.

#include <stdio.h>
#include <string.h>
#include <vector>

int n, p, q;
int delta_row[8] = { -1, 1, -2, 2, -2, 2, -1, 1 };//DFS的搜索顺序，按照列从左往右的顺序进行搜索。
int delta_col[8] = { -2, -2, -1, -1, 1, 1, 2, 2 };//即搜索的优先级(-1, -2)=(1, -2)>(-2, -1)=(2, -1)>(-2, 1)=(2, 1)>(-1, 2)=(1, 2)，等于的意思是先搜索哪一个不影响字典序
int explored[30][30];//标记是否为已搜索过的节点
int cnt;
struct Node {
    int row, col;
} path[30];//记录下路径

void Init() {
    memset(explored, 0, sizeof(explored));
    cnt = 0;
}

bool Dfs(int row, int col) {
    explored[row][col] = 1;//标记为已搜索的节点
    cnt++;//深度加1
    path[cnt].row = row;
    path[cnt].col = col;
    for (int i = 0; i < 8; i++) {//按照列从左往右的顺序进行搜索
        int row_new = row + delta_row[i], col_new = col + delta_col[i];
        if (row_new >= 1 && row_new <= p && col_new >= 1 && col_new <= q && !explored[row_new][col_new]) {//骑士要去巡逻的新节点(row_new, col_new)在棋盘范围内且未搜索过
            if (Dfs(row_new, col_new))//若找到路径，直接break，一层层跳出递归，若没找到，对下一个优先级的要去巡逻的新节点进行搜索
                break;
        }
    }
    if (cnt == p * q)//若深度为p*q，说明我们找到了路径
        return true;
    else {//若没找到，回溯到前一节点，当前节点置为未搜索的节点，树的深度减1
        explored[row][col] = 0;
        cnt--;
        return false;
    }
}

int main() {
    scanf("%d", &n);
    for (int t = 1; t <= n; t++) {
        scanf("%d %d", &p, &q);
        printf("Scenario #%d:\n", t);
        Init();
        if (Dfs(1, 1)) {
            for (int i = 1; i <= p * q; i++)
                printf("%c%c", 'A' + path[i].col - 1, '1' + path[i].row - 1);
            printf("\n");
        }
        else
            printf("impossible\n");
        if (t != n)
            printf("\n");
    }
    return 0;
}

参考：

[1] Knight‘s tour
[2] 算法设计

POJ2488 深度优先搜索+回溯

标签：arc   https   struct   new   alt   扫描   NPU   str   false   

原文地址：https://www.cnblogs.com/wtyuan/p/12106652.html