标签:ble product tin || eof main sync memset names
题意:
你有三个姐妹她们分别有 a , b , c枚硬币,你有n枚,你可以把硬币随意分给她们(必须分完),使她们的硬币数A = B = C
分析:
题目的条件有两点:
①A = B = C
②在满足①的前提下必须把硬币分完
我们首先要满足第一点。因为硬币个数有限,我们要尽可能用最少的硬币使 A = B = C,所以只要让a,b,c中小的两个等于最大的即可
那么我们剩下的硬币数就为 $n-\left( \max -a\right) -\left( \max -b\right) -\left( \max -c\right)$ 判断剩余数是否大于0即可(足够分配)
对于第二个条件,只要判断我们剩下的硬币是否是三的倍数即可(只有为三倍数才可以等量均分保持A = B = C)

#include<bits/stdc++.h> using namespace std; int main() { int t; cin >> t; while(t --) { int a , b , c , n; cin >> a >> b >> c >> n; int MAX = max(a , max(b , c)); n -= (MAX - a) + (MAX - b) + (MAX - c); if(n < 0 || n % 3 != 0) cout << "NO" << ‘\n‘; else cout << "YES" << ‘\n‘; } return 0; }
题意:
有n个盒子需要你捡,第i个盒子的坐标为 (Xi , Yi)。你从(0,0)出发,每次只能选择向上或者向右移动,问能否将n个盒子都捡完,若可以捡完,则输出字典序最小的一条路线
分析:
我们将n个盒子的坐标先按照x值再按照y值排序。因为是按照 X 升序排序的,所以当 Yi > Yi + 1 时,很显然此时第i个盒子和第i+1个盒子有一个不能走到
而 Yi <= Yi+1 时,为了保证字典序最小,我们先加上(Xi+1 - Xi)个L, 再加上(Yi+1 - Yi)个纵坐标即可

#include<bits/stdc++.h> using namespace std; #define fi first #define se second const int N = 2e5 + 10; pair<int , int> ha[N]; int main() { int t; cin >> t; while(t --) { int n , flag = 1 ; string ans = ""; cin >> n; for(int i = 1 ; i <= n ; i ++) cin >> ha[i].fi >> ha[i].se; ha[0].fi = 0 , ha[0].se = 0; sort(ha , ha + 1 + n); for(int i = 0 ; i < n ; i ++) { if(ha[i].se > ha[i + 1].se) { flag = 0 ; break; } int dis1 = ha[i + 1].fi - ha[i].fi; int dis2 = ha[i + 1].se - ha[i].se; while(dis1) ans += "R" , dis1 --; while(dis2) ans += ‘U‘ , dis2 --; } if(flag) cout << "YES" << ‘\n‘ << ans << ‘\n‘; else cout << "NO" << ‘\n‘; } return 0; }
题意:
给你一个 n ,要求三个整数 a ,b ,c 使得 a * b * c = n 并且 a、b、c >= 2
分析:
先枚举 n 的因子,再判断因子是否可以再分解即可

#include<bits/stdc++.h> using namespace std; int main() { int t; cin >> t; while(t --) { int n; cin >> n; int ans1 , ans2 , ans3; int flag = 0; for(int i = 2 ; i * i <= n ; i ++) { if(n % i == 0) { for(int j = 2 ; j * j < i ; j ++) { if(i % j) continue; ans1 = n / i , ans2 = j , ans3 = i / j; flag = 1 ; break; } int now = n / i; for(int j = 2 ; j * j < now ; j ++) { if(now % j || now / j == i || j == i) continue; ans1 = i , ans2 = j , ans3 = now / j; flag = 1 ; break; } } } if(!flag) cout << "NO" << ‘\n‘; else cout << "YES" << ‘\n‘ << ans1 << " " << ans2 << " " << ans3 << ‘\n‘; } return 0; }
题意:
给你 q 个询问和 一个 x , 每次询问输入一个数 n ,你可以把它减任意次 x 或 加任意次 x,然后添入数组,问每次询问结束时数组里最小的没出现的非负整数是多少
分析:
我们可以想象有若干个长度为 x 的区间[0 , x - 1] ,那么每次询问的数 n 只会出现在某个区间的 n % x位置上。为了满足题目要求,我们要尽可能让它填在比较靠前的区间里
cnt [i] 表示此次询问时,若干个区间一共有cnt[i] 个 i 可以填到若干个区间中的 i 位置上(为了满足题目要求,我们从第一个区间的第i个位置开始填,然后再填第二个区间第i个位置)
然后我们从第一个区间开始检查。若到当前位置时cnt[i] != 0,则我们让cnt[i] --(表示我们拿一个i填在这个位置上),同时往下一个位置跳,直到遇到一个没有数可填的位置——cnt[pos] = 0

#include<bits/stdc++.h> using namespace std; map<int , int>cnt; int main() { int q , x , ans = 0; cin >> q >> x; while(q --) { int n; cin >> n; cnt[n % x] ++; while(cnt[ans % x]) cnt[ans % x] -- , ans ++; cout << ans << ‘\n‘; } return 0; }
题意:
给你一个 n * m 的矩阵,你执行两种操作:
① 把矩阵中任意一个元素改为任意一个数
② 把矩阵中任意一列整体往上挪一个单元格,如右图矩阵我们对第一列向上挪了一个单元格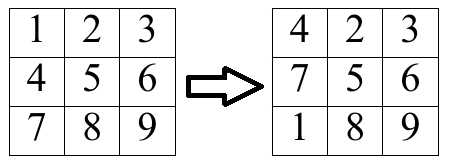
要求用最少的操作次数使矩阵 a[i][j] = (i - 1) * m + j
分析:
因为题目只能对一列或者一个元素进行操作,所以我们逐列进行维护。
对第i行第j列的元素a[i][j] 我们假设它将成为这列的起点(第一个元素) 那么最坏的操作次数cost[i]为 i + N (把它移动到第1位需要i次 如果元素全都很奇葩需要更改N次)
对于每一列的操作,我们先初始化cost[i] = i + N , 然后如果a[i][j]可以作为第h行的答案的答案,那么cost[h] --(把a[h][j]行设为起点的最坏操作- 1)
最后遍历cost[1] ~ cost[n] 挑选最小的cost加到ans里即可

#include<bits/stdc++.h> using namespace std; const int N = 2e5 + 10; int main() { int n , m ; cin >> n >> m; vector<vector<int>>a(n , vector<int>(m)); for(int i = 0 ; i < n ; i ++) for(int j = 0 ; j < m ; j ++) { cin >> a[i][j]; a[i][j] --; } int ans = 0; for(int j = 0 ; j < m ; j ++) { vector<int>cost(n); for(int i = 0 ; i < n ; i ++) cost[i] = i + n; for(int i = 0 ; i < n ; i ++) { if(a[i][j] % m == j && a[i][j] < n * m) { int h = i - a[i][j] / m; if(h < 0) h += n; cost[h] --; } } ans += *min_element(cost.begin() , cost.end()); } cout << ans << ‘\n‘; return 0; }
题意:
给你一棵树,要求你找出任意三点 A,B,C,使得 A~B,B~C,A~C 之间的边最多(边并集最大)
分析:
比赛最后几分钟做出来了,然而一时马虎,提交到E题上去了。然后评测机也不给力,等赛后几分钟我才知道交错题了。再放到F题上提交,一发就过了。。。
可证明一组最优解中一定有两个点是直径的两端点,那么题目就转换成求树直径端点及与两端点边并集最大的点,于是就很简单了
我们先一次bfs求出树直径DIS即其一端点A,再对端点A进行bfs求出另一端点B及每个点到端点A的距离dis1[i],最后再bfs端点B求出每个点到B的距离dis2[i]
最后遍历每个点,取边并集最大的即可(边并集= $\dfrac {dis1\left[ i\right] +dis2\left[ i\right] -DIS}{2}+DIS$)
现在给出证明:
假设某个答案取连接点x。x最远的树到达的点是s,根据树的直径算法,s是树的某个直径a的端点。假设x的最远和第二远的点组成的链是b,b就会和a有一段公共部分。我们取a和b相交部分距离s最远的那个点y。那么取这个链上点y的答案一定比x更优

#include<bits/stdc++.h> using namespace std; const int N = 5e5 + 10; struct Edge{ int nex , to , w; }edge[N]; int one , two , DIS; int head[N] , vis[N] , d[N]; int tot = 0; void add(int u , int v , int w) { edge[tot].w = w; edge[tot].to = v; edge[tot].nex = head[u]; head[u] = tot ++; } int bfs(int st) { memset(d , 0 , sizeof(d)); memset(vis , 0 , sizeof(vis)); queue<int>que; que.push(st); vis[st] = 1; int now; while(!que.empty()) { now = que.front() , que.pop(); for(int i = head[now] ; ~i ; i = edge[i].nex) { int TO = edge[i].to; if(vis[TO]) continue; d[TO] = d[now] + edge[i].w; vis[TO] = 1; que.push(TO); if(DIS < d[TO]) DIS = d[TO]; } } return now; } int dis1[N] , dis2[N]; int main() { ios::sync_with_stdio(false); memset(head , -1 , sizeof(head)); int n ; cin >> n; for(int i = 1 ; i < n ; i ++) { int x , y; cin >> x >> y; add(x , y , 1); add(y , x , 1); } DIS = 0; int one , two , three; one = bfs(1); two = bfs(one); for(int i = 1 ; i <= n ; i ++) dis1[i] = d[i]; bfs(two); for(int i = 1 ; i <= n ; i ++) dis2[i] = d[i]; int ans = 0; for(int i = 1 ; i <= n ; i ++) { if((dis1[i] + dis2[i] - DIS) / 2 + DIS > ans && i != one && i != two) ans = (dis1[i] + dis2[i] - DIS) / 2 + DIS , three = i; } cout << ans << ‘\n‘; cout << one << " " << two << " " << three << ‘\n‘; return 0; }
为了更好的供人观看,每一份代码我都是重新手写的,请多支持
Codeforces Round #615 (Div. 3)
标签:ble product tin || eof main sync memset names
原文地址:https://www.cnblogs.com/StarRoadTang/p/12230408.html