标签:find lock print ima 实现 short stat 循环 最短路径
本算法可以算是贪心算法的实现,通过从起点出发去寻找与其他点的最短距离,找到该最短距离的顶点,然后里该顶点为中转点,去寻找
以中转点出发的到其他顶点更近的距离,从而通过一步步的选择找到最优的路径!
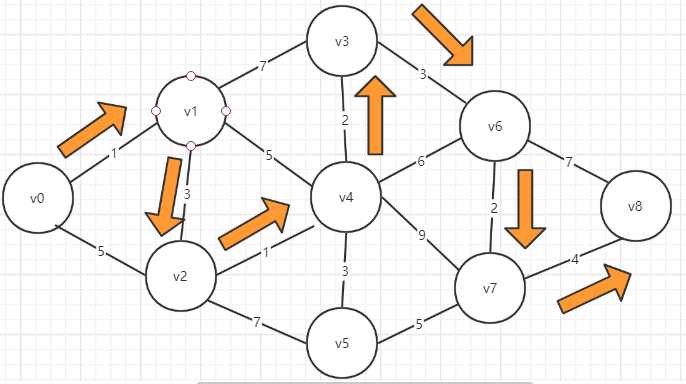
流程如上图所示,v0到其他顶点的距离设置成一个数组path[0,1,5,65535,65535,65535,65535,65535,65535]其中,65535代v0待这个点之间距离无穷大。首先在path中找到最近的一个顶点为v1,然后我们以v1为中转点来更新该数组,怎么更新尼?加入以v0过v1然后达到其他顶点的路径小于v0直接到达其他顶点的路径,则直接用该较小路径来替代数组中数值。依次不断循环来去找到v0到v2,到v3,到v4,到v5,到v6,到v7,到v8的距离。就可以了。
package nearstWay; import sun.security.util.Length; /** * @author :dazhu * @date :Created in 2020/3/18 11:37 * @description:最短路径 * @modified By: * @version: $ */ public class Main { public static void main(String[] args){ //制作顶点数组,邻接矩阵 int inf = 65535; Vertex[] vertexList = new Vertex[]{ new Vertex("v0"), new Vertex("v1"), new Vertex("v2"), new Vertex("v3"), new Vertex("v4"), new Vertex("v5"), new Vertex("v6"), new Vertex("v7"), new Vertex("v8") }; int[][] adjMat = new int[][]{ { 0, 1, 5,inf,inf,inf,inf,inf,inf}, { 1, 0, 3, 7, 5,inf,inf,inf,inf}, { 5, 3, 0,inf, 1, 7,inf,inf,inf}, {inf, 7,inf, 0, 2,inf, 3,inf,inf}, {inf, 5, 1, 2, 0, 3, 6, 9,inf}, {inf,inf, 7,inf, 3, 0,inf, 5,inf}, {inf,inf,inf, 3, 6,inf, 0, 2, 7}, {inf,inf,inf,inf, 9, 5, 2, 0, 4}, {inf,inf,inf,inf,inf,inf, 7, 4, 0}, }; Graph g = new Graph(vertexList,adjMat); Dijkstra djk = new Dijkstra(g); djk.findNearestWay(0); } } class Dijkstra{ public Graph g = null; public int nVertex; public int[] pathMatirx;//用于存储最短路径下表的数组 public int[] shortPathTable;//用于存储个点最短路径的权重值 public int[] markArr;//markArr[k] = 1代表求得v0到vk的最短路径了 public Dijkstra(Graph g){ this.g = g; this.nVertex = g.vertexList.length; //根据输出的graph顶点数量来初始化数组 this.pathMatirx =new int[nVertex]; this.shortPathTable = new int[nVertex]; this.markArr = new int[nVertex]; } public void findNearestWay(int v0){ int v=0,w=0,k=0; int min; //初始化数据 for(int i=0;i<nVertex;i++){ this.markArr[i] = 0; //初始化shotPathTable为v0到其他顶点的距离 this.shortPathTable[i] = g.adjMat[v0][i]; //初始化路径数组为0 this.pathMatirx[i] = 0; } //代表v0到v0之间不需要求路径!! this.markArr[0] = 1; //开始主循环,每次循环求的v0到某个顶点v之间的最短路径,直到所有顶点都求的了!!! for(v=1;v<nVertex;v++){ min = 65535;//当前所知离v0最近的距离,初始化成适当大的值 //寻找里v0最近的顶点 for(w =0;w<nVertex;w++) { //当且仅当该点没有被寻找最短路径且路径小于min下 if ((markArr[w]==0)&&shortPathTable[w]<min){ //保存最短路径中的顶点于k k = w; //将min替换为该最短路径 min = shortPathTable[w]; } } //将目前找到的最近顶点置为1,代表着v0到vk已经找到了最短路径 this.markArr[k] = 1; //修正当前最短路径及距离!! //如果v0经过前面求得vk到达其他顶点的距离,小于v0直接道道其他顶点的距离 //则更新v0的shortPathTable[]为最短距离 for(w =0;w<nVertex;w++){ if(markArr[w]==0&&min+g.adjMat[k][w]<shortPathTable[w]){ shortPathTable[w] = min+g.adjMat[k][w]; pathMatirx[w] = k; } } } } } class Graph{ public Vertex[] vertexList; public int adjMat[][]; public int nVerts; public Graph(Vertex[] vertexList,int[][] adjMat){ //初始化,顶点数组 this.vertexList = vertexList; //创建边矩阵 this.adjMat = adjMat; //初始顶点顺序 this.nVerts = 0; } //添加顶点到顶点数组 public void addVertex(String lab){ vertexList[nVerts++] = new Vertex(lab); } //添加边到边矩阵 //weight 0:权值为0的相连 //weight k:权值为k的相连 //weight 无穷大:不相连 public void addEdge(int start,int end,int weight){ adjMat[start][end] = weight; adjMat[end][start] = weight; } //显示所有顶点 public void showVertexs(){ for(int i=0;i<vertexList.length;i++){ System.out.println(vertexList[i]); } } //打印指定顶点 public void printVertex(int v){ System.out.println(vertexList[v].label); } } //顶点类的结构 //顶点可以存在数组中,用index来表示, //当然也可以存在链表中 class Vertex{ public String label;//label public boolean wasVisited;//是否遍历过的标记。 public Vertex(String label){ this.label = label; wasVisited = false; } }
标签:find lock print ima 实现 short stat 循环 最短路径
原文地址:https://www.cnblogs.com/dazhu123/p/12519611.html