标签：color 有趣的 编号 一句话 否则 描述 路径 技术 span
Description
Input
Output
Sample Input
100 7 1 101 1 2 1 2 2 2 3 2 3 3 1 1 3 2 3 1 1 5 5
Sample Output
3
思路：
这道题为经典的带权并查集例题， 动物间的相对关系有三种可能：同类， 吃， 被吃
于是可以这样创建一个数组 relation[]，同时构造数组pre[]， pre[i]表示i的父节点。 relation[i]=0表示 i 与 pre[i] 同类， =1表示 i 吃 pre[i] ， =2表示 pre[i] 吃 i。
关于路径压缩：(find函数)， 我们可以发现权值（关系对应的值） 并不是直接累加， 但找规律后可以发现 A->C = (A->B + B->C) % 3，因此关系值的更新需要累加再模3。 find函数如下：
1 int find(int x) { 2 if (x != pre[x]) { 3 int px = find(pre[x]); 4 relation[x] = (relation[x] + relation[pre[x]]) % 3; 5 pre[x] = px; 6 } 7 return pre[x]; 8 }
至于合并过程， 从上面的规律可知， 只是在一般带权并查集的合并基础上取模
一般带权并查集的合并思路：
------>
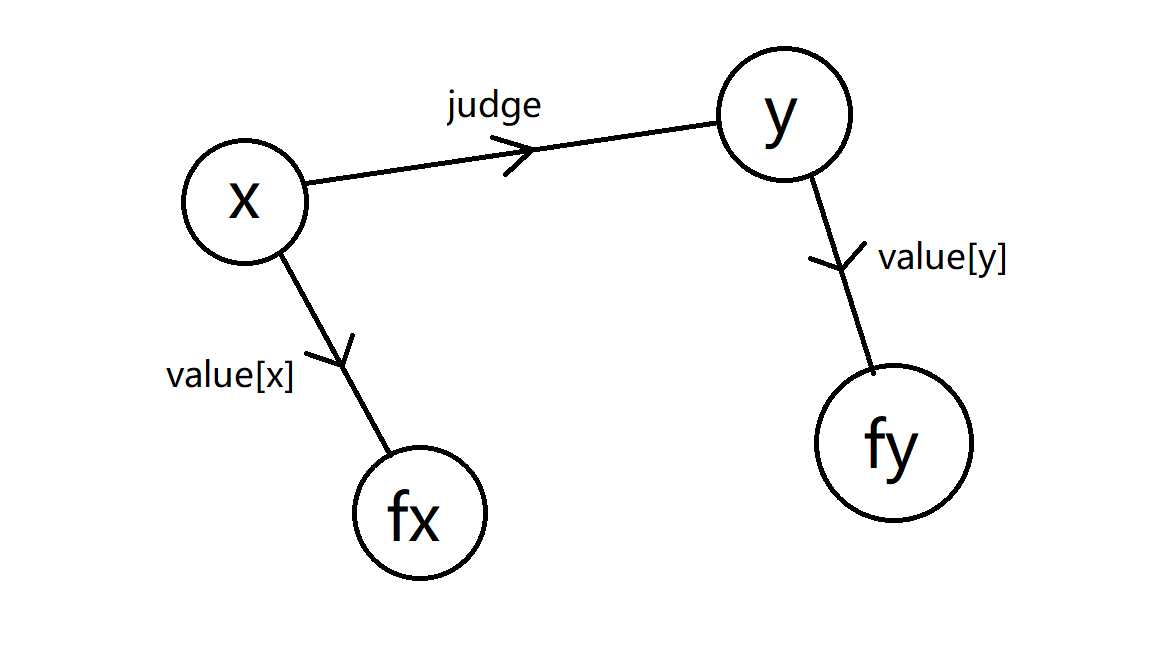1 if (fx != fy) { 2 pre[fx] = fy; 3 value[fx] = judge + value[y] - value[x] 4 }
总体上， c语言代码如下：
1 #include <stdio.h> 2 #include <string.h> 3 #define maxn 50010 4 int relation[maxn], pre[maxn]; 5 // relation[i] = 0: i与pre[i]为同类 1: i吃pre[i] 2: pre[i]吃i 6 7 void init() { 8 for (int i = 0; i < maxn; i++) { 9 relation[i] = 0; 10 pre[i] = i; 11 } 12 } 13 14 int find(int x) { 15 if (x != pre[x]) { 16 int px = find(pre[x]); 17 relation[x] = (relation[x] + relation[pre[x]]) % 3; 18 pre[x] = px; 19 } 20 return pre[x]; 21 } 22 23 int jion(int x, int y, int judge) { 24 int fx = find(x); 25 int fy = find(y); 26 if (fx == fy) { 27 if ((relation[x] - relation[y] + 3) % 3 != judge) return 1; 28 else return 0; 29 } 30 else { 31 pre[fx] = fy; 32 relation[fx] = (relation[y] + judge - relation[x] + 3) % 3; 33 } 34 return 0; 35 } 36 37 int main() { 38 int N, K, x, y, judge, ans = 0; 39 scanf("%d%d", &N, &K); 40 init(); 41 for (int i = 0; i < K; i++) { 42 scanf("%d%d%d", &judge, &x, &y); 43 if (x > N || y > N || (x == y && judge == 2)) { 44 ans++; 45 continue; 46 } 47 if (jion(x, y, judge-1)) { 48 ans++; 49 } 50 } 51 printf("%d\n", ans); 52 return 0; 53 }
标签：color 有趣的 编号 一句话 否则 描述 路径 技术 span
原文地址：https://www.cnblogs.com/y2ek/p/12654234.html
