码迷，mamicode.com
首页 > 其他好文 > 详细

$CF\ 634\ (Div3)$

时间：2020-04-15 01:01:37      阅读：84      评论：0      收藏：0      [点我收藏+]

标签：span   贪吃蛇   replace   位置   顺序   二分   sudoku   abc   不能   

\(CF\ 634\ (Div3)\)

\(A.\)

给定 \(n\)，问有多少对正整数 \(a,\ b\)，使得 \(a + b = n\) 且 \(a > b\)

如果 \(n\) 是偶数，那么 \(ans = n / 2 - 1\)

如果 \(n\) 是奇数，那么 \(ans = n / 2\)

#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 1e5 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;
 
 
inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}
 
int t, n;;
int main()
{
    t = read();
    while(t--) {
        n = read();
        int ans = 0;
        if(n % 2) ans = n / 2;
        else ans = n / 2 - 1;
        printf("%d\n", ans);
    }
    return 0;
}

\(B.\)

构造一个长度为 \(n\) 的字符串，使得每一个长为 \(a\) 的子串都只含有 \(b\) 个不同的字符

构造方式如下

先按照顺序填上前 \(b\) 个小写字母，剩下的 \(a - b\) 个位置填第 \(b\) 个字符

将这样一个子串 \(s\) 填满整个长为 \(n\) 的字符串即可

例如样例 \(7\ 5\ 3\)

构造 \(s = abccc\)，答案即为 \(abcccab\)

感性理解，就是一个贪吃蛇在子串 \(s\) 上移动，\(s\) 的正确性构造保证了整个字符串的正确性...

#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 1e5 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;
 
 
inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}
 
int t, a, b, c;
int main()
{
    t = read();
    while(t--) {
        a = read(), b = read(), c = read();
        string s = "";
        for(int i = 0; i < c; ++i)
            s += ‘a‘ + i;
        for(int i = 0; i < b - c; ++i)
            s += ‘a‘ + c - 1;
        for(int i = 0; i < a / b; ++i)
            cout<<s;
        for(int i = 0; i < a % b; ++i)
            cout<<s[i];
        cout<<endl;
    }
    return 0;
}

\(C.\)

给定一组数 \(a\)，要求将其划分为两个长度一样的集合 \(s,\ t\)，\(s\) 中每个数都一样，\(t\) 中每个数都不一样，\(t\) 中的数也可以出现在 \(s\) 中，求最大的可能的长度

一开始的想法是二分长度的答案，用 \(map\) 记录每个数出现的次数，然后去 \(check\)

后来 \(get\) 了一个比较好的想法

用 \(x\) 表示 \(a\) 中 \(distinct\) 的值的数量

用 \(y\) 表示 \(a\) 中出现次数最多的值的出现次数

\(if\ x = y,\ ans = x - 1\)
\(else,\ ans = min(x,\ y)\)

/*二分答案*/
#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 2e5 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;
 
inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}
 
int t, n, a[N];
map<int, int> mp;
 
bool check(int x)
{
    int f1 = 0, f2 = 0;
    set<int> st;
    for(int i = 1; i <= n; ++i)
        st.insert(a[i]);
    for(int i = 1; i <= n; ++i) {
        if(mp[a[i]] >= x) {
            if(mp[a[i]] == x) st.erase(a[i]);
            if(st.size() >= x) return 1;
            st.insert(a[i]);
        }
    }
    return 0;
}
int main()
{
    t = read();
    while(t--) {
        mp.clear();
        n = read();
        for(int i = 1; i <= n; ++i) a[i] = read(), mp[a[i]]++;
        int l = 0, r = n / 2;
        int ans = 0;
        while(l <= r) {
            int mid = l + r >> 1;
            //cout<<l<<‘ ‘<<r<<endl;
            if(check(mid)) ans = mid, l = mid + 1;
            else r = mid - 1;
        }
        printf("%d\n", ans);
    }
    return 0;
}
/*
4
7
4 2 4 1 4 3 4
5
2 1 5 4 3
1
1
4
1 1 1 3
*/

/*good idea*/
#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 2e5 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;


inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}

int t, n;
map<int, int> mp;

int main()
{
    t = read();
    while(t--) {
        mp.clear();
        n = read();
        int x = 0;
        for(int i = 1; i <= n; ++i) {
            int y = read();
            mp[y]++;
            x = max(x, mp[y]);
        }
        int ans = 0;
        if(mp.size() == x) ans = x - 1;
        else ans = min((int)mp.size(), x);
        printf("%d\n", ans);
    }
    return 0;
}
/*
4
7
4 2 4 1 4 3 4
5
2 1 5 4 3
1
1
4
1 1 1 3
*/

\(D.\)

给定一个正确的数独，允许至多修改 \(9\) 个数字，使得其成为 \(Anti-Sudoku\)

数独满足

  • 每一行不重数
  • 每一列不重数字
  • 每一个 \(3\times 3\) 的正方形不重数字

技术图片

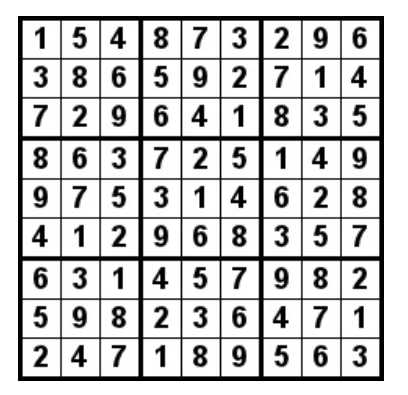
\(Anti-Sudoku\) 满足

  • 每一行至少有两个位置重复
  • 每一列至少有两个位置重复
  • 每一个 \(3\times 3\) 的正方形至少有两个位置重复

观察发现，每个正方形内都要修改一个位置，并且这些位置所在的行、列不能重复，可以用类似于 \(N\) 皇后的搜索方法来写

后来 \(get\) 到一个很好的 \(idea\)，既然给定的是正确的数独，那么我们只需要将任意一个数换成另一个数字就可以了，例如将所有的 \(3\) 换成 \(1\)

/*dfs*/
#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 2e5 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;
int t, n, vis[20][20];
char g[20][20];
int f = 0, cnt;
const int dx[] = {0, 1, 1, 1, 2, 2, 2, 3, 3, 3};
const int dy[] = {0, 1, 2, 3, 1, 2, 3, 1, 2, 3};
int row[20], col[20];
 
inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}
 
void out()
{
    //puts("XCY");
    for(int i = 1; i <= 9; ++i)
        puts(g[i] + 1);
}
 
bool check()
{
    map<int, int> mp;
    for(int i = 1; i <= 9; ++i) {
        int f = 0;
        for(int j = 1; j <= 9; ++j) {
            mp[g[i][j]]++;
            if(mp[g[i][j]] > 1) {f = 1; break;}
        }
        if(!f) return 0;
        mp.clear();
    }
    for(int i = 1; i <= 9; ++i) {
        int f = 0;
        for(int j = 1; j <= 9; ++j) {
            mp[g[j][i]]++;
            if(mp[g[j][i]] > 1) {f = 1; break;}
        }
        if(!f) return 0;
        mp.clear();
    }
    for(int k = 1; k <= 3; ++k) {
        for(int l = 1; l <= 3; ++l) {
            int f = 0;
            for(int i = (k - 1) * 3 + 1; i <= k * 3; ++i) {
                for(int j = (l - 1) * 3 + 1; j <= l * 3; ++j) {
                    mp[g[i][j]]++;
                    if(mp[g[i][j]] > 1) {f = 1; break;}
                }
                if(f) break;
            }
            mp.clear();
            if(!f) return 0;
        }
    }
    return 1;
}
 
void dfs(int step)
{
    //cout<<step<<endl;
    if(f) return;
    if(step > 9) {
        cnt++;
        if(check()) f = 1, out();
        else return;
    }
    for(int i = 3 * (dx[step] - 1) + 1; i <= 3 * dx[step]; ++i) {
        if(row[i]) continue;
        for(int j = 3 * (dy[step] - 1) + 1; j <= 3 * dy[step]; ++j) {
            if(col[j]) continue;
            for(int k = 1; k <= 9; ++k) {
                if(g[i][j] == k + ‘0‘) continue;
                if(vis[i][j]) continue;
                int temp = g[i][j];
                vis[i][j] = 1;
                g[i][j] = k + ‘0‘;
                row[i] = 1, col[j] = 1;
                dfs(step + 1);
                if(f) return;
                g[i][j] = temp;
                vis[i][j] = 0;
                row[i] = 0, col[j] = 0;
            }
        }
    }
}
int main()
{
    t = read();
    while(t--) {
        memset(vis, 0, sizeof(vis));
        memset(col, 0, sizeof(col));
        memset(row, 0, sizeof(row));
        f = 0;
        for(int i = 1; i <= 9; ++i)
            scanf("%s", g[i] + 1);
        dfs(1);
        //cout<<cnt<<endl;
    }
    return 0;
}
/*
154873296
386592714
729641835
863725149
975314628
412968357
631457982
598236471
247189563
*/

/*replace 3 with 1*/
#include <bits/stdc++.h>
#define fi first
#define se second
#define pii pair<int, int>
#define arrayDebug(a, l, r) for(int i = l; i <= r; ++i) printf("%d%c", a[i], " \n"[i == r])
typedef long long LL;
typedef unsigned long long ULL;
const LL INF = 0x3f3f3f3f3f3f3f3f;
const int inf = 0x3f3f3f3f;
const int DX[] = {0, -1, 0, 1, 0, -1, -1, 1, 1};
const int DY[] = {0, 0, 1, 0, -1, -1, 1, 1, -1};
const int MOD = 1e9 + 7;
const int N = 4e2 + 7;
const double PI = acos(-1);
const double EPS = 1e-6;
using namespace std;
int t, n;
char g[20][20];
 
inline int read()
{
    char c = getchar();
    int ans = 0, f = 1;
    while(!isdigit(c)) {if(c == ‘-‘) f = -1; c = getchar();}
    while(isdigit(c)) {ans = ans * 10 + c - ‘0‘; c = getchar();}
    return ans * f;
}
 
int main()
{
    t = read();
    while(t--) {
        for(int i = 1; i <= 9; ++i)
            scanf("%s", g[i] + 1);
        for(int i = 1; i <= 9; ++i)
            for(int j = 1; j <= 9; ++j)
                if(g[i][j] == ‘3‘) g[i][j] = ‘1‘;
        for(int i = 1; i <= 9; ++i)
            puts(g[i] + 1);
    }
    return 0;
}

$CF\ 634\ (Div3)$

标签：span   贪吃蛇   replace   位置   顺序   二分   sudoku   abc   不能   

原文地址：https://www.cnblogs.com/ChenyangXu/p/12702355.html
踩
(0)
赞
(0)
   
举报
评论 一句话评论（0）
登录后才能评论！
分享档案
更多>
2021年07月29日 (22)
2021年07月28日 (40)
2021年07月27日 (32)
2021年07月26日 (79)
2021年07月23日 (29)
2021年07月22日 (30)
2021年07月21日 (42)
2021年07月20日 (16)
2021年07月19日 (90)
2021年07月16日 (35)
周排行
mamicode.com排行更多图片
更多
友情链接
兰亭集智   国之画   百度统计   站长统计   阿里云   chrome插件   新版天听网
关于我们 - 联系我们 - 留言反馈
© 2014 mamicode.com 版权所有  联系我们:gaon5@hotmail.com
迷上了代码！