水题(water) 【斐波那契数列】

标签：insert   分析   amp   VID   lang   splay   mic   font   博客   

题意：

其中，\(f(1)=1,f(2)=1\)。
传送门

分析：

首先先看斐波那契数列的几何意义：

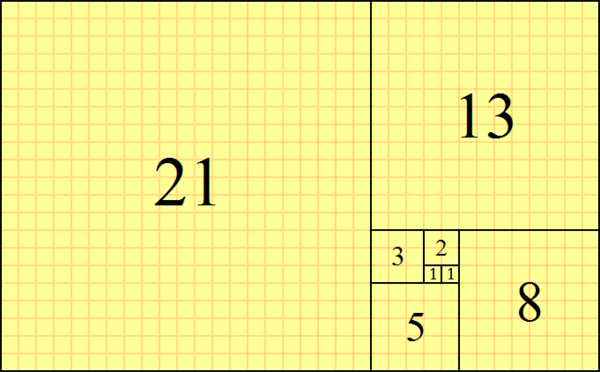
图中各数字为正方形的边长。
可以发现其面积关系刚好满足题目中的等式：

因此 \(f(n)\) 实际上就是斐波那契数列，所以当 \(x\) 是斐波那契数时，求 \(m\) 进制下末尾 \(0\) 的个数。否则，求 \(z\) 皇后数（可以预处理出）。

代码：

#include <bits/stdc++.h>
using namespace std;
typedef long long ll;
int maxn=90;
int a[14]={-1, 1, 0, 0, 2, 10, 4, 40, 92, 352, 724, 2680, 14200, 73712};//n皇后前几项
set<ll>st;
ll num[10],e[10];
void init()
{
    ll a=1,b=1,c;
    st.insert(1LL);
    for(int i=1;i<=maxn;i++)
    {
        c=a+b;//cout<<"c="<<c<<endl;
        a=b;
        b=c;
        st.insert(c);
    }
}
ll divide(ll n)
{
    ll cnt=0;
    for(ll i=2;i*i<=n;i++)
    {
        if(n%i==0)
        {
            num[++cnt]=i;
            e[cnt]=0;
            while(n%i==0)
            {
                e[cnt]++;
                n/=i;
            }
        }
    }
    if(n>1)
    {
        num[++cnt]=n;
        e[cnt]=1;
    }
    return cnt;
}
ll ct(ll x,ll p)
{
    ll cnt=0;
    while(x)
    {
        cnt+=(x/p);
        x/=p;
    }
    return cnt;
}
int main()
{
    ll x,m;
    init();
    scanf("%lld%lld",&x,&m);
    if(st.count(x))//是斐波那契数列
    {
        ll cnt=divide(m);
        ll minn=1e18;//要足够大，小了会WA,注意是求阶乘后面的0的个数
        for(ll i=1;i<=cnt;i++)
        {
            ll t=ct(x,num[i]);
            minn=min(t/e[i],minn);
        }
        printf("%lld\n",minn);
    }
    else
    {
        ll z=(x%min(13*1LL,m))+1;
        printf("%d\n",a[z]);
    }
    return 0;
}

参考博客

水题(water) 【斐波那契数列】

标签：insert   分析   amp   VID   lang   splay   mic   font   博客   

原文地址：https://www.cnblogs.com/1024-xzx/p/12706781.html