标签:inf span 后缀 ima max lazy tac 中缀表达式 不同
1.用栈求中缀表达式的值:
建立2个栈,S1暂存操作数,S2暂存运算符,当遇到操作数则入S1,遇到运算符准备入S2,首先若S2为空或者S2栈顶为‘(‘,则运算符直接入S2栈,若S2不空并且S2栈顶非‘(‘,若当前扫描运算符的运算优先级大于栈顶运算符优先级,则入栈S2,否则对S2不停地执行出栈操作,每出栈一个运算符就同时从S1出栈2个操作数,先出栈的排在右边,后出战的排在左边,然后拿这2个操作数与运算符进行运算并将结果存入S1
int getPriority(char a){ if(a == ‘+‘ || a==‘-‘){ return 0; }else{ return 1; } } int calSub(float opand1,char op,float opand2,float &result){ if(op == ‘+‘) result =opand1+opand2; if(op == ‘-‘) result =opand1-opand2; if(op == ‘*‘) result=opand1*opand2; if(op == ‘/‘){ if(fabs(opand2)<MIN){ return 0; }else{ result=opand1/opand2; } } return 1; } int calStackTopTwo(float s1[],int &top1,char s2[],int &top2){ float opand1,opand2,result; char op; int flag; opand2=s1[top1--]; opand1=s1[top1--]; op=s2[top2--]; flag=calSub(opand1,op,opand2,result); if(flag == 0){ cout<<"ERROR"<<endl; return 0; } s1[++top1]=result; return flag; } float calInfix(char exp[]){ float s1[MaxSize]; int top1=-1; char s2[MaxSize]; int top2=-1; int i=0; while(exp[i]!=‘\0‘){ if(‘0‘<=exp[i] && exp[i]<=‘9‘){ s1[++top1]=exp[i]-‘0‘; ++i; }else if(exp[i] == ‘(‘){ s2[++top2]=‘(‘; ++i; }else if(exp[i] == ‘+‘||exp[i] ==‘-‘||exp[i]==‘*‘||exp[i]==‘/‘){ if(top2 == -1 ||s2[top2] ==‘(‘||getPriority(exp[i])>getPriority(s2[top2])){ s2[++top2]=exp[i]; ++i; }else{ int flag=calStackTopTwo(s1,top1,s2,top2); if(flag == 0){ return 0; } } }else if(exp[i] ==‘)‘){ while(s2[top2] !=‘(‘){ int flag=calStackTopTwo(s1,top1,s2,top2); if(flag == 0){ return 0; } } --top2;//左括号丢掉 ++i; } } while(top2!=-1){ int flag=calStackTopTwo(s1,top1,s2,top2); if(flag == 0){ return 0; } } return s1[top1]; }
2.用Stack求后缀表达式的值
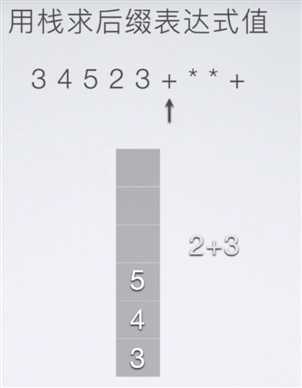
int calSub(float opand1,char op,float opand2,float &result){ if(op == ‘+‘) result =opand1+opand2; if(op == ‘-‘) result =opand1-opand2; if(op == ‘*‘) result=opand1*opand2; if(op == ‘/‘){ if(fabs(opand2)<MIN){ return 0; }else{ result=opand1/opand2; } } return 1; } float calPostFix(char exp[]){ float s[MaxSize];int top=-1;for(int i=0;exp[i]!=‘\0‘;++i){ if(‘0‘<=exp[i] &&exp[i]<=9){ s[++top]=exp[i]-‘0‘; }else{ float opnd1,opnd2,result; char op; int flag; opnd2=s[top--]; opnd1=s[top--]; op=exp[i]; flag=calSub(opnd1,op,opnd2,result); if(flag == 0){ cout<<"ERROR"<<endl; } s[++top]=result; } } return s[top]; }
3.用Stack求前缀表达式的值
不同点:从右往左扫描,先出栈的排左边,后出栈的排右边。
float calPreFix(char exp[],int len){ float s[MaxSize];int top=-1; int i=len-1; for(i;i>=0;i--){ if(‘0‘<=exp[i] &&exp[i]<=9){ s[++top]=exp[i]-‘0‘; }else{ float opnd1,opnd2,result; char op; int flag; opnd2=s[top--]; opnd1=s[top--]; op=exp[i]; flag=calSub(opnd2,op,opnd1,result); if(flag == 0){ cout<<"ERROR"<<endl; } s[++top]=result; } } return s[top]; }
标签:inf span 后缀 ima max lazy tac 中缀表达式 不同
原文地址:https://www.cnblogs.com/jcahsy/p/13187655.html