标签：lazy   tps   子节点   查询   c++   ini   直接   can   splay   

前置芝士

熟练掌握二叉排序树的操作，了解 \(Treap\) 的左旋和右旋。

引言

\(Treap\) 巧妙地使用随机数，解决了二叉查找树保持平衡的问题。但随机数的不稳定，导致它在极小概率的情况下不能保持树的平衡。故我们需要一种更加稳定的数据结构（虽然它不是很好写）。

正文

\(Splay\) ，又名分裂树，是一种二叉排序树，它能在 \(O(log_n)\) 内完成插入、查找和删除操作。它由丹尼尔·斯立特 \(Daniel Sleator\) 和 罗伯特·恩卓·塔扬 \(Robert Endre Tarjan\) 在1985年发明的。¹

\(Splay\) 的变量

\(Splay\) 的每个节点 \(x\) 有如下七种变量：
\(val_x\) : 该节点的值。
\(siz_x\) : 该节点的子树包含的节点数量；
\(sons_{x, 0}、sons_{x, 1}\): 该节点的左右子节点；
\(fa_x\) : 该节点的父亲节点；
\(num_x\) : \(val_x\) 的数量；

具体操作

初始化节点

即将该节点上述5个变量的值清空。

void init (int x) {
	fa[x] = siz[x] = num[x] = val[x] = sons[x][0] = sons[x][1] = 0;
	return ;
}

更新节点

更新该节点的子树包含的节点数（ \(siz\) 数组）。

void pushup (int x) {
	siz[x] = siz[sons[x][0]] + siz[sons[x][1]] + num[x];
	return ;
}

判断节点与父亲的关系

int get (int x) {
	return sons[fa[x]][1] == x;
}

连接节点

设要将 \(x\) 连接到 \(y\) 的 \(z\) 儿子上（0为左儿子，1为右儿子，下同）。

void link (int x, int y, int z) {
	if (x)
		fa[x] = y;
	if (y)
		sons[y][z] = x;
	return ;
}

旋转

此处的旋转和 \(Treap\) 中的旋转有略微的不同。\(Treap\) 中的旋转是将当前节点挪至其原位置的左儿子或右儿子中，而 \(Splay\) 的旋转是将当前节点上移，但旋转的过程大致相同。
设 \(f\) 为当前节点x的父亲，\(ff\) 为 \(f\) 的父亲，\(d1\) 为 \(f\) 与 \(x\) 的关系（左儿子为0，右儿子为1），\(d2\) 为 \(ff\) 与 \(f\) 的关系。先将 \(x\) 的 \(d1\) ^ 1儿子连接到 \(f\) 点的 \(d1\) 儿子，再将 \(ff\) 连接到 \(f\) 的 \(d1\) ^ 1 儿子上，最后将已经移至原 \(f\) 位置的 \(x\) 点连接到 \(ff\) 点的 \(d2\) 儿子上。

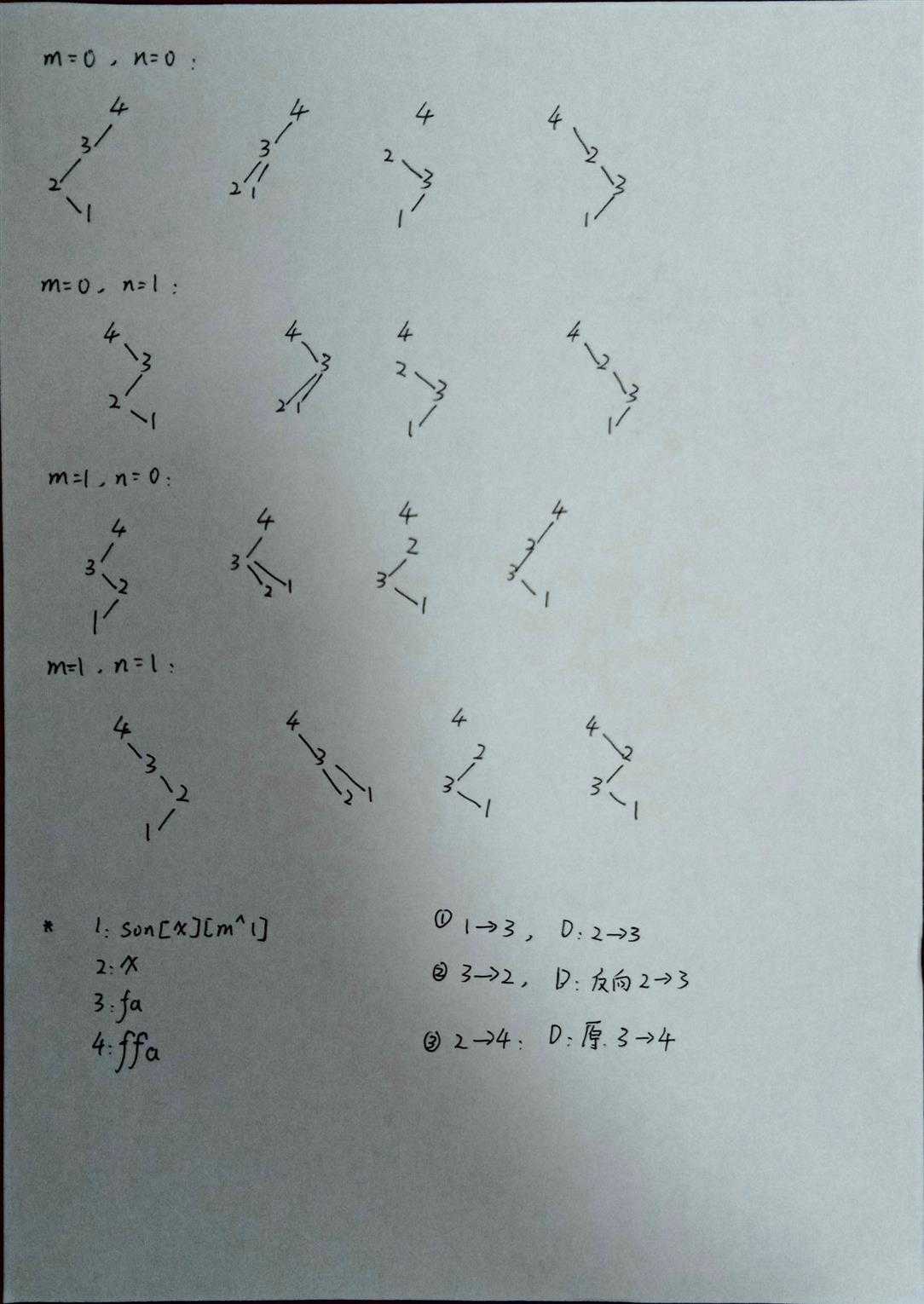
这是笔者在学习 \(Splay\) 时自己手绘的上旋步骤，可以方便读者更好地理解 \(Splay\) 的上旋操作。

void rotate (int x) {
	int f = fa[x], ff = fa[f], d1 = get (x), d2 = get (f);
	link (sons[x][d1 ^ 1], f, d1);
	link (f, x, d1 ^ 1);
	link (x, ff, d2);
	pushup (f);
	pushup (x);
	return ;
}

\(splay\)

\(splay\) 操作是 \(Splay\) 平衡树的核心，它保证了在经过插入删除等操作后，树的平衡性和随机性不受到破坏。（这里的 \(splay\) ，非指数据结构名，而是该数据结构的一种将某节点上旋到规定节点的一种操作。以下用" \(Splay\) "指数据结构名，" \(splay\) "指 \(Splay\) 的连续上旋操作）。
设目前需要上旋的节点为 \(x\) ，\(x\) 的父亲节点为 \(f\) 。现要将该点上旋，分三种情况讨论：

1、\(f\) 为目标点。此时可以直接上旋；

2、\(f\) 与 \(f\) 的父亲节点的关系和 \(x\) 与 \(f\) 的关系相同（即 \(get(x) = get (f)\) ）。此时，先上旋 \(f\) ，再上旋 \(x\)；

3、\(f\) 与 \(f\) 的父亲节点的关系和 \(x\) 与 \(f\) 的关系不同（即 \(get(x) ≠ get (f)\) ）。此时，连续上旋两次 \(x\) ；

若模拟上述操作可发现，在使用这种方式进行上旋的时候，树的平衡性并没有遭到破坏，因此 \(Splay\) 可以随意对树的任意节点使用。无特殊情况下，为了方便操作，一般直接旋到根节点。

void splay (int x, int top = 0) {
	for (int now; (now = fa[x]) && now != top; rotate (x))
		if (fa[now])
			rotate (get (now) == get (x) ? now : x);
	if (!top)
		rt = x;
	return ;
}

求根节点前缀

因为二叉排序树的性质，直接求左子树的最大值即可。

int pre () {
	int now = sons[rt][0];
	while (sons[now][1])
		now = sons[now][1];
	return now;
}

求根节点后继

因为二叉排序树的性质，直接求右子树的最小值即可。

int suc () {
	int now = sons[rt][1];
	while (sons[now][0])
		now = sons[now][0];
	return now;
}

求某数（\(x\)）的排名

从根节点向下遍历，若 \(x\) 大于当前节点的值，则在最终返回答案中加上 左子树的节点数 和 当前节点的同值的数，并查询右儿子；若 \(x\) 小于当前节点的值，则直接查询左儿子。

int find (int x) {
	int now = rt, ret = 0;
	while (1)
		if (x == val[now]) {
			ret += siz[sons[now][0]] + 1;
			splay (now);
			return ret;
		} else if (x < val[now]) {
			now = sons[now][0];
			continue ;
		} else {
			ret += siz[sons[now][0]] + num[now];
			now = sons[now][1];
		}
	return ret;
}

查询某排名的数

和 \(Treap\) 的操作相同，可以直接查看我在 \(Treap\) 中的教程。此处不再赘述。

int ranks (int x) {
	int now = rt;
	while (1)
		if (x <= siz[sons[now][0]])
			now = sons[now][0];
		else if (x > siz[sons[now][0]] + num[now]) {
			x -= (siz[sons[now][0]] + num[now]);
			now = sons[now][1];
		} else {
			splay (now);
			return val[now];
		}
}

插入操作

和 \(Treap\) 中的插入操作几乎相同。但是和 \(Treap\) 不同的是，不需要使用随机数进行保持平衡，而是在每次插入完成后直接将插入的点 \(Splay\) 到根节点，以此用一种很暴力的方式来保持树的平衡。

void ins (int x) {
	if (!rt) {
		rt = ++ cnt;
		num[rt] = siz[rt] = 1;
		val[rt] = x;
		return ;
	}
	int now = rt, f = 0;
	while (1) {
		if (val[now] == x) {
			num[now] ++;
			pushup (now);
			pushup (f);
			splay (now);
			return ;
		}
		f = now;
		now = sons[now][val[now] < x];
		if (!now) {
			now = ++ cnt;
			num[now] = siz[now] = 1;
			val[now] = x;
			fa[now] = f;
			sons[f][val[f] < x] = now;
			pushup (f);
			splay (now);
			return ;
		}
	}
	return ;
}

删除操作

首先运行一遍 \(find\) 函数，不过不是为了寻找排名，而是将需删除的节点移至根节点，方便在删除结点之后的连边操作。其他操作与 \(Treap\) 相同。

void del (int x) {
	find (x);
	if (num[rt] > 1) {
		num[rt] --;
		pushup (rt);
		return ;
	}
	if (!sons[rt][0] && !sons[rt][1]) {
		init (rt);
		rt = 0;
		return ;
	}
	if (!sons[rt][0] && sons[rt][1]) {
		int t = rt;
		rt = sons[rt][1];
		fa[rt] = 0;
		init (t);
		return ;
	}
	if (sons[rt][0] && !sons[rt][1]) {
		int t = rt;
		rt = sons[rt][0];
		fa[rt] = 0;
		init (t);
		return ;
	}
	if (sons[rt][0] && sons[rt][1]) {
		int p1 = rt, p2 = pre ();
		splay (p2);
		link (sons[p1][1], rt, 1);
		init (p1);
		pushup (rt);
		return ;
	}
}

整体代码

#include <bits/stdc++.h>
using namespace std;
const int N = 1e5 + 5;
int n, m, cnt, rt, sons[N][2], fa[N], val[N], siz[N], num[N];
void init (int x) {
	fa[x] = siz[x] = num[x] = val[x] = sons[x][0] = sons[x][1] = 0;
	return ;
}
int get (int x) {
	return sons[fa[x]][1] == x;
}
void link (int x, int y, int z) {
	if (x)
		fa[x] = y;
	if (y)
		sons[y][z] = x;
	return ;
}
void pushup (int x) {
	siz[x] = siz[sons[x][0]] + siz[sons[x][1]] + num[x];
	return ;
}
void rotate (int x) {
	int f = fa[x], ff = fa[f], d1 = get (x), d2 = get (f);
	link (sons[x][d1 ^ 1], f, d1);
	link (f, x, d1 ^ 1);
	link (x, ff, d2);
	pushup (f);
	pushup (x);
	return ;
}
void splay (int x, int top = 0) {
	for (int now; (now = fa[x]) && now != top; rotate (x))
		if (fa[now])
			rotate (get (now) == get (x) ? now : x);
	if (!top)
		rt = x;
	return ;
}
void ins (int x) {
	if (!rt) {
		rt = ++ cnt;
		num[rt] = siz[rt] = 1;
		val[rt] = x;
		return ;
	}
	int now = rt, f = 0;
	while (1) {
		if (val[now] == x) {
			num[now] ++;
			pushup (now);
			pushup (f);
			splay (now);
			return ;
		}
		f = now;
		now = sons[now][val[now] < x];
		if (!now) {
			now = ++ cnt;
			num[now] = siz[now] = 1;
			val[now] = x;
			fa[now] = f;
			sons[f][val[f] < x] = now;
			pushup (f);
			splay (now);
			return ;
		}
	}
	return ;
}
int find (int x) {
	int now = rt, ret = 0;
	while (1)
		if (x == val[now]) {
			ret += siz[sons[now][0]] + 1;
			splay (now);
			return ret;
		} else if (x < val[now]) {
			now = sons[now][0];
			continue ;
		} else {
			ret += siz[sons[now][0]] + num[now];
			now = sons[now][1];
		}
	return ret;
}
int ranks (int x) {
	int now = rt;
	while (1)
		if (x <= siz[sons[now][0]])
			now = sons[now][0];
		else if (x > siz[sons[now][0]] + num[now]) {
			x -= (siz[sons[now][0]] + num[now]);
			now = sons[now][1];
		} else {
			splay (now);
			return val[now];
		}
}
int pre () {
	int now = sons[rt][0];
	while (sons[now][1])
		now = sons[now][1];
	return now;
}
int suc () {
	int now = sons[rt][1];
	while (sons[now][0])
		now = sons[now][0];
	return now;
}
void del (int x) {
	find (x);
	if (num[rt] > 1) {
		num[rt] --;
		pushup (rt);
		return ;
	}
	if (!sons[rt][0] && !sons[rt][1]) {
		init (rt);
		rt = 0;
		return ;
	}
	if (!sons[rt][0] && sons[rt][1]) {
		int t = rt;
		rt = sons[rt][1];
		fa[rt] = 0;
		init (t);
		return ;
	}
	if (sons[rt][0] && !sons[rt][1]) {
		int t = rt;
		rt = sons[rt][0];
		fa[rt] = 0;
		init (t);
		return ;
	}
	if (sons[rt][0] && sons[rt][1]) {
		int p1 = rt, p2 = pre ();
		splay (p2);
		link (sons[p1][1], rt, 1);
		init (p1);
		pushup (rt);
		return ;
	}
}
int main () {
	scanf ("%d", &n);
	for (int i = 1; i <= n; i ++) {
		int opt, x;
		scanf ("%d%d", &opt, &x);
		if (opt == 1)
			ins (x);
		else if (opt == 2)
			del (x);
		else if (opt == 3)
			printf ("%d\n", find (x));
		else if (opt == 4)
			printf ("%d\n", ranks (x));
		else if (opt == 5) {
			ins (x);
			printf ("%d\n", val[pre ()]);
			del (x);
		} else if (opt == 6) {
			ins (x);
			printf ("%d\n", val[suc ()]);
			del (x);
		}
	}
	return 0;
}

References

[1] Sleator, Daniel D.; Tarjan, Robert E., Self-Adjusting Binary Search Trees (PDF), Journal of the ACM, 1985, 32 (3): 652–686

Splay

标签：lazy   tps   子节点   查询   c++   ini   直接   can   splay   

原文地址：https://www.cnblogs.com/HarryHuang2004/p/13219936.html