标签:des c style class blog code
图的【十字链表】表示法是一种链式存储结构,可以看成是【邻接表】和【逆邻接表】的组合
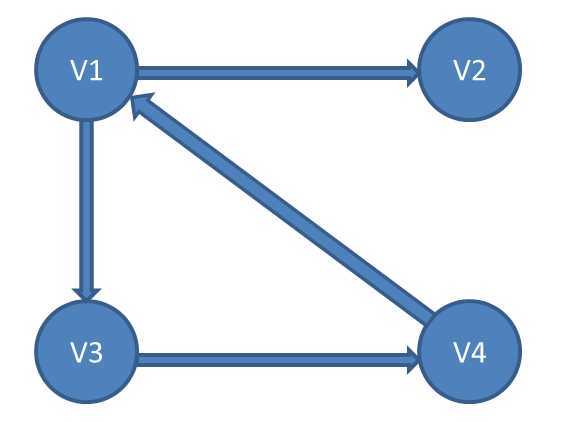
本文中用到的有向图
/************************************************************************
有向图的存储:十字链表
有向图的十字链表存储结构,是有一种链式存储结构,可以看成是【邻接表】和【逆邻接表】
的结合。
图中每条弧对应一个【弧结点】,每个顶点对应一个【顶点结点】
弧结点
--------------------------------------------
| tailvex | headvex | hlink | tlink | info |
--------------------------------------------
talivex:以该弧为【弧尾】的结点在图中的位置
headvex:以该弧为【弧头】的结点在图中的位置
hlink: 下一条与该弧有【相同弧头的弧】
tlink: 下一条与该弧有【相同弧尾的弧】
info: 弧的相关信息,权值等
顶点结点
-----------------------------
| data | firstin | firstout |
-----------------------------
data: 该结点的数据
firstin: 第一条以该弧为弧头的【弧结点】
firstout:第一条以该弧为弧尾的【弧结点】
************************************************************************/
相关数据结构
//顶点结点最大数量
int const MAX_VERTEXNUM = 100;
//数据结构
typedef int InfoType;
typedef int Data;
//弧结点
typedef struct _tagArcBox
{
int tailvex; //该弧的弧尾结点在图中的位置
int headvex; //该弧的弧头结点在图中的位置
struct _tagArcBox* hlink; //下一条与该弧有相同弧头结点的弧
struct _tagArcBox* tlink; //下一条与该弧有相同弧尾结点的弧
InfoType info; //弧的相关信息
}ArcBox;
//顶点结点
typedef struct _tagArcNode
{
Data data; //数据
ArcBox* firstin; //第一条以该节点为弧尾的弧
ArcBox* firstout; //第一条以该结点为弧头的弧
}ArcNode;
//十字链表存储结构的有向图
typedef struct _tagOLGraph
{
ArcNode vertex[MAX_VERTEXNUM]; //顶点向量
int vexnum; //顶点数
int arcnum; //弧树
}OLGraph, *POLGraph;
从顶点向量中查找该顶点在图中的位置(下标)
//输入图的【顶点向量】和某个顶点的数据
//获取此顶点在顶点向量中的位置(下标)
int LocateNode(ArcNode* pNodesArr, Data data, int length)
{
if( NULL == pNodesArr ) return NULL;
for (int i = 0; i < length; ++ i)
{
if (pNodesArr[i].data == data) return i;
}
return -1;
}
//输入【图】和某顶点的数据
//获取该顶点在顶点向量中的位置(下标)
int LocateNode(POLGraph& pOLGraph, Data data)
{
return (NULL == pOLGraph ?
NULL : LocateNode(pOLGraph->vertex, data, pOLGraph->vexnum) );
}
有向图的创建
/************************************************************************
当使用这种函数原型时:
void CreateOLGraph(POLGPraph& pOLGraph)
{
pOLGraph = new OLGraph();
//codes
pOLGraph->vexnum = num; //这里报运行时错误! why?
}
************************************************************************/
//创建十字链表有向图
POLGraph CreateOLGraph()
{
POLGraph pOlGraph = NULL;
__try
{
pOlGraph = new OLGraph();
if(NULL == pOlGraph) {cerr << "申请图结点失败!\n"; return NULL;}
int num = 0;
cout << "请输入图的顶点数量:";
cin >> num;
pOlGraph->vexnum = num;
cout << endl;
cout << "请输入图的弧的数量:";
cin >> num;
pOlGraph->arcnum = num;
cout << endl;
Data data = 0;
cout << endl << "--------开始 初始化顶点向量-----------"<<endl;
for (int i = 0; i < pOlGraph->vexnum; ++i)
{
cout << "请输入结点的值:";
cin >> data;
pOlGraph->vertex[i].data = data;
pOlGraph->vertex[i].firstin = NULL;
pOlGraph->vertex[i].firstout = NULL;
cout<<endl;
} //for
cout <<endl<<"------------结束 初始化顶点向量------------"<<endl;
cout<<endl<<endl;
cout << "************开始 初始化弧结点 **************"<<endl;
for(int i = 0; i < pOlGraph->arcnum; ++ i)
{
cout << "请输入弧的弧尾结点:";
cin >> data;
int begin = LocateNode(pOlGraph->vertex, data, pOlGraph->arcnum);
if(-1 == begin) {cerr << "您输入的弧尾结点不在图中,请重新输入"<<endl; --i; continue;}
cout << "请输入弧的弧头结点:";
cin >> data;
int end = LocateNode(pOlGraph->vertex, data, pOlGraph->arcnum);
if(-1 == end) {cerr << "您输入的弧头结点不在图中,请重新输入"<<endl; -- i; continue;}
cout << "请输入弧的权值:";
cin >> data;
cout<<endl<<endl;
ArcBox* pArcBox = new ArcBox();
if(NULL == pArcBox) {cerr << "申请弧结点失败!"<<endl; -- i; continue;}
pArcBox->tailvex = begin; //该弧的弧尾在图中的位置
pArcBox->headvex = end; //该弧的弧头在图中的位置
pArcBox->hlink = pOlGraph->vertex[end].firstin; //下一条与该弧有相同弧尾的弧结点
pArcBox->tlink = pOlGraph->vertex[begin].firstout; //下一条与该弧有相同弧头的弧结点
pArcBox->info = data; //权值
pOlGraph->vertex[begin].firstout = pOlGraph->vertex[end].firstin = pArcBox;
} //for
} //__try
__except(1)
{
cerr << endl<<"有异常发生"<<endl;
}
return pOlGraph;
}
运行情况:

出度和入度
//求图的出度
//先根据输入的顶点的值,求得该点所在的顶点向量的分量
//然后得到该点的firstout,然后再得到所有与该弧有相同弧尾
//结点的弧(的条数)
int OutDegree(POLGraph& pOLGraph, Data data)
{
int nCount = 0;
//根据结点的值定位该点在图的顶点向量中的位置(下标)
int nIndex = LocateNode(pOLGraph, data);
if(-1 == nIndex) {cerr << "该点不在图中,所以没有出度!\n"<<endl; return -1;}
//得到该结点指针
ArcNode* pArcNode = &(pOLGraph->vertex[nIndex]);
if(NULL == pArcNode) {cerr << "在图中查找该顶点出错!\n"<<endl; return -1;}
//第一条该顶点为弧尾的弧(该顶点结点的第一条出边)
ArcBox* pArcBox = pArcNode->firstout;
//查找所有以该【顶点结点】为【弧尾】的【弧结点】
while(NULL != pArcBox)
{
++ nCount;
pArcBox = pArcBox->tlink;
}
return nCount;
}
//定点的入度
int InDegree(POLGraph& pOLGraph, Data data)
{
int nCount = 0;
//定位该顶点结点在顶点向量中的位置(下标)
int nIndex = LocateNode(pOLGraph, data);
if(-1 == nIndex){cerr << "该点不在图中,所以没有入度!\n"<<endl; return -1;}
//得到该顶点结点的指针
ArcNode* pArcNode = &(pOLGraph->vertex[nIndex]);
if(NULL == pArcNode) {cerr << "在图中查找该点出错!"<<endl; return -1;}
//第一条以该顶点结点为弧头的弧(该顶点结点的第一条入边)
ArcBox* pArcBox = pArcNode->firstin;
//查找所有以该【顶点结点】为【弧头】的【弧结点】
while(NULL != pArcBox)
{
++nCount;
pArcBox = pArcBox->hlink;
}
return nCount;
}
查找出度 / 入度
POLGraph pGraph = CreateOLGraph();
int num = 0;
for(int i = 0; i < pGraph->vexnum + 3; ++ i)
{
cout << "请输入带查的顶点的值:";
cin >> num;
cout<<"结点 "<< num << " 的出度OutDegree为:"<<OutDegree(pGraph, num);
cout<<endl;
cout<<"结点 "<< num << " 的入度InDegree为:"<<InDegree(pGraph, num);
cout<<endl<<endl;
}

深度优先遍历(DFS)
/************************************************************************
深度优先遍历
从某顶点出发,访问之,然后获得该顶点的第一条出边(firstout),如果该出边
不为空,则获得该条出边的【弧头】结点在图中的位置(下标),查看此下标的结点
是否被访问过,如果没有则根据此下标获取该结点,然后递归访问之;如果此结点
被访问过了,则说明出现回路,及此条弧指向了之前访问过的结点,需要跳出循环,
否则出现死循环。
************************************************************************/
//顶点结点是否遍历过标志数组
bool* pVisited = NULL;
void DFS(POLGraph& pOLGraph, ArcNode* pArcNode)
{
int nIndex = LocateNode(pOLGraph, pArcNode->data);
pVisited[nIndex] = true;
cout << "the node is "<<pArcNode->data<<endl;
ArcBox* pArcBox = pArcNode->firstout;
while(NULL != pArcBox)
{
//该弧的弧头在图中的位置
int nHeadVex = pArcBox->headvex;
if (pVisited[nHeadVex] == false)
{
ArcNode* pHeadNode = &(pOLGraph->vertex[nHeadVex]);
DFS(pOLGraph, pHeadNode);
}
//如果某条弧的弧头结点已经被访问过时,则说明已经有了回路,此时要跳出循环
//否则会在while中死循环
else
{
break;
}
}
}
//有向图的深度优先遍历
void DFSTraverse(POLGraph& pOLGraph)
{
if(NULL == pOLGraph) {cerr << "该图为空!"; return;}
pVisited = new bool[pOLGraph->vexnum]();
for (int i = 0; i < pOLGraph->vexnum; ++ i) pVisited[i] = false;
for (int i = 0; i < pOLGraph->vexnum; ++ i)
{
if (! pVisited[i])
{
DFS(pOLGraph, &pOLGraph->vertex[i]);
}
}
}
深度优先遍历结果

广度优先遍历(BFS)
【算法与数据结构】图 -- 十字链表,布布扣,bubuko.com
标签:des c style class blog code
原文地址:http://www.cnblogs.com/cuish/p/3764550.html