跳跃表 -- 随机平衡原理

标签：

Skip lists are a data structure that can be used in place of balanced trees. Skip lists use probabilistic balancing rather than strictly enforced balancing and as a result the algorithms for insertion and deletion in skip lists are much simpler and significantly faster than equivalent algorithms for balanced trees.

Enhanced LinkedList

要理解SkipList，得先从LinkedList说起，

LinkedList 增删改查的时间复杂度都是O(N)，它最大的问题就是通过一个节点只能reach到下一个节点（Doubly LinkedList 是一种改进方案），那么改进的思路就是通过一个节点reach到多个节点，例如下图，

这种情况下便可将复杂度减小为O(N/2)。这是一种典型的空间换时间的优化思路。

SkipList 更进一步，采用了分治算法和随机算法设计。将每个节点所能reach到的最远的节点，两个节点之间看成是一个组，整个SkipList被分成了许多个组，而这些组的形成是随机的。

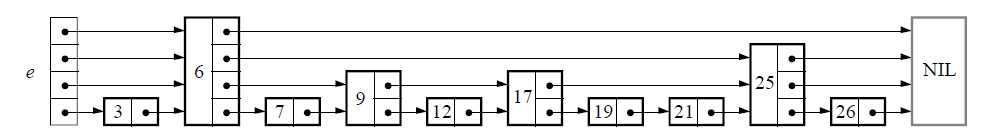
（顺时针旋转90度看，SkipList其实更像是一个图结构^_^）

上图中，[3, 6]，[6, 25]，[25, 26]是三个大组，而在[6, 25]这个组里面又包含了[6, 9]，[9, 17]，[17, 25]这三个组，其中还继续细分了下去。当你在SkipList中查找某个节点时，很容易就可以跳过某个分组，这样便大大提升了查找效率。这样的分组方式可以实现二分查找。

每个节点所能reach到的最远的节点是随机的，正如作者所说，SkipList使用的是概率平衡而不是强制平衡。

O(logN)?

既然是随机算法，那怎么能保证O(logN)的复杂度？SkipList作者在论文中有给出了说明，这里从另一个角度说下我的理解。先定义一下，A node that has k forward pointers is called a level k node。假设k层节点的数量是k+1层节点的P倍，那么其实这个SkipList可以看成是一棵平衡的P叉树，从最顶层开始查找某个节点需要的时间是O(logpN)，which is O(logN) when p is a constant。

下面看下Redis与LevelDB中实现SkipList所使用的随机算法。

Redis

在t_zset.c中找到了redis使用的随机算法。

/* Returns a random level for the new skiplist node we are going to create.
 * The return value of this function is between 1 and ZSKIPLIST_MAXLEVEL
 * (both inclusive), with a powerlaw-alike distribution where higher
 * levels are less likely to be returned. */
int zslRandomLevel(void) {
    int level = 1;
    while ((random()&0xFFFF) < (ZSKIPLIST_P * 0xFFFF))
        level += 1;
    return (level<ZSKIPLIST_MAXLEVEL) ? level : ZSKIPLIST_MAXLEVEL;
}

执行level += 1;的概率为ZSKIPLIST_P，也就是说k层节点的数量是k+1层节点的1/ZSKIPLIST_P倍。ZSKIPLIST_P（这个P是作者论文中的p）与ZSKIPLIST_MAXLEVEL在redis.h中定义，

#define ZSKIPLIST_MAXLEVEL 32 /* Should be enough for 2^32 elements */
#define ZSKIPLIST_P 0.25      /* Skiplist P = 1/4 */

所以redis中的SkipList相当于是一棵四叉树。

LevelDB

在skiplist.h中找到了LevelDB使用的随机算法。

template<typename Key, class Comparator>
int SkipList<Key,Comparator>::RandomHeight() {
  // Increase height with probability 1 in kBranching
  static const unsigned int kBranching = 4;
  int height = 1;
  while (height < kMaxHeight && ((rnd_.Next() % kBranching) == 0)) {
    height++;
  }
  assert(height > 0);
  assert(height <= kMaxHeight);
  return height;
}

(rnd_.Next() % kBranching) == 0)的概率为1/kBranching，所以LevelDB中的SkipList也是一棵四叉树（kBranching = 4;不就是这个意思吗^_^）。

参考资料

跳跃表 -- 随机平衡原理

标签：

原文地址：http://www.cnblogs.com/thrillerz/p/4556338.html