Now you get a number N, and a M-integers set, you should find out how many integers which are small than N, that they can divided exactly by any integers in the set. For example, N=12, and M-integer set is {2,3}, so there is another set {2,3,4,6,8,9,10}, all the integers of the set can be divided exactly by 2 or 3. As a result, you just output the number 7.

  There are a lot of cases. For each case, the first line contains two integers N and M. The follow line contains the M integers, and all of them are different from each other. 0<N<2^31,0<M<=10, and the M integer are non-negative and won’t exceed 20.

  For each case, output the number.

12 2
2 3

7
今天扣了一下容斥定理，离散结构中有，却是不记得了，总结一下，实现容斥原理大致有三种方法：dfs，队列数组，二进制，可以按照实际情况以及个人爱好自己选择
容斥定理内容：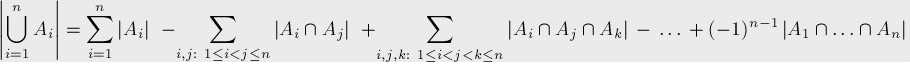

大家可以理解为奇数个的并集则是加，偶数个的并集则是减，具体的建议大家翻阅资料了解原理
这道题目很明显符合容斥定理，所以我就用二进制过了

#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
typedef long long LL;
const int MAXN = 50 + 5;
int n, m;
LL A[MAXN];
LL gcd(LL a,LL b) {
    return b ? gcd(b, a % b) : a;
}
LL lcm(LL a,LL b) {
    return a / gcd(a, b) * b;
}
int main() {
    while(~ scanf("%d%d", &n, &m)) {
        for(int i = 0; i < m; i ++) {
            scanf("%I64d", &A[i]);
        }
        int ms = 0;
        for(int i = 0 ;i < m;i ++){
            if(A[i] == 0) continue;
            A[ms ++] = A[i];
        }
        m = ms;
        LL ans = 0;
        for(int i = 1; i < (1 << m); i ++) {
            int bits = 0;
            LL res = 1;
            for(int j = 0; j < m; j ++) {
                if(i & (1 << j)) {
                    bits ++;
                    res = lcm(res, A[j]);
                }
            }
            if(bits & 1) ans += (LL)(n - 1) / res;
            else ans -= (LL)(n - 1) / res;
        }
        printf("%I64d\n", ans);
    }
    return 0;
}