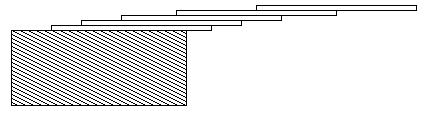
How far can you make a stack of cards overhang a table? If you have one card, you can create a maximum overhang of half a card length. (We‘re assuming that the cards must be perpendicular to the table.) With two cards you can make the top card overhang the bottom one by half a card length, and the bottom one overhang the table by a third of a card length, for a total maximum overhang of 1/2 + 1/3 = 5/6 card lengths. In general you can make n cards overhang by 1/2 + 1/3 + 1/4 + ... + 1/(n + 1) card lengths, where the top card overhangs the second by 1/2, the second overhangs tha third by 1/3, the third overhangs the fourth by 1/4, etc., and the bottom card overhangs the table by 1/(n + 1). This is illustrated in the figure below.
附上AC代码:
#include <iostream>
#include <cstdio>
#include <string>
#include <cmath>
#include <iomanip>
#include <ctime>
#include <climits>
#include <cstdlib>
#include <cstring>
#include <algorithm>
typedef unsigned int UI;
typedef long long LL;
typedef unsigned long long ULL;
typedef long double LD;
const double PI = 3.14159265;
const double E = 2.71828182846;
int main()
{
using namespace std;
std::ios::sync_with_stdio(false);
double len;
while (cin >> len && len > 0)
{
double sum = 0;
int i;
for (i=2; sum<len; i++)
sum += 1.0/i;
cout << i-2 << " card(s)\n";
}
return 0;
}
版权声明:本文为博主原创文章,未经博主允许不得转载。
原文地址:http://blog.csdn.net/silenceneo/article/details/47776339